Find the sides of the square LMNO whose diagonal measures 6 cm.
To find the sides of the square LMNO whose diagonal measures 6 cm, we can follow these steps:
1. Understand the Properties of the Square:
- In a square, all sides are equal, and the diagonals bisect each other at right angles.
2. Identify the Diagonal:
- The diagonal of square LMNO is given as 6 cm.
3. Use the Relationship Between the Diagonal and the Side:
- The relationship between the side length (let's denote it as x) and the diagonal d of a square is given by the formula:
d=x√2
- Here, d=6 cm.
4. Set Up the Equation:
- Substitute the value of the diagonal into the formula:
6=x√2
5. Solve for x:
- To isolate x, divide both sides by √2:
x=6√2
- To rationalize the denominator, multiply the numerator and denominator by √2:
x=6√22=3√2
6. Conclusion:
- The length of each side of the square LMNO is 3√2 cm.
Final Answer:
The sides of the square LMNO are 3√2 cm.
---
- In a square, all sides are equal, and the diagonals bisect each other at right angles.
- The diagonal of square LMNO is given as 6 cm.
- The relationship between the side length (let's denote it as x) and the diagonal d of a square is given by the formula:
d=x√2
- Here, d=6 cm.
- Substitute the value of the diagonal into the formula:
6=x√2
- To isolate x, divide both sides by √2:
x=6√2
- To rationalize the denominator, multiply the numerator and denominator by √2:
x=6√22=3√2
- The length of each side of the square LMNO is 3√2 cm.
The sides of the square LMNO are 3√2 cm.
Topper's Solved these Questions
- PRACTICAL GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - PRACTICAL GEOMETRYEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL-2 COMPREHENSION TYPE)
4 Videos
- PLAYING WITH NUMBERSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - PLAYING WITH NUMBERSEXERCISE - Olympiad/HOTS Corner
6 Videos
- RATIONAL NUMBERS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - Olympiad/HOTS Corner
20 Videos
Similar Questions
Knowledge Check
Find the side of a square whose diagonal is 10 cm.
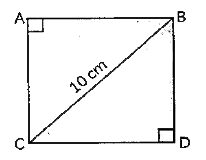 A5√2cmB10√2cmC5cmD10cm
A5√2cmB10√2cmC5cmD10cmLet A be the area of a square whose each side is 10 cm. Let B be the area of the square whose diagonals are 14 cm each. Then (A-B) is equal to
A0B1C2D4
What is the area of a square whose diagonal measures 4 cm?
View SolutionWhat is the area of a square whose diagonal measures 4 cm?
View SolutionFind the side of a square whose diagonal is 16√2cm.
View SolutionFind the area of square whose diagonal.is 5√2 cm
View SolutionFind the side and perimeter of a square whose diagonal is 10 cm.
View SolutionFind the side and perimeter of a square whose diagonal is 10cm.
View Solution
