Construct a quadrilateral ABCD in which AB=3.8cm, BC=5cm,CD=5cm, DA=5.5cm and AC=8cm.
To construct the quadrilateral ABCD with the given dimensions, follow these steps:
Step-by-Step Construction
1. Draw Line Segment AB:
- Use a ruler to draw a line segment AB that measures 3.8 cm. Label the endpoints as A and B.
Hint: Make sure to measure accurately using the ruler.
2. Draw an Arc for BC:
- From point B, use a compass to draw an arc with a radius of 5 cm. This arc represents all possible locations for point C.
Hint: Keep the compass point at B and swing the compass to create a circular arc.
3. Draw an Arc for DA:
- From point A, draw another arc with a radius of 5.5 cm. This arc represents all possible locations for point D.
Hint: Ensure the compass width is set to 5.5 cm before drawing the arc.
4. Mark the Intersection Point:
- The two arcs you drew from points A and B will intersect at two points. Label one of these intersection points as C and the other as D.
Hint: You may need to adjust the compass if the arcs do not intersect clearly.
5. Draw Line Segments BC and DA:
- Connect points B and C with a straight line. Then, connect points D and A with another straight line.
Hint: Use a ruler to ensure the lines are straight.
6. Draw Line Segment CD:
- Finally, connect points C and D with a straight line.
Hint: Double-check the lengths of each side to ensure they match the given dimensions.
7. Verify the Diagonal AC:
- Measure the distance between points A and C to confirm that it is 8 cm.
Hint: Use the ruler to measure the diagonal carefully.
Final Check
- Ensure that all sides are correctly measured:
- AB = 3.8 cm
- BC = 5 cm
- CD = 5 cm
- DA = 5.5 cm
- AC = 8 cm
- Use a ruler to draw a line segment AB that measures 3.8 cm. Label the endpoints as A and B.
- From point B, use a compass to draw an arc with a radius of 5 cm. This arc represents all possible locations for point C.
- From point A, draw another arc with a radius of 5.5 cm. This arc represents all possible locations for point D.
- The two arcs you drew from points A and B will intersect at two points. Label one of these intersection points as C and the other as D.
- Connect points B and C with a straight line. Then, connect points D and A with another straight line.
- Finally, connect points C and D with a straight line.
- Measure the distance between points A and C to confirm that it is 8 cm.
- Ensure that all sides are correctly measured:
- AB = 3.8 cm
- BC = 5 cm
- CD = 5 cm
- DA = 5.5 cm
- AC = 8 cm
Topper's Solved these Questions
- PRACTICAL GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - PRACTICAL GEOMETRYEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL-2 COMPREHENSION TYPE)
4 Videos
- PLAYING WITH NUMBERSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - PLAYING WITH NUMBERSEXERCISE - Olympiad/HOTS Corner
6 Videos
- RATIONAL NUMBERS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIONAL NUMBERS EXERCISE - Olympiad/HOTS Corner
20 Videos
Similar Questions
Construct as quadrilateral ABCD in which AB=4.8cm, BC=4.3cm, CD=3.6cm, AD=4.2cm and diagonal AC=6cm.
View SolutionFind the area of the quadrilateral ABCD in which AB=3cm,BC=4cm,CD=4cm,DA=5cm and AC=5cm.
View SolutionFind the area of a quadrilateral ABCD in which AB=3 cm, BC=4 cm, CD=4 cm, DA=5 cm and AC=5 cm.
View SolutionConstruct a quadrilateral ABCD given that AB=3.5 cm, BC=4cm, CD=4.5cm, DA=5cm and ∠B=60∘.
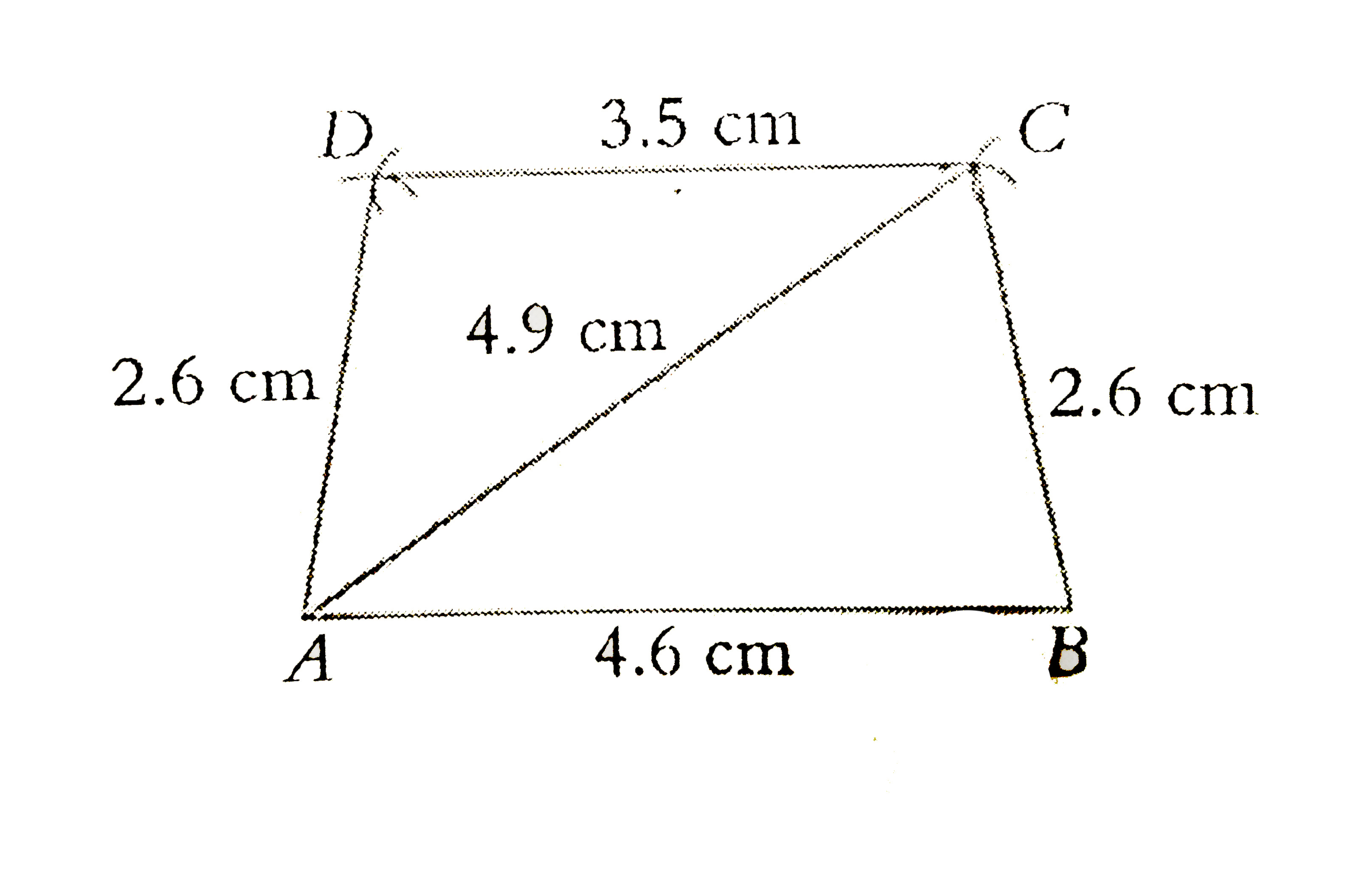
View SolutionConstruct a quadrilateral ABCD in which AB =4.6 cm , BC=2.6 cm, CD=3.5 cm, AD=2.6 cm , and the diagonal AC=4.9 cm.
View SolutionFind the area of a quadrilateral ABCD is which AB=3cm, BC=4cm, CD=4cm, DA=5cm and AC=5cm
S=12(a+b+c)=12(3+4+5)=6
arof ABC=sqrt(s(s-a)(s-b)(s-c)<br>ar ABC=√6(6−3)(6−4)(6−5)
⇒√6×3×2×1=6cm2
Now in △ADC,S=a+b+c2
⇒s=5+4+52=142=7cm
By using Heron’s formula
Area of △ADC=√s(s−a)(s−b)(s−c)
=√7(7−5)(7−4)(7−5)
=√7×2×3×2
=2√21cm2
Area of△ADC=9.2cm2 (approx.)
Area of the quadrilateral ABCD = Area of △ADC+Areaof△ABC=9.2cm2+6cm2=15.2cm2
Thus, the area of the quadrilateral,ABCDis15.2cm2.
View SolutionIs it possible to construct a quadrilateral ABCD in which AB = 3 cm, BC = 4 cm, CD = 5.4 cm, DA = 5.9 cm and diagonal AC = 8 cm? If not, why?
View SolutionConstruct a quadrilateral ABCD in which AB=4cm, BC=3.4cm, AD=2.8cm, diagonal AC=4.6cm and diagonal BD=4.2cm.
View SolutionConstruct a quadrilateral ABCD in which AB=4cm, BC=6cm, CD=6cm, ∠B=120∘ and ∠C=90∘.
View SolutionConstruct a quadrilateral ABCD given that AB=5cm, BC=4cm, CD=4.5cm, DA=3cm and diagonal AC=4cm.
View Solution
