Is it possible to construct a quadrilateral ABCD in which AB = 3 cm, BC = 4 cm, CD = 5.4 cm, DA = 5.9 cm and diagonal AC = 8 cm? If not, why?
To determine whether it is possible to construct a quadrilateral ABCD with the given dimensions, we will analyze the triangle formed by points A, B, and C, which is part of the quadrilateral.
1. Identify the Given Dimensions:
- AB = 3 cm
- BC = 4 cm
- CD = 5.4 cm
- DA = 5.9 cm
- AC = 8 cm
2. Check Triangle Inequality for Triangle ABC:
- In triangle ABC, the sides are AB, BC, and AC.
- We need to check if the sum of the lengths of any two sides is greater than the length of the third side.
3. Calculate the Sum of AB and BC:
- AB + BC = 3 cm + 4 cm = 7 cm
4. Compare the Sum with AC:
- We have AC = 8 cm.
- Now, we compare: AB + BC (7 cm) < AC (8 cm).
5. Apply the Triangle Inequality Rule:
- According to the triangle inequality theorem, the sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
- Here, since 7 cm (AB + BC) is less than 8 cm (AC), it violates the triangle inequality.
6. Conclusion:
- Since the triangle inequality is not satisfied, it is not possible to construct triangle ABC with the given dimensions.
- Therefore, it is also not possible to construct quadrilateral ABCD with the provided side lengths.
Final Answer:
No, it is not possible to construct quadrilateral ABCD with the given dimensions because the triangle inequality is violated in triangle ABC.
---
- AB = 3 cm
- BC = 4 cm
- CD = 5.4 cm
- DA = 5.9 cm
- AC = 8 cm
- In triangle ABC, the sides are AB, BC, and AC.
- We need to check if the sum of the lengths of any two sides is greater than the length of the third side.
- AB + BC = 3 cm + 4 cm = 7 cm
- We have AC = 8 cm.
- Now, we compare: AB + BC (7 cm) < AC (8 cm).
- According to the triangle inequality theorem, the sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
- Here, since 7 cm (AB + BC) is less than 8 cm (AC), it violates the triangle inequality.
- Since the triangle inequality is not satisfied, it is not possible to construct triangle ABC with the given dimensions.
- Therefore, it is also not possible to construct quadrilateral ABCD with the provided side lengths.
No, it is not possible to construct quadrilateral ABCD with the given dimensions because the triangle inequality is violated in triangle ABC.
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - APPLICATIONS, GAMES AND PUZZLES
3 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBOOK - NCERT EXEMPLARCHAPTER - SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTEXERCISE - Exercise
52 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
Similar Questions
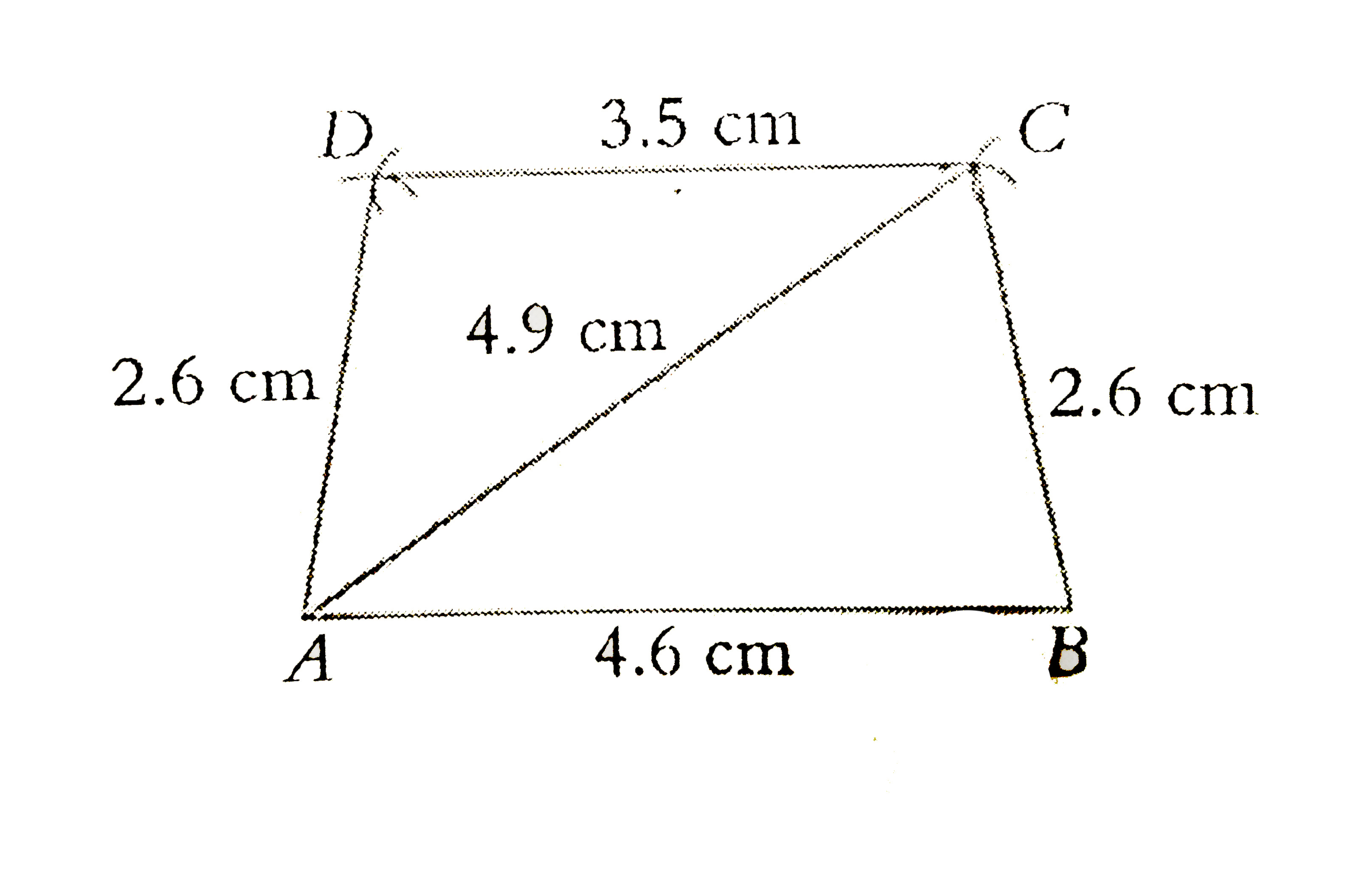
Construct a quadrilateral ABCD in which AB =4.6 cm , BC=2.6 cm, CD=3.5 cm, AD=2.6 cm , and the diagonal AC=4.9 cm.
View SolutionConstruct as quadrilateral ABCD in which AB=4.8cm, BC=4.3cm, CD=3.6cm, AD=4.2cm and diagonal AC=6cm.
View SolutionConstruct a quadrilateral ABCD in which AB=3.8cm, BC=5cm,CD=5cm, DA=5.5cm and AC=8cm.
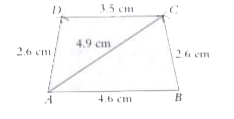
View SolutionConstruct a quadrilateral ABCD in which AB = 4 . 6 cm, BC = 2.6 cm, CD = 3.5 cm AD = 2 . 6 cm, and the diagonal AC = 4 . 9 cm
View SolutionConstruct a quadrilateral ABCD given that AB=5cm, BC=4cm, CD=4.5cm, DA=3cm and diagonal AC=4cm.
View SolutionConstruct a quadrilateral ABCD in which AB=4cm, BC=3.4cm, AD=2.8cm, diagonal AC=4.6cm and diagonal BD=4.2cm.
View SolutionConstruct a quadrilateral ABCD given that AB=3.5 cm, BC=4cm, CD=4.5cm, DA=5cm and ∠B=60∘.
View SolutionFind the area of a quadrilateral ABCD in which AB=3 cm, BC=4 cm, CD=4 cm, DA=5 cm and AC=5 cm.
View SolutionConstruct a quadrilateral ABCD with AB = 4 cm, BC = 2.8 cm, CD = 4 cm, ∠B=75∘and∠C=105∘
View SolutionConstruct a ΔABC in which AB=4cm,BC=5.2cm and CA=4.5cm.
View Solution
