Let OABCD be a pentagon in which the sides OA and CB are parallel and the sides OD and AB are parallel as shown in figure. Also, OA:CB=2:1 and OD:AB=1:3. if the diagonals OC and AD meet at x, find OX:XC.Solution in Punjabi
The correct Answer is:2:5
Let O be the origin of reference.
Let the position vectors of A,B,C and D be a,b,c and d,
respectively.
then, OA:CB=2:1
⇒OACB=21⇒OA+2CB

⇒OA=2CB
⇒a=2(b−c) . . . (i)
and OD:AB=1:3
⇒ODAB=13⇒3OD=AB
⇒3OD=AB
⇒3d=(b−a)=b−2(b−c) [using eq. (i)]
⇒3d=−b+2c . . . (ii)
Let OX:XC=λ:1andAX:XD=μ:1
Now, X divides OC in the ratio λ:1. therefore,
PV of X=λcλ+1 . . . (iii)
X also divides AD in the ratio μ:1
PV of X=μd+aμ+1
from eqs. (iii) and (iv), we get
λcλ+1=μd+aμ+1
⇒(λλ+1)c=(μμ+1)d+(1μ+1)a
⇒(λλ+1)c=(μμ+1)(−b+2c3)+(1μ+1)2(b−c)
[using eqs. (i) and (iv)]
⇒(λλ+1)c=(2μ+1−μ3(μ+1))b+(2μ3(μ+1)−2μ+1)c
⇒(λλ+1)c=(6−μ3(μ+1))b+(2μ−63(μ+1))c
⇒(6−μ3(μ+1))b+(2μ−63(μ+1)−λλ+1)c=0
⇒6−μ3(μ+1)=0and2μ−63(μ+1)−λλ+1=0
(since, b and c are non-collinear)
⇒μ=6andλ=25
Hence, OX:XC=2:5
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
9 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Single Integer Answer Type Questions)
7 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
Similar Questions
If vectors −−→AB=−3ˆi+4ˆkand−−→AC=5ˆi−2ˆj+4ˆk are the sides of a ΔABC, then the length of the median throught A is
View SolutionIf the resultant of three forces F1=pˆi+3ˆj−ˆk,F2=6ˆi−ˆkandF3=−5ˆi+ˆj+2ˆk acting on a particle has a magnitude equal to 5 units, then the value of p is
View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
View SolutionIn figure (OA)/(OC)=(OB)/(OD)=(1)/(2) and AB=5cm. Find the value of DC
View SolutionOABCD is a pyramid with square base ABCD such that bar(OA),bar(OB),bar(OC),bar(OD) are unit vectors and bar(OA) , bar(OB) = bar(OB) .bar(OC) = bar(OC) . bar(OD) = bar(OD) . bar(OA) =1/2, then volume of the pyramid is
View Solutionएक चक्रीय चतुर्भुज ABCD कि दो भुजाएँ AB और CD समांतर है। सिध्द कीजिए कि भुजाएँ AD और BC आपस में बराबर है और विकर्ण AC और BD भी बराबर है।
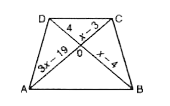
View Solutionदी गई आकृति में AB, CD के समान्तर है । यदि OA=3x−19,OB=x−4,
OC=x−3 तथा OD=4 है तो x का मान ज्ञात कीजिए ।
View SolutionIf OA=a, OB=b, OC=c are the co-terminus edges of regular parallelopiped, then the shortest distance between the diagonal and the side OA not meeting the diagonal is
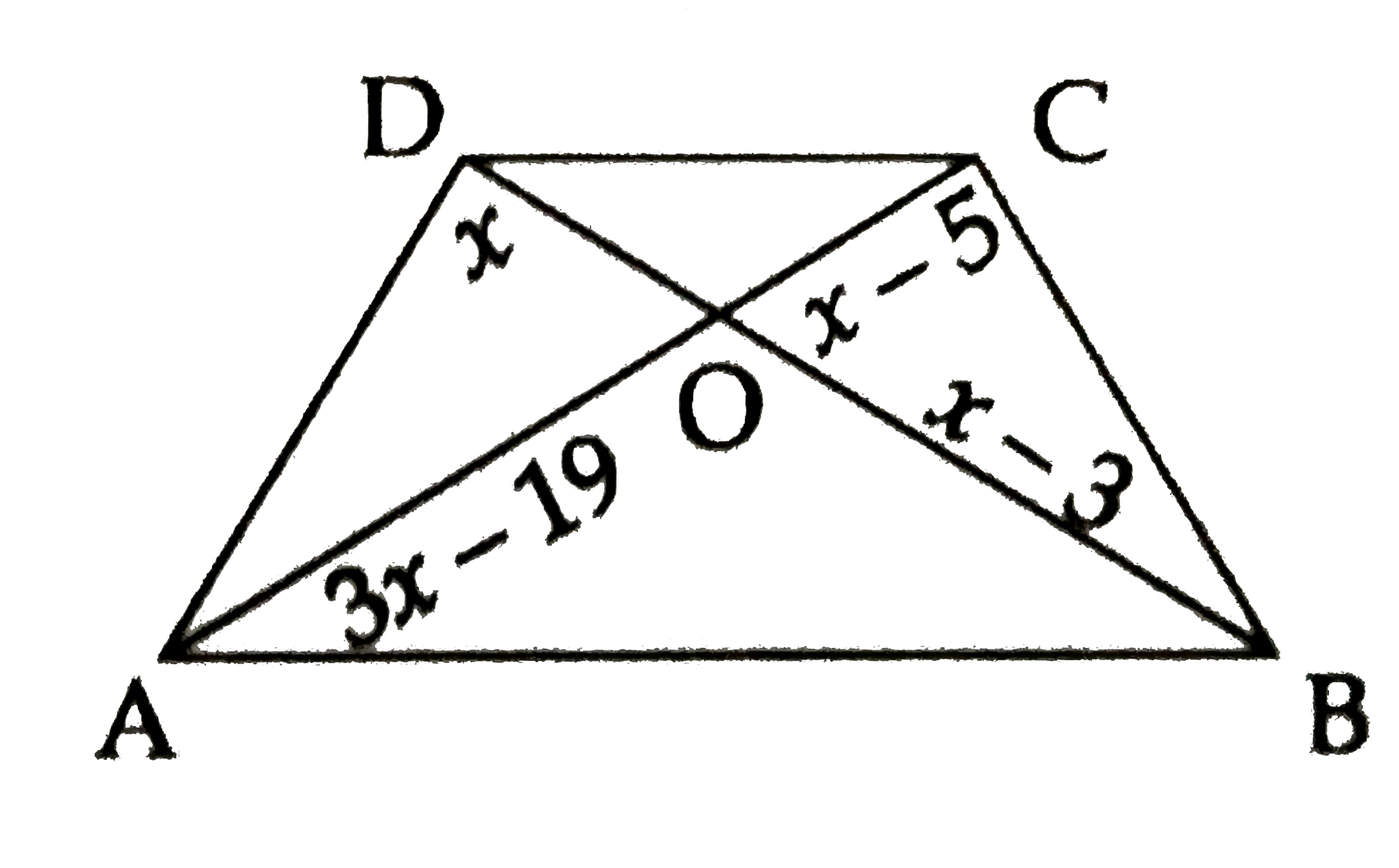
View SolutionIn the adjoining figure , seg AB || side DC , OD = x OB = x -3 , OC = x-5 , OA = 3x -19. Find the value of x .
View SolutionIn the adjacent figure, ABCD is a trapezium with AB || DC. Find the value of x. ( (i) If OA = 4x-2, OB = 4x + 1, OC = 2x + 2 and OD = 3x -1. (ii) If OA = 5x, OB = 7x -1, OC = 4x and OD = 5x + 1.
View Solutionदी गई आकृति में, AB के समान्तर DC है। यदि OA = 3x - 19, OB = x- 4, OC = x-3 तथा OD = 4 हो, तो x का मान ज्ञात कीजिए।
View Solutionआकृति में, ABCD एक समलम्ब चतुर्भुज हैं, जिसमें भुजा AB भुजा DC के समान्तर हैं तथा E भुजा AD का मध्य-बिंदु हैं। E भुजा BC पर इस प्रकार है खंड EF, भुजा DC के समान्तर हैं। सिद्ध कीजिए कि EF=12(AB+DC)
View Solutionकिसी समांतर चतुर्भुज ABCD में यदि विकर्णों का कटान-बिंदु O हो और OC = 6 सेमी, OB = 8 सेमी तो OA, OD, AC एवं BD के मान निकालिए।
View SolutionThe following figure shows a trapezium ABCD in which AB is parallel to DC and AD= BC
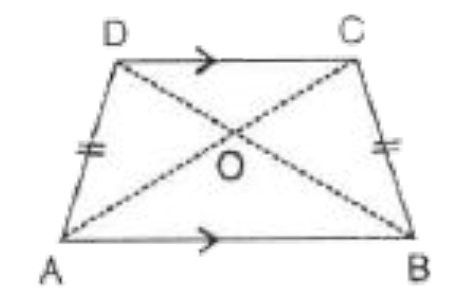
Prove that:
OA = OB and OC= OD
View SolutionAB and BD are two parallel sides of trapezium ABCD. if the diagonals AC and BD meet at O, then prove that (AO)/(OC) = (BO)/(OD) .
View SolutionThe side AB of a square ABCD is parallel to the x-axis. Find the slopes of all its sides.

Also, find :
the slope of the diagonal AC.
View Solution
