Let α,β,γ parametric angles of 3 points P,Q and R respectively lying on x2+y2=1. If the length of chords AP, AQ and AR are in GP where A is (-1,0), then [Given,α,β,γ∈(0,2π)]. Solution in Punjabi
The correct Answer is:A, D
Step by step video & image solution for Let alpha , beta , gamma parametric angles of 3 points P,Q and R respectively lying on x^(2)+y^(2)=1 . If the length of chords AP, AQ and AR are in GP where A is (-1,0), then [Given , alpha , beta ,gamma in (0,2pi)]. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Passage Based Questions)
13 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Trigonometric Equations and Inequations Exercise 3 :Passage Based Questions
1 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Single Option Correct Type Questions)
60 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam
2 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
Similar Questions
If number of solution and sum of solution of the equation 3sin2x−7sinx+2=0,x∈[0,2π] are respectively N and S and fn(θ)=sinnθ+cosnθ. On the basis of above information , answer the following questions.
Value of N is
View SolutionIf an angle and a side of a right angle triangle is known , then rest of the sides and angles can be found as follows
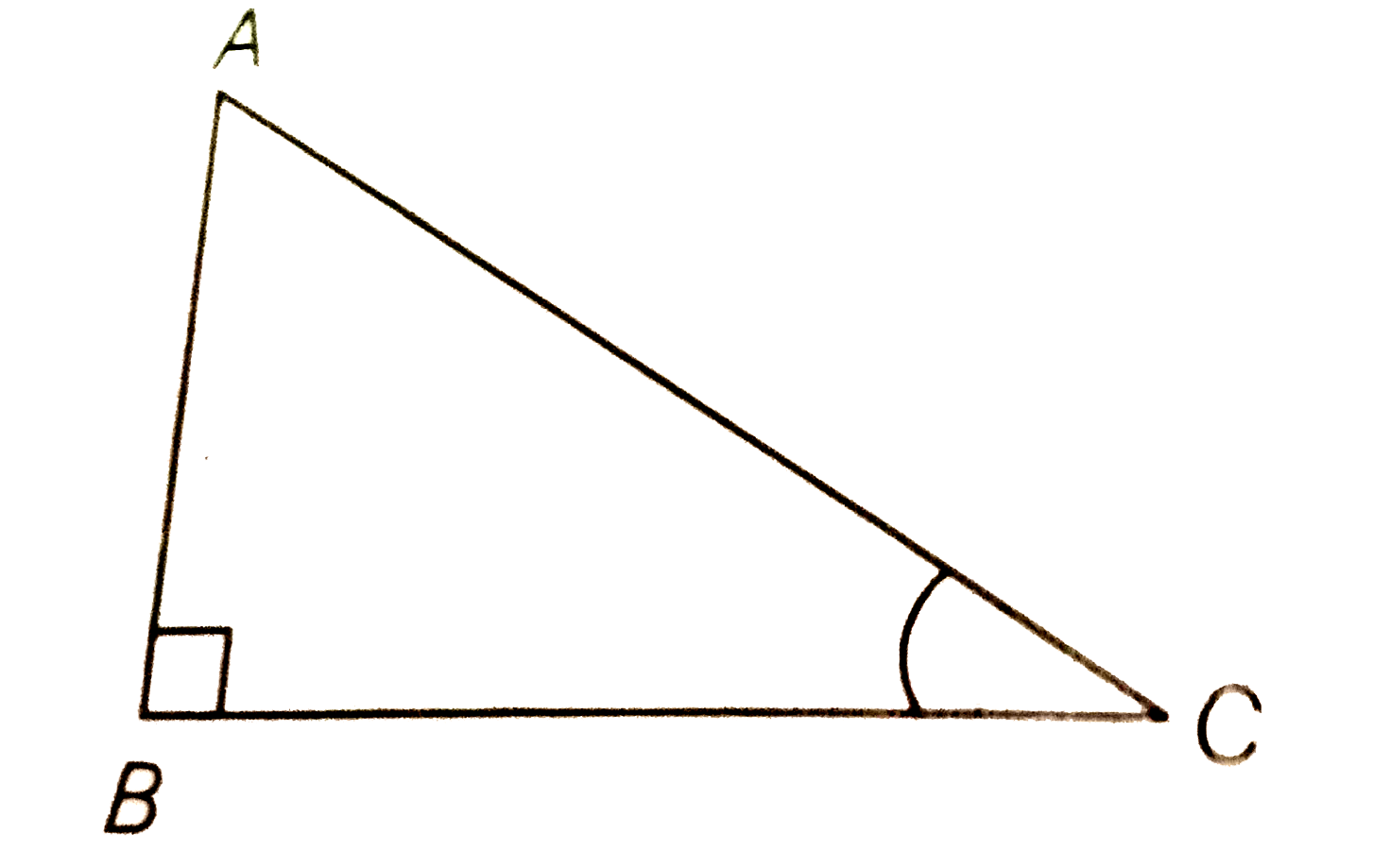
In ΔABC(figure ), if ∠B=90∘,∠C=θandBC=x, then AB=xtanθandAC=xsecθ .
Now, consider in isosceles triangle PQR (Figure 2),
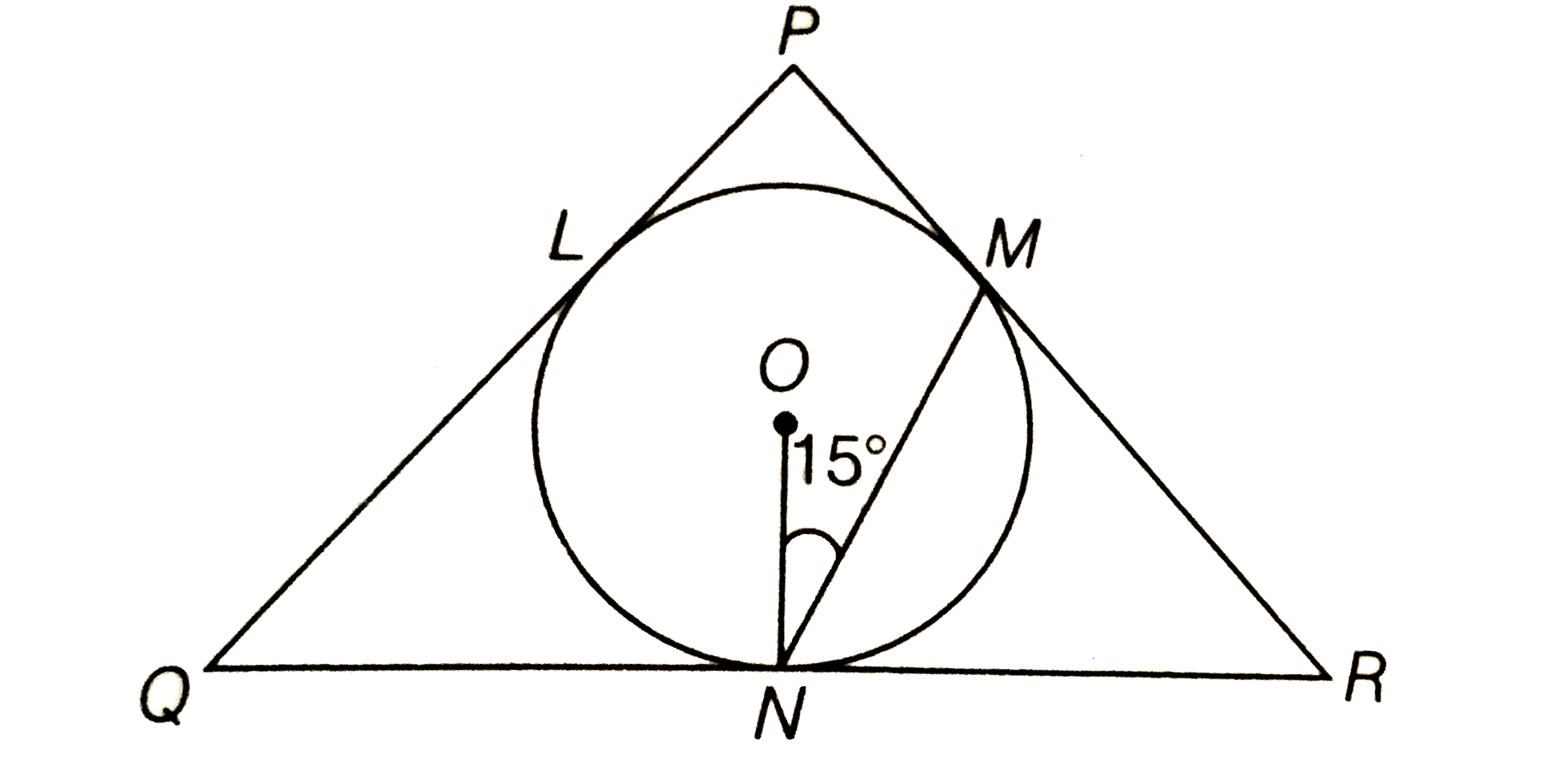
Where PQ=PR and 20N=√3
On the basis of the above information answer the question
The angle of triangle PQR are
View SolutionShow that sinx−sinycosx+cosy=tan(x−y2)
View SolutionConsider the line L1:x−12=y−1=z+31,L2:x−41=y+31=z+32 find the angle between them.
View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
View SolutionProve that |[alpha,beta,gamma] ,[alpha^2,beta^2,gamma^2] , [beta+gamma, gamma+alpha, beta+alpha]| = (alpha-beta)(beta-gamma)(gamma-alpha)(alpha+beta+gamma)
View Solutionalpha,beta and gamma are parametric angles of three points P,Q and R respectively,on the circle x^(2)+y^(2)=1 and A is the point (-1,0). If the lengths of the chords AP,AQ and AR are in GP, then (cos alpha)/(2),(cos beta)/(2) and (cos gamma)/(2) are in
View Solutionसाबित करे कि
∣∣ ∣ ∣∣αβγα2β2γ2β+γγ+αα+β∣∣ ∣ ∣∣=(β−γ)(γ−α)(α−β)(α+β+γ).
View Solutionयदि α,β,γ बहुपद f(x)=x3−px2+qx−r के शून्यक हो, तो 1αβ+1βγ+1γα=
View SolutionLet ,α,β,γ be the roots of
x3+px2+qx+r=0. Then find the
(v) (α+β)(β+γ)(γ+α)
View SolutionProve that, ∣∣ ∣ ∣∣αβγα2β2γ2β+γγ+αα+β∣∣ ∣ ∣∣=(α−β)(β−γ)(γ−α)(α+β+γ)
View SolutionIf α,β,γ are the roots of x3+3px+q=0 then the equation whose roots are α+1β+γ−α,β+1γ+α−βandγ+1α+β−γ is
View SolutionLet P(α,β,γ)andQ(1,−1,0) be points such that the mid-point of PQ is R(x,y,z). If x is the AM of αandβ,y is the G.M. of βandγandz is the H.M . Of γandα then :
View SolutionSuppose α,β,γ are the roots of x3+x2+x+2=0 then the value of (α+β−2γγ)(β+γ−2αα)(γ+α−2ββ)
View SolutionProve that ∣∣ ∣ ∣∣⎡⎢⎣αβγα2β2γ2α3β3γ3⎤⎥⎦∣∣ ∣ ∣∣=αβγ(α−β)(β−γ)(γ−α)
View Solutionਡਿਟਰਮੀਨੈਂਟ ਦੀਆਂ ਵਿਸ਼ੇਸ਼ਤਾਵਾਂ ਦਾ ਪ੍ਰਯੋਗ ਕਰਕੇ ਸਿੱਧ ਕਰੋ ਕਿ : ∣∣ ∣ ∣∣αα2β+γββ2γ+αγγ2α+β∣∣ ∣ ∣∣=(β−γ)(γ−α)(α−β)(α+β+γ)
View Solution
