Question

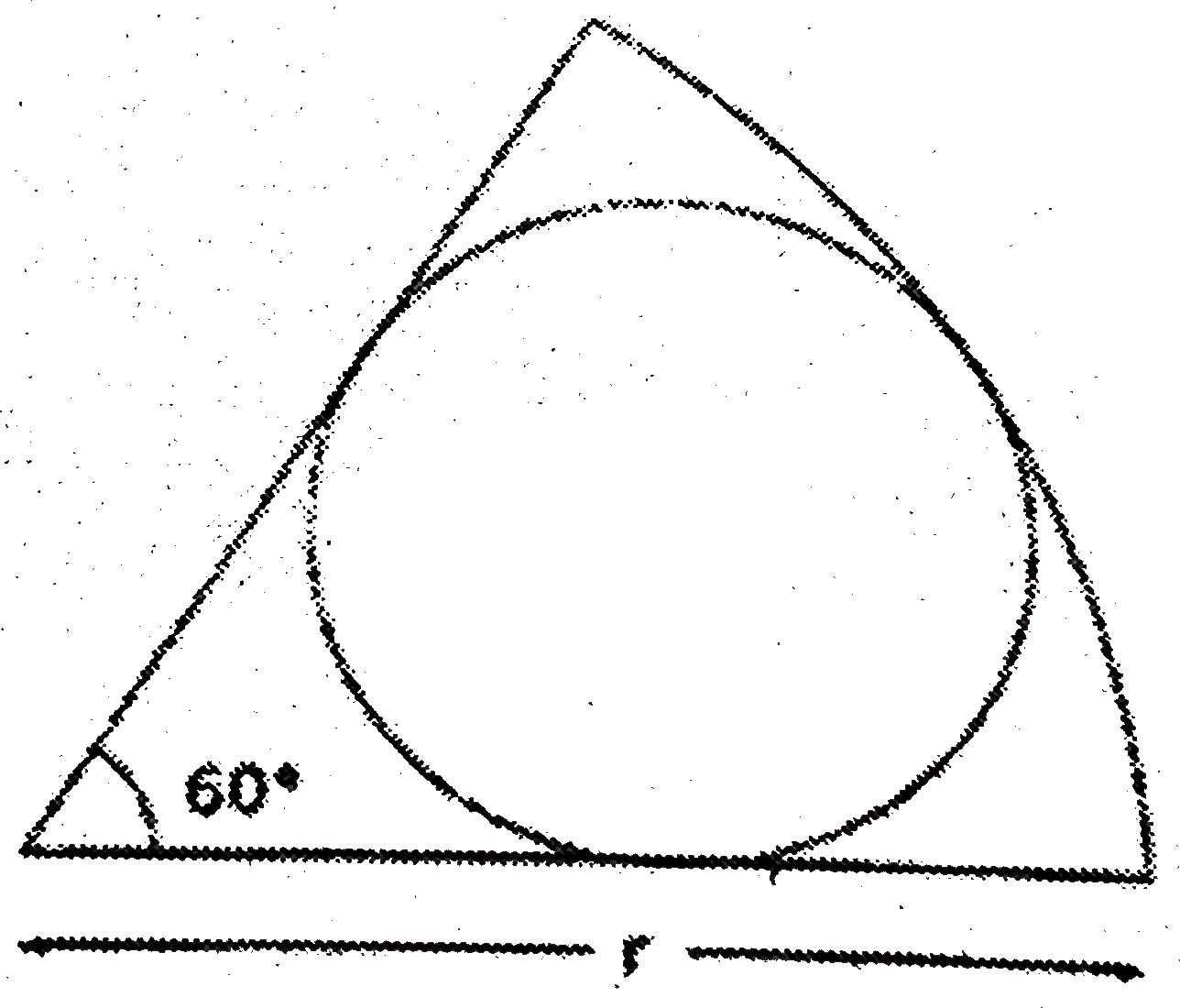
If inside a big circle exactly $n(n\le 3)$
small circles, each of radius $r,$
can be drawn in such a way that each small circle touches the big
circle and also touches both its adjacent small circles, then the radius of
big circle is
(a) $r(1+\cos ec\frac{\pi }{n})$
(b) $\left(\frac{1+\frac{\tan \pi }{n}}{\frac{\cos \pi }{\pi }}\right)$

(c) $r[1+\cos ec\frac{2\pi }{n}]$
(d) $\frac{r{[s\in \frac{\pi }{2n}+\frac{\cos \left(2\pi \right)}{n}]}^2}{\frac{\sin \pi }{n}}$

Options

A. `r(1+ cosec (pi)/(n))`
B. `((1+tan""(pi)/(n ))/(cos ""(pi)/(n )))`
C. `r[1+cosec (2pi)/(n)]`
D. `(r [sin ""(pi)/(2n)+ cos ""(2pi)/(n)]^(2))/(sin "'(pi)/(n))`

Answer

A::D