A spacecraft which is moving with a speed u relative to the earth in the x-direction, enters the gravitational field of a much more massive planet which is moving with a speed 3u in the negative x-direction. The spacecraft exits following the trajectory as shown below.

The speed of the spacecraft with respect to the earth a long time after it has escaped the planet's gravity is given by

A

u

B

4u

C

2u

D

7u

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

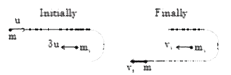
from momentum conservation
$- μ + m_{13} u = m_{1} v_{1} + m v_{2}$ ......(1)
from energy conservation
$\frac{1}{2} μ^{2} + \frac{1}{2} m_{19} u^{2} = \frac{1}{2} m_{1} v_{1}^{2} + \frac{1}{2} m v_{2}^{2}$
$\frac{1}{2} μ^{2} + \frac{1}{2} m_{1} (3 u - v_{1}) (3 u + v_{1}) = \frac{1}{2} m v_{2}^{2}$
from equation ...(1)
$\Rightarrow m_{1} (3 u - v_{1}) = m (v_{2} + u)$
$\frac{1}{2} μ^{2} + \frac{1}{2} m (v_{2} + u) (3 u + v_{1}) = \frac{1}{2} m v_{2}^{2}$
as $m_{1} > > > m$ , we can assume $v_{1} \approx 3 u$
$u^{2} + (v_{2} + u) (6 u) = v_{2}^{2}$
$\Rightarrow v_{2} = 7 u$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A spacecraft is launched in a circular orbit very close to earth. What additional velocity should be given to the spacecraft so that it might escape the earth's gravitational pull.
A $20.2 K m s^{- 1}$
B $3.25 K m s^{- 1}$
C $8 K m s^{- 1}$
D $11.2 K m s^{- 1}$
Question 2 - Select One
The mass of a planet is twice the mass of earth and diameter of the planet is thrie the diameter of the earth, then the acceleration due to gravity on the planet's surface is
A $g / 2$
B $2 g$
C $2 g / 9$
D $3 g / \sqrt{2}$
Question 3 - Select One
The mass of a planet is six times that of the earth. The radius of the planet is twice that of the earth. It's the escape velocity from the earth is $v$ , then the escape velocity from the planet is:
A $\sqrt{3} v$
B $\sqrt{2} v$
C $\sqrt{5} v$
D $\sqrt{12} v$

KVPY PREVIOUS YEAR-QUESTION PAPER 2020-PART-I : PHYSICS

Home
Ask Doubt
Profile