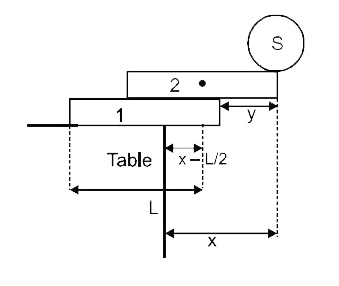
Two identical uniform rectangular blocks (with longest side L) and a solid sphere of radius R are to be balanced at the edge of a heavy table such that the centre of the sphere remains at the maximum possible horizontal distance from the vertical edge of the table without toppling as indicated in the figure.
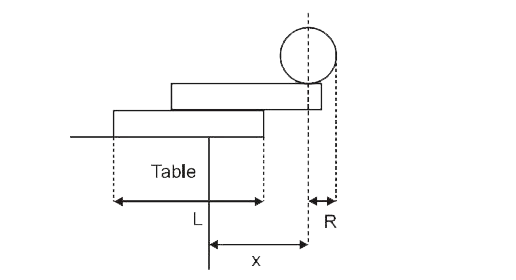
If the mass of each block is M and of the sphere is M/2, then the maximum distance x that can be achieved is
8L/15
5L/6
(3L/4 + R)
(7L/15 + R)
The correct Answer is:A
2+ S System lie above edge of 1
M2y−M(L2−y)=0
y2+y=L2
y=L3
Now 1 + 2 + S centre of mass will lie above the table .
3M2(x−L3)+M(x−L3−L2)=0
3x2−L2+x−L3−L2=0
5x2=4L3 x=8L15
Topper's Solved these Questions
- QUESTION PAPER 2013BOOK - KVPY PREVIOUS YEARCHAPTER - QUESTION PAPER 2013EXERCISE - PART-I ( PHYSICS)
20 Videos
- QUESTION PAPER 2013BOOK - KVPY PREVIOUS YEARCHAPTER - QUESTION PAPER 2013EXERCISE - PART-II ( PHYSICS)
10 Videos
- QUESTION PAPER 2013BOOK - KVPY PREVIOUS YEARCHAPTER - QUESTION PAPER 2013EXERCISE - PART-II ( PHYSICS)
10 Videos
- MOCK TEST 9BOOK - KVPY PREVIOUS YEARCHAPTER - MOCK TEST 9EXERCISE - EXERCISE
19 Videos
- QUESTION PAPER 2020BOOK - KVPY PREVIOUS YEARCHAPTER - QUESTION PAPER 2020EXERCISE - PART-I : PHYSICS
50 Videos
Similar Questions
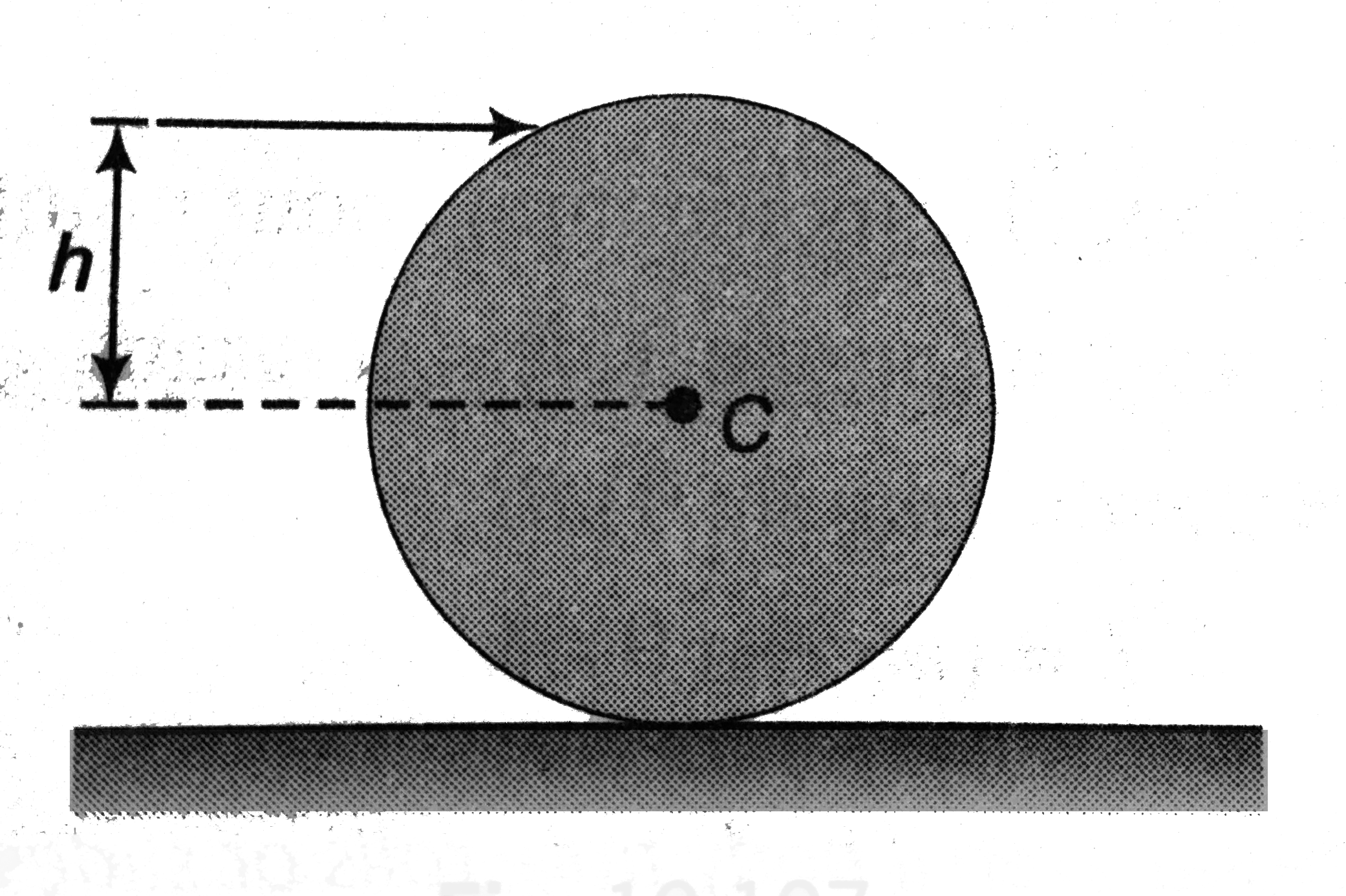
A solid sphere of mass M and radius R is hit by a cue at a height h above the centre C. for what value of h the sphere will rool without slipping ?
View SolutionTwo identical spheres each of mass M and Radius R are separated by a distance 10R. The gravitational force on mass m placed at the midpoint of the line joining the centres of the spheres is
View Solution
Knowledge Check
The radius of gyration of a solid sphere of radius r about a certain axis is r. The distance of this axis from the centre of the sphere is
ArB0.5 rC√0.6rD√0.4rA spherical hole is made in a solid sphere of radius R. The mass of the sphere before hollowing was M. The gravitational field at the centre of the hole due to the remaining mass is
 AzeroBGM8R2CGM2R2DGMR2
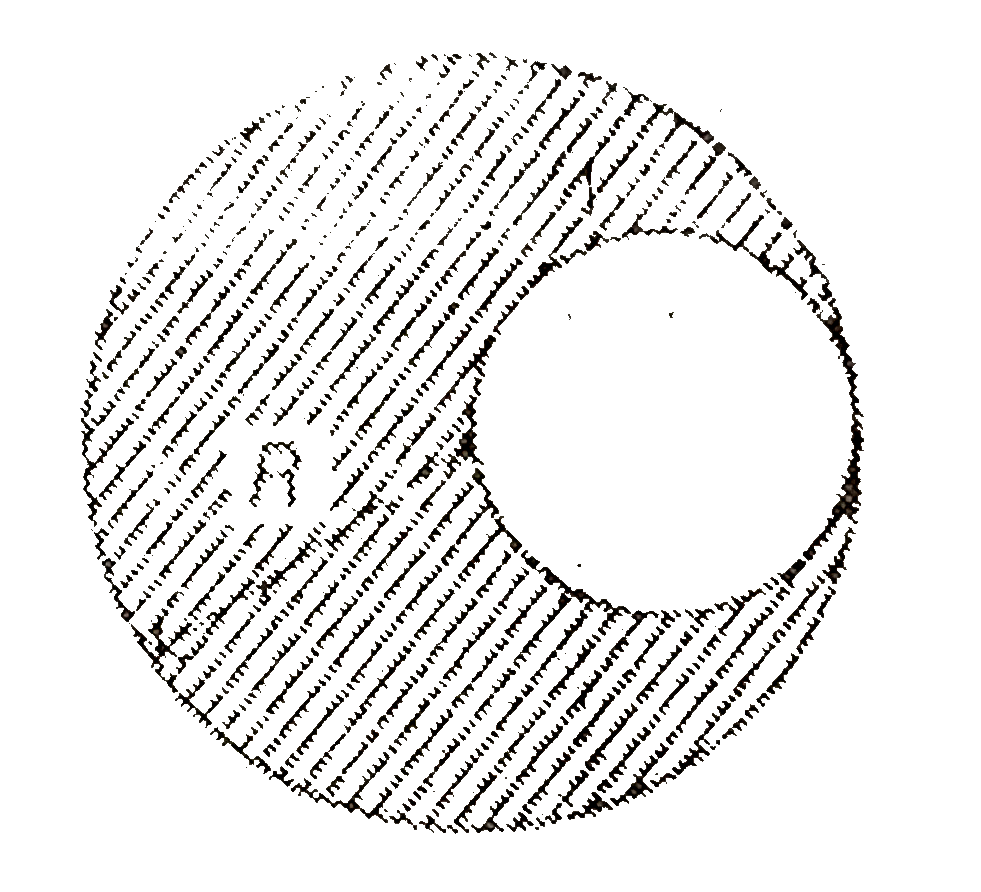
AzeroBGM8R2CGM2R2DGMR2A hemispherical cavity of radius R is created in a solid sphere of radius 2R as shown in the figure . Then y -coordinate of the centre of mass of the remaining sphere is
 AYCM=−R15BYCM=−R40CYCM=−R30DYCM=−R20
AYCM=−R15BYCM=−R40CYCM=−R30DYCM=−R20
The gravitational field due to an uniform solid sphere of mass M and radius a at the centre of the sphere is
View SolutionA uniform rope of length l lies on a table. If the coefficient of friction is μ, then the maximum length L of the part of this rope which can overhang from the edge of the table without sliding down is
View SolutionA uniform solid sphere of radius R has a cavity of radius 1m cut from it if centre of mass of the system lies at the periphery of the cavity then

View SolutionA uniform rope of length l lies on a table . If the coefficient of friction is μ , then the maximum length l1 of the part of this rope which can overhang from the edge of the table without sliding down is :
View SolutionA uniform rope of length l lies on a table if the coefficient of friction is , then the maximum length l, of the part of this rope which can over hang from the edge of the table without sliding down is -
View Solution
