Quadrilateral ABCD is circumscribed about a circle. If the lengths of AB, BC, CD are 7cm, 8.5 cm and 9.2 cm respectively then the length (in cm) of DA is.
16.2
7.7
7.2
10.7
To find the length of side DA in the circumscribed quadrilateral ABCD, we can use the property of tangential quadrilaterals. In a tangential quadrilateral, the sum of the lengths of opposite sides is equal. This means:
AB + CD = BC + DA
Given:
- AB = 7 cm
- BC = 8.5 cm
- CD = 9.2 cm
- DA = ?
We can substitute the known values into the equation:
1. Step 1: Write the equation based on the property of tangential quadrilaterals.
AB + CD = BC + DA
Substituting the known lengths:
7 cm + 9.2 cm = 8.5 cm + DA
2. Step 2: Calculate the left side of the equation.
7 cm + 9.2 cm = 16.2 cm
So, we have:
16.2 cm = 8.5 cm + DA
3. Step 3: Isolate DA.
To find DA, we can rearrange the equation:
DA = 16.2 cm - 8.5 cm
4. Step 4: Perform the subtraction.
DA = 16.2 cm - 8.5 cm = 7.7 cm
Thus, the length of side DA is 7.7 cm.
- AB = 7 cm
- BC = 8.5 cm
- CD = 9.2 cm
- DA = ?
Topper's Solved these Questions
Similar Questions
A quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB+CD=AD+BC
View SolutionIn a ΔABC, D, E and F are the midpoints of BC, CA and AB respectively. If the lengths of side AB, BC and CA are 7 cm, 8 cm and 9 cm respectively, the perimeter of ΔDEF is a cm. The value of a/2 is
View Solution
Knowledge Check
Quadrilateral ABCD is circumscribed to a circle. If AB=6cm, BC=7cm and CD=4cm then the length of AD is
A3cmB4cmC6cmD7cmQuadrilateral ABCD circumscribes a circle. If AB = 8 cm, BC = 7 cm and CD = 6 cm, then the length of AD is:
A7 cmB6.8 cmC7.5 cmD6 cmA quadrilateral ABCD is drawn to circumscribe a circle such that its sides. AB , BC ,CD and DA touch the circle at P,Q,R and S , respectively . If AB = x cm BC = 7 cm , CR = 3 cm and AS = 5 cm , then x= ?
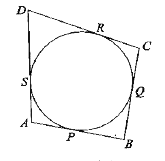 A10 cmB8 cmC9 cmD7 cm
A10 cmB8 cmC9 cmD7 cm
In figure,a right triangle ABC,circumscribes a circle of radius r.If AB and BC are of length 8 cm and 6 cm respectively, find the value of r.
View SolutionIn figure, a quadrilateral ABCD is drawn to circumscribe a circle such that its sides AB, BC, CD and AD touch the circle at P, Q, R and S respectively. If AB = x cm, BC = 7 cm, CR = 3cm and AS = 5cm, then x = ______.

View SolutionIn a ΔABC,D,EandF are respectively the mid-points of BC, CA and AB. If the lengths of side AB, BC and CA are 7 cm, 8 cm and 9 cm respectively, find the permeter of ΔDEF.
View SolutionABCD is a quadrilateral AC=19 cm. The lengths of perpendicular from B and D on AC are 5 cm and 7 cm respectively . Then , the area of ABCD ( in cm2) is :
View SolutionIf in ΔABC,DE∣∣BC,AB=7.5cm,BD=6cm and DE=2cm, then the length of BC in cm is :
View Solution