Assertion: The velocity of flow of a liquid is smaller when pressure is larger and vice versa.
Reason: According to Bernoulli's theorem, for the stream line flow of an ideal liquid, the total energy per unit mass remains constant.
Statement-1, is true, Statement-2 is true, Statement-2 is correct explanation for statement-1
Statement-1 is true, statement-2 is true, statement-2 is NOT a correct explantion for statement-1
statement-1 is true, statement-2 is false
statement-1 is false statement-2 is true
The correct Answer is:A
To solve the question, we need to analyze the assertion and reason provided and apply Bernoulli's theorem.
1. Understanding the Assertion:
The assertion states that "The velocity of flow of a liquid is smaller when pressure is larger and vice versa." This implies an inverse relationship between pressure and velocity in a fluid flow.
2. Understanding the Reason:
The reason states that "According to Bernoulli's theorem, for the streamline flow of an ideal liquid, the total energy per unit mass remains constant." Bernoulli's theorem can be expressed as:
P+12ρV2+ρgh=constant
where P is the pressure, ρ is the density of the fluid, V is the velocity, and h is the height above a reference level.
3. Applying Bernoulli's Theorem:
For a horizontal pipe, the height h remains constant, so we can simplify Bernoulli's equation to:
P1+12ρV21=P2+12ρV22
Rearranging gives:
P2=P1+12ρ(V21−V22)
4. Analyzing the Relationship:
If we assume V1<V2 (the velocity at point 1 is less than at point 2), then V21<V22. This means that V21−V22 is negative, leading to:
P2<P1
This indicates that when the velocity increases (from V1 to V2), the pressure decreases (from P1 to P2), confirming the assertion.
5. Conclusion:
Both the assertion and the reason are true. The assertion correctly describes the relationship between pressure and velocity in fluid flow, and the reason accurately reflects Bernoulli's theorem.
Final Answer:
Both the assertion and the reason are true, and the correct option is that both statements are valid.
---
The assertion states that "The velocity of flow of a liquid is smaller when pressure is larger and vice versa." This implies an inverse relationship between pressure and velocity in a fluid flow.
The reason states that "According to Bernoulli's theorem, for the streamline flow of an ideal liquid, the total energy per unit mass remains constant." Bernoulli's theorem can be expressed as:
P+12ρV2+ρgh=constant
where P is the pressure, ρ is the density of the fluid, V is the velocity, and h is the height above a reference level.
For a horizontal pipe, the height h remains constant, so we can simplify Bernoulli's equation to:
P1+12ρV21=P2+12ρV22
Rearranging gives:
P2=P1+12ρ(V21−V22)
If we assume V1<V2 (the velocity at point 1 is less than at point 2), then V21<V22. This means that V21−V22 is negative, leading to:
P2<P1
This indicates that when the velocity increases (from V1 to V2), the pressure decreases (from P1 to P2), confirming the assertion.
Both the assertion and the reason are true. The assertion correctly describes the relationship between pressure and velocity in fluid flow, and the reason accurately reflects Bernoulli's theorem.
Both the assertion and the reason are true, and the correct option is that both statements are valid.
Topper's Solved these Questions
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 3 (Comprehension based questions)
24 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 4 A (Elasticity)
7 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 2 (One or more than one correct answer)
53 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
Similar Questions
Assertion : When an ideal fluid flows through a pipe of non-uniform cross-section, then pressure is more at that section where area is more if the pipe is horizontal.
Reason : According to Bernoulli's theorem speed at broader cross-section will be less.
View SolutionAssertion: An ideal fluid is flowing through a pipe. Speed of fluid particles is more at places where pressure is low.
Reason: Bernoulli's theoren can be derived from work-energy theorem.
(A)Both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
(B)Both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
(C)Assertion is true, but the Reason is false.
(D)Assertion is false but the Reason is true.
View Solution
Knowledge Check
Assertion: Bernoulli's equation hold for non-steady or turbulent flows.
Reason: In these situations, velocity and pressure are constant with time.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true and reason is false.DIf both assertion and reason are false.Assertion :When height of a tube is less than liquid rise int eh capillary tube, the liquid does not overflow.
Reason : Product of radius of meniscus and height of liquid in the capilliary tube always remains constant.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true and reason is false.DIf both assertion and reason are false.
Fluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.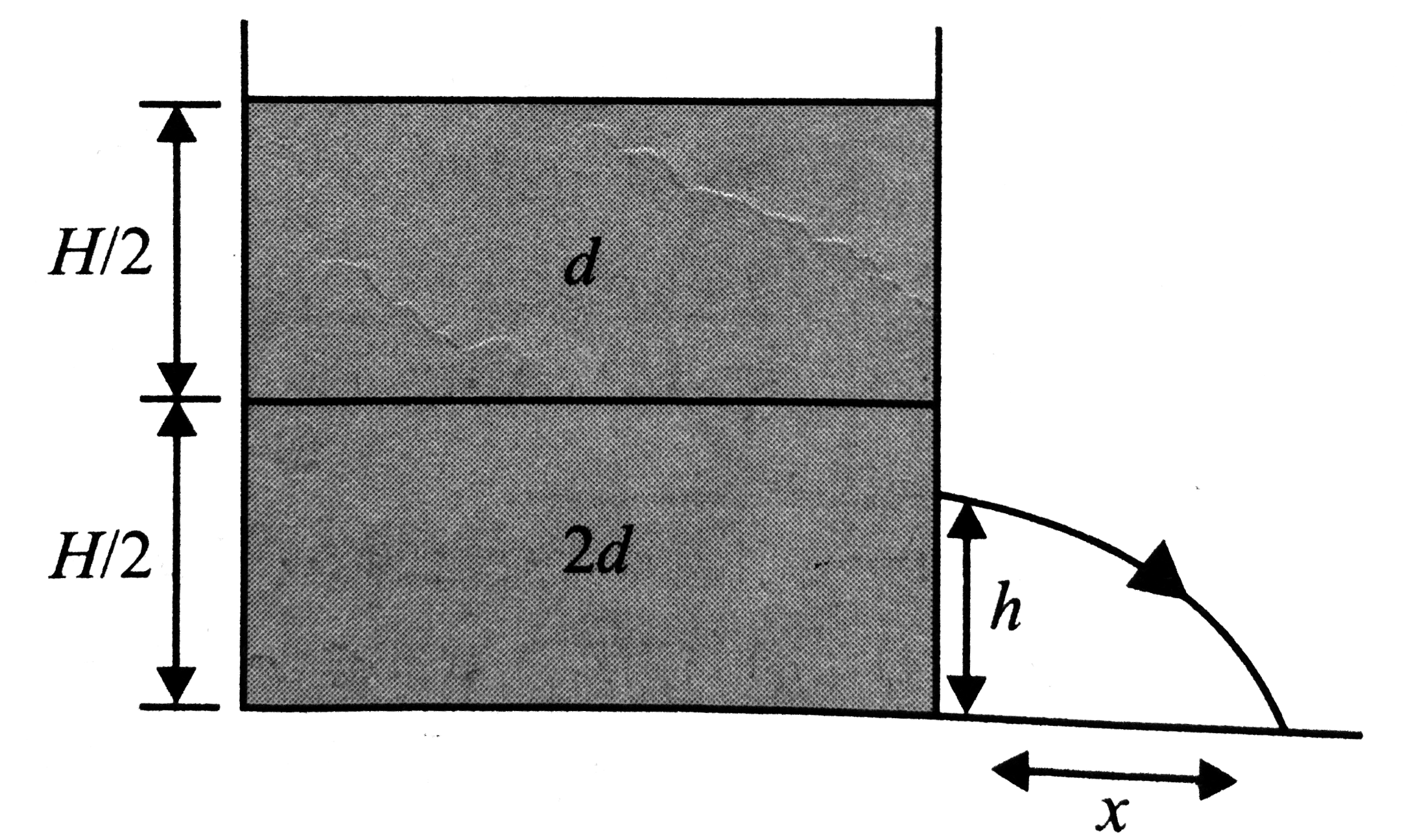
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The total pressure at the bottom of the container is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the Body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.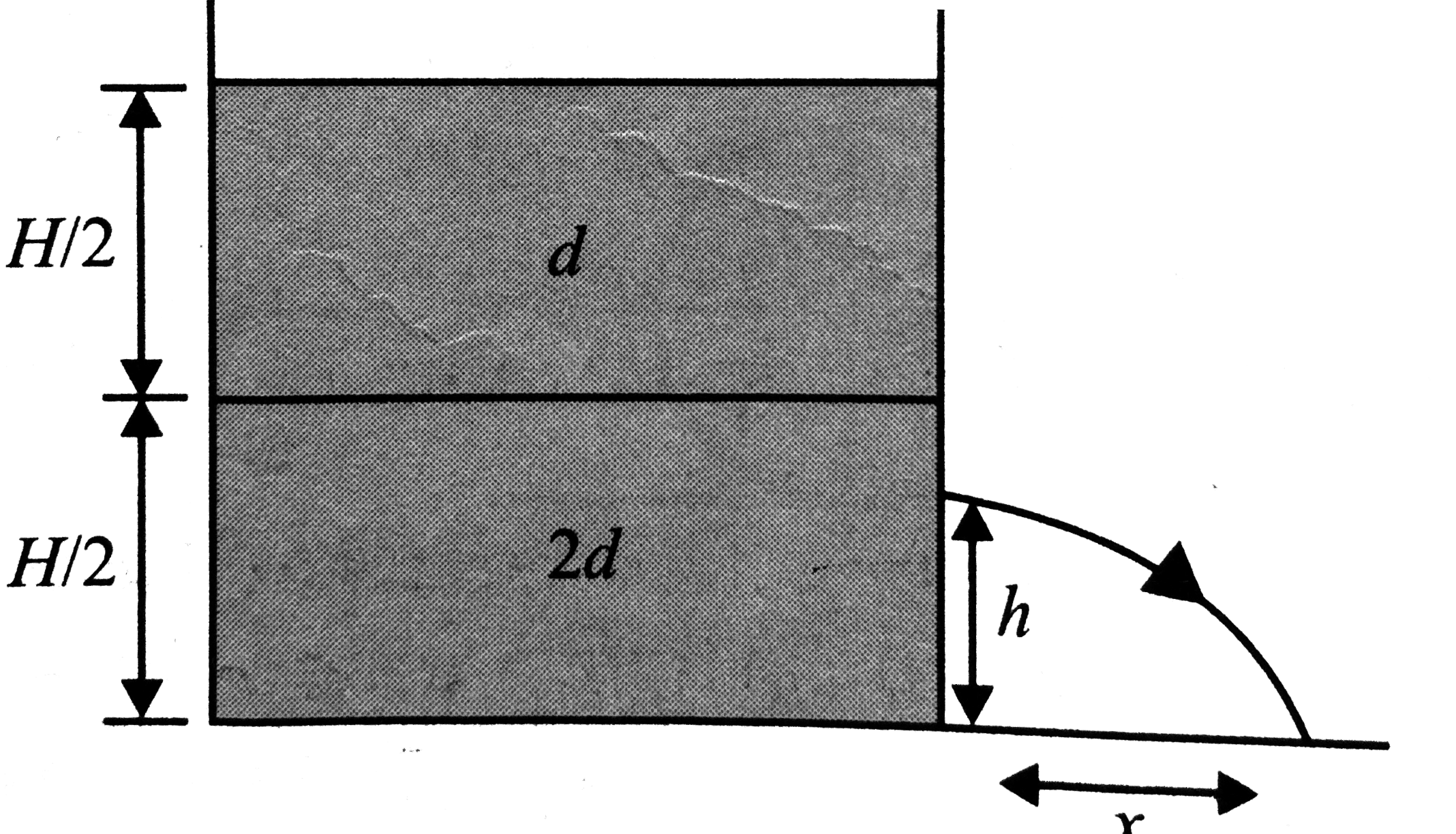
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The density of the solid is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.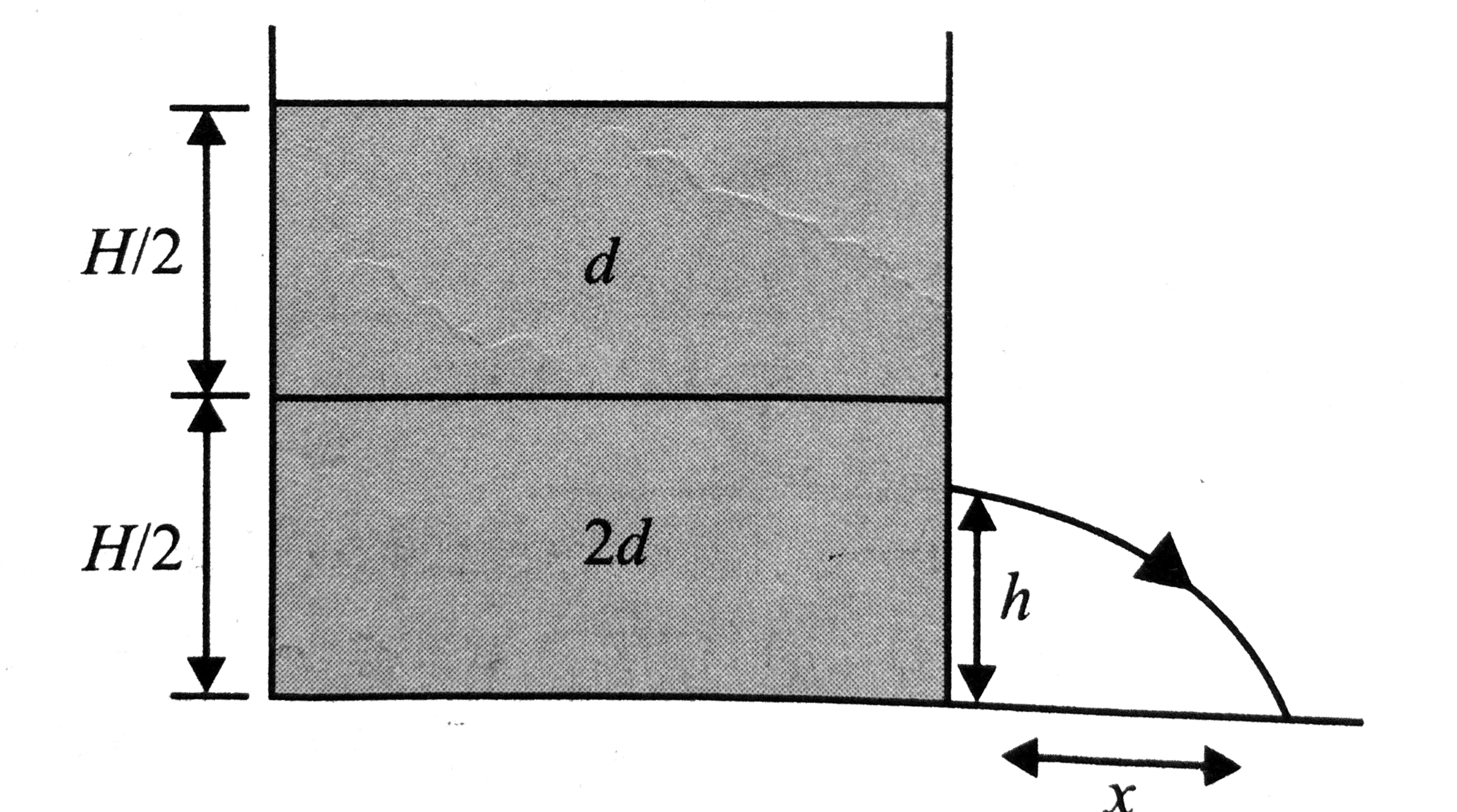
Situation II:
A cyliner is removed and the original arrangement is restoreed.A tiny hole of area s(s<<A) is punched on the veritical sideof the containier at a height h(h<H/2)
The horizontal distance x travelled by the liquid is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform cross sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.
Situation II:
A cyliner is removed and the original arrangement is restored.A tiny hole of area s(s<<A) is punched on the veritical sideof the containier at a height h(h<H/2)
The initial speed of efflux of the liquid at the hole is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.
Situation II:
A cyliner is removed and the original arrangement is restoreed.A tiny hole of area s(s<<A) is punched on the veritical side of the containier at a height h(h<H/2)
The height hm at which the hole should be punched so that the liquid travels the maximum distance is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the ody immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.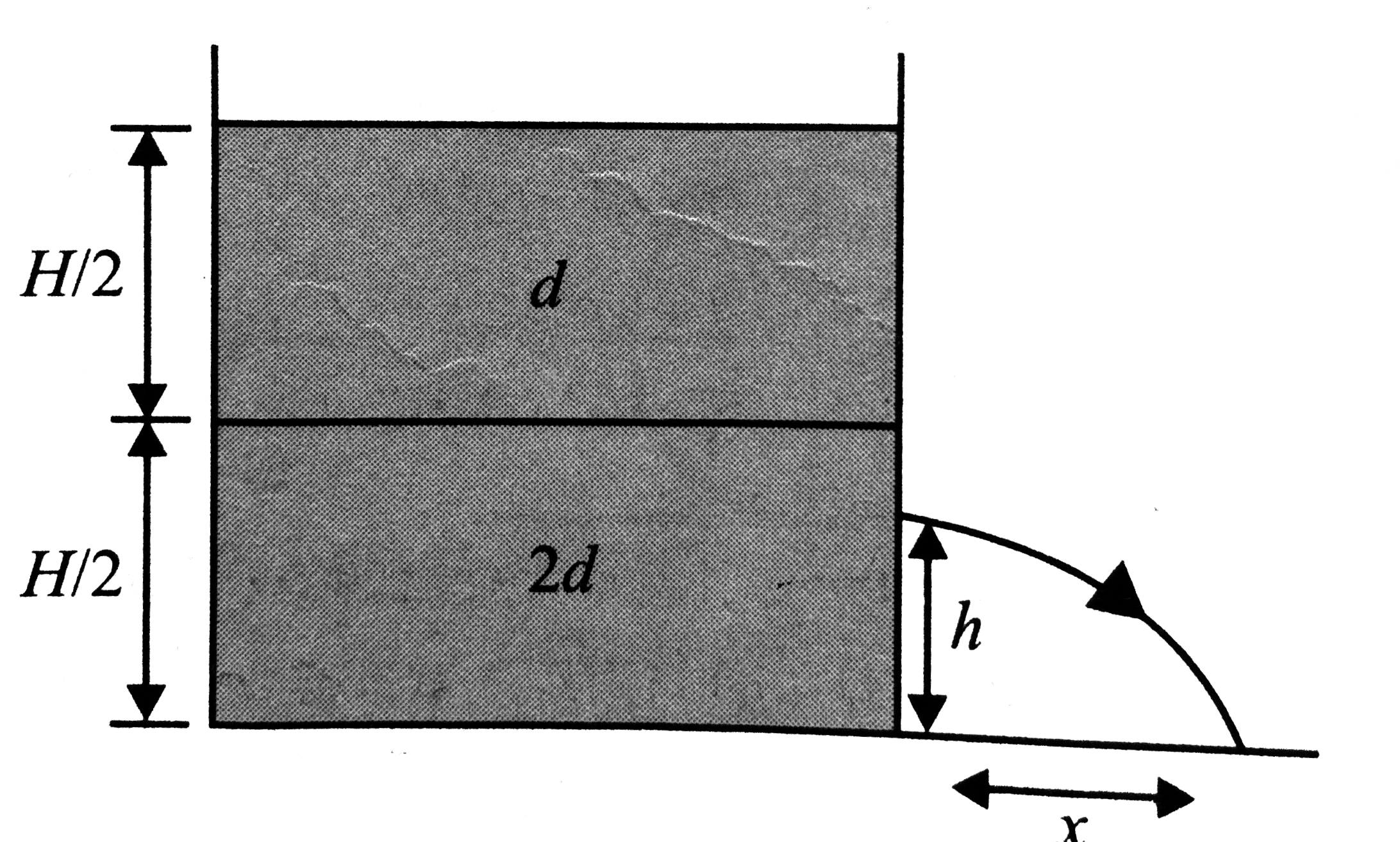
Situation II:
A cyliner is removed and the original arrangement is restoreed.A tiny hole of area s(s<<A) is punched on the veritical side of the container at a height h(h<H/2)
The maximum distance travelled xm is
View Solution
