A thin spherical shell of total mass M and radius R is held fixed. There is a small hole in the shell. A mass m is released from rest a distance R from the hole along a line that passes through the hole and also through the centre of the shell. This mass subsequently moves under the gravitational force of the shell. How long does the mass take to travel from the hole to the point diametrically opposite.
The correct Answer is:2√R3GM
To solve the problem, we need to analyze the motion of the mass m as it travels from the hole in the spherical shell to the point diametrically opposite. The solution can be broken down into two parts: the motion until it reaches the hole and the motion inside the shell.
Step 1: Analyze the motion until the hole
1. Initial Setup: The mass m is released from a distance R from the hole. The gravitational force acting on it is due to the mass M of the spherical shell.
2. Conservation of Energy: We can apply the conservation of mechanical energy. The initial potential energy (PE) when the mass is at distance R from the hole is given by:
\(
PE_i = -\frac{GMm}{R}
\)
where G is the gravitational constant.
When the mass reaches the hole (at a distance R2 from the center), the potential energy is:
\(
PE_f = -\frac{GMm}{R/2} = -\frac{2GMm}{R}
\)
3. Change in Potential Energy: The change in potential energy as the mass moves from the initial position to the hole is:
\(
\Delta PE = PE_f - PE_i = -\frac{2GMm}{R} + \frac{GMm}{R} = -\frac{GMm}{R}
\)
4. Kinetic Energy: The change in kinetic energy (KE) is equal to the negative change in potential energy:
\(
KE = \Delta PE = -\frac{GMm}{R}
\)
Since the mass starts from rest, its initial kinetic energy is zero:
\(
\frac{1}{2}mv^2 = \frac{GMm}{R}
\)
Solving for v, we get:
\(
v = \sqrt{\frac{2GM}{R}}
\)
Step 2: Analyze the motion inside the shell
1. Motion Inside the Shell: Inside a thin spherical shell, the gravitational force is zero, and the potential energy is constant. Therefore, the mass will continue to move with the velocity it had when it reached the hole.
2. Distance to Travel: The distance from the hole to the diametrically opposite point is:
\(
d = 2R
\)
3. Time Calculation: The time t taken to travel this distance at constant speed v is given by:
\(
t = \frac{d}{v} = \frac{2R}{\sqrt{\frac{2GM}{R}}}
\)
Simplifying this, we find:
\(
t = 2R \cdot \sqrt{\frac{R}{2GM}} = 2\sqrt{\frac{R^3}{2GM}}
\)
Final Result
Thus, the total time taken for the mass m to travel from the hole to the point diametrically opposite is:
\(
t = 2\sqrt{\frac{R^3}{2GM}}
\)
\(
PE_i = -\frac{GMm}{R}
\)
where G is the gravitational constant.
\(
PE_f = -\frac{GMm}{R/2} = -\frac{2GMm}{R}
\)
\(
\Delta PE = PE_f - PE_i = -\frac{2GMm}{R} + \frac{GMm}{R} = -\frac{GMm}{R}
\)
\(
KE = \Delta PE = -\frac{GMm}{R}
\)
Since the mass starts from rest, its initial kinetic energy is zero:
\(
\frac{1}{2}mv^2 = \frac{GMm}{R}
\)
Solving for v, we get:
\(
v = \sqrt{\frac{2GM}{R}}
\)
\(
d = 2R
\)
\(
t = \frac{d}{v} = \frac{2R}{\sqrt{\frac{2GM}{R}}}
\)
Simplifying this, we find:
\(
t = 2R \cdot \sqrt{\frac{R}{2GM}} = 2\sqrt{\frac{R^3}{2GM}}
\)
\(
t = 2\sqrt{\frac{R^3}{2GM}}
\)
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 4 B (Brain Storming Subjective Exercise)
20 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 5 A (Previous Year Questions)
26 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 3 (Miscellaneous Type Questions)
20 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
Similar Questions
A thin spherical shell of mass M and radius R has a small hole. A particle of mass m released at its mouth. Then
View SolutionA ball of mass m and radius r rolls inside a hemispherical shell of radius R. It is released from rest from point A as shown in figure. The angular velocity of centre of the ball in position B about the centre of the shell is.
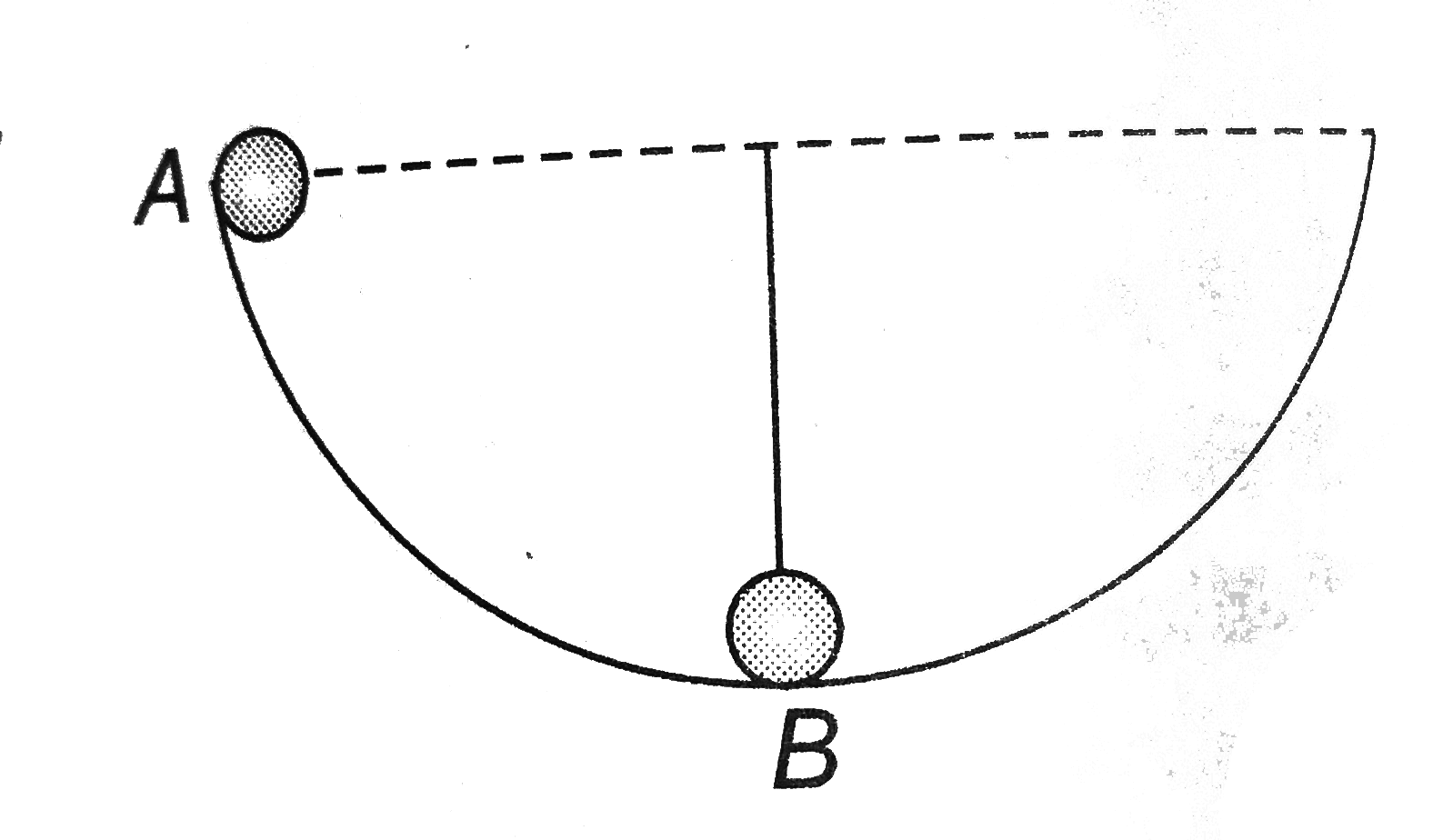 .
.
View Solution
Knowledge Check
A particle of mass m is placed at the centre of a unifrom spherical shell of mass 3 m and radius R The gravitational potential on the surface of the shell is .
AGMRB−GMRC−4GMRD−2GMRA point mass m is placed inside a spherical shell of radius R and mass M at a distance R2 form the centre of the shell. The gravitational force exerted by the shell on the point mass is
AGMmR2B2GMmR2CzeroD4MmR2
A body of mass m is placed at the centre of the spherical shell of radius R and mass M. The gravitation potential on the surface of the shell is
View SolutionFigure shows a hemispherical shell of mass M and radius R and a concentric shell of mass m and radius 3R/4.

View SolutionA small hole is made in a disc of mass M and radius R at a distance R/4 from centre. The disc is supported on a horizontal peg through this hole. The moment of inertia of the disc about horizontal peg is
View SolutionA point charge Q is placed inside a conducting spherical shell of inner radius 3R and outer radius 5R at a distance R from the centre of the shell. The electric potential at the centre of the shell will be
View SolutionA fixed spherical shell of inner radius R and outer radius 2R has mass M, A particle of mass m is held at rest at a distance 2R from the surface of the body. If the particle is released from that point, with what speed will it hit surface of the spherical body ?
View SolutionA solid sphere of mass m and radius r is placed inside a hollow thin spherical shell of mass M and radius R as shown in the figure. A particle of mass m' is placed on the line joining the two centres at a distance x from the point of contact of the sphere and the shell. Find the magnitude of the resultant gravitational force on this particle due to the sphere and the shell if

r<x<2r
View Solution