A metallic rod of length has one end free and other end rigidly clamped. Longitudinal stationary waves are set up in the rod in such a way that there are total six antinodes present along the rod. The amplitude of an antinode is . young's modulus and density of the rod are and respectively. Consider the free end to be at origin and at particles at free end are at positive extreme.
The equation describing stress developed in the rod is
The correct Answer is:B
To solve the problem, we need to derive the equation describing the stress developed in a metallic rod that is clamped at one end and has longitudinal stationary waves set up in it. Here’s a step-by-step solution:
Step 1: Understanding the Problem
We have a metallic rod of length L=1m with one end free and the other end clamped. There are 6 antinodes in the rod, and the amplitude of the antinode is given as A=4×10−6m. The Young's modulus Y is 6.4×1010N/m2 and the density ρ is 4×103kg/m3.
Step 2: Equation of the Stationary Wave
The equation for the stationary wave can be expressed as:
y(x,t)=Acos(kx)cos(ωt)
where k is the wave number and ω is the angular frequency.
Given that there are 6 antinodes, the wave number k can be calculated as:
k=nπL=6π1=6πrad/m
Thus, the equation becomes:
y(x,t)=4×10−6cos(6πx)cos(ωt)
Step 3: Finding the Angular Frequency
To find ω, we can use the relationship between the speed of sound v, Young's modulus Y, and density ρ:
v=√Yρ
Calculating v:
v=√6.4×10104×103=√1.6×107≈4000m/s
Now, using the relationship ω=vk:
ω=4000×6π=24000πrad/s
Thus, the equation of the stationary wave is:
y(x,t)=4×10−6cos(6πx)cos(24000πt)
Step 4: Finding the Strain
Strain ϵ is defined as the change in length per unit length, which can be obtained by differentiating the displacement y(x,t) with respect to x:
ϵ=∂y∂x
Calculating the derivative:
ϵ=−4×10−6⋅6πsin(6πx)cos(24000πt)
Thus, the strain is:
ϵ=−24π×10−6sin(6πx)cos(24000πt)
Step 5: Finding the Stress
Stress σ is given by:
σ=Y⋅ϵ
Substituting the values:
σ=6.4×1010⋅(−24π×10−6sin(6πx)cos(24000πt))
Calculating the stress:
σ=−1.536×105πsin(6πx)cos(24000πt)
Final Equation
Thus, the equation describing the stress developed in the rod is:
σ=−1.536×105πsin(6πx)cos(24000πt)
We have a metallic rod of length L=1m with one end free and the other end clamped. There are 6 antinodes in the rod, and the amplitude of the antinode is given as A=4×10−6m. The Young's modulus Y is 6.4×1010N/m2 and the density ρ is 4×103kg/m3.
The equation for the stationary wave can be expressed as:
y(x,t)=Acos(kx)cos(ωt)
where k is the wave number and ω is the angular frequency.
k=nπL=6π1=6πrad/m
Thus, the equation becomes:
y(x,t)=4×10−6cos(6πx)cos(ωt)
To find ω, we can use the relationship between the speed of sound v, Young's modulus Y, and density ρ:
v=√Yρ
Calculating v:
v=√6.4×10104×103=√1.6×107≈4000m/s
Now, using the relationship ω=vk:
ω=4000×6π=24000πrad/s
Thus, the equation of the stationary wave is:
y(x,t)=4×10−6cos(6πx)cos(24000πt)
Strain ϵ is defined as the change in length per unit length, which can be obtained by differentiating the displacement y(x,t) with respect to x:
ϵ=∂y∂x
Calculating the derivative:
ϵ=−4×10−6⋅6πsin(6πx)cos(24000πt)
Thus, the strain is:
ϵ=−24π×10−6sin(6πx)cos(24000πt)
Stress σ is given by:
σ=Y⋅ϵ
Substituting the values:
σ=6.4×1010⋅(−24π×10−6sin(6πx)cos(24000πt))
Calculating the stress:
σ=−1.536×105πsin(6πx)cos(24000πt)
Thus, the equation describing the stress developed in the rod is:
σ=−1.536×105πsin(6πx)cos(24000πt)
Topper's Solved these Questions
Similar Questions
A metallic rod of length 1m has one end free and other end rigidly clamped. Longitudinal stationary waves are set up in the rod in such a way that there are total six antinodes present along the rod. The amplitude of an antinode is 4×10−6m. young's modulus and density of the rod are 6.4×1010N/m2 and 4×103Kg/m3 respectively. Consider the free end to be at origin and at t=0 particles at free end are at positive extreme.
The equation describing displacements of particles about their mean positions is.View SolutionA metallic rod of length 1m has one end free and other end rigidly clamped. Longitudinal stationary waves are set up in the rod in such a way that there are total six antinodes present along the rod. The amplitude of an antinode is 4×10−6m. young's modulus and density of the rod are 6.4×1010N/m2 and 4×103Kg/m3 respectively. Consider the free end to be at origin and at t=0 particles at free end are at positive extreme.
The magnitude of strain at midpoint of the rod at t=1sec isView SolutionA metallic rod of length 1m is rigidly clamped at its mid point. Longirudinal stationary wave are setup in the rod in such a way that there are two nodes on either side of the midpoint. The amplitude of an antinode is 2×10−6m. Write the equation of motion of a point 2 cm from the midpoint and those of the constituent waves in the rod, (Young,s modulus of the material of the rod =2×1011Nm−2 , density =8000kg−m−3). Both ends are free.
View SolutionCalculate the speed of longitudinal wave in steel. Young's modulus for steel is 3×1010N/m2 and its density 1.2×103kg/m3
View SolutionCalculate the speed of longitudinal wave in steel. Young's modulus for steel is 3×1010N/m2 and its density 1.2×103kg/m3
View SolutionA rod of nickel of length l is clamped at its midpoint . The rod is stuck and vibrations are set up in the rod . Find the general expression for the frequency of the longitudinal vibrations of the rod . Young's modulus and density of the rod is Yandρ , respectively.
View SolutionA metallic rod of length 1 m, young's modulus 3×1011Nm−3 and density is 7.5×102kgm3clamped at its middle. Longitudinal stationary vibrations are produced in the rod with the total number of displacement nodes equal to 3. The frequency of vibrations is
View SolutionGiven Y=2×1011N/m2,ρ=104kg/m3
Find out elongation in rod.
 View Solution
View SolutionA metal rod of length 1m is clamped at two points as shown in the figure. Distance of the clamp from the two ends are 5 cm and 15 cm, respectively. Find the minimum and next higher frequency of natural longitudinal oscillation of the rod. Given that Young's modulus of elasticity and density of aluminium are Y=1.6×1011Nm−2 and ρ=2500kgm−3 , respectively.
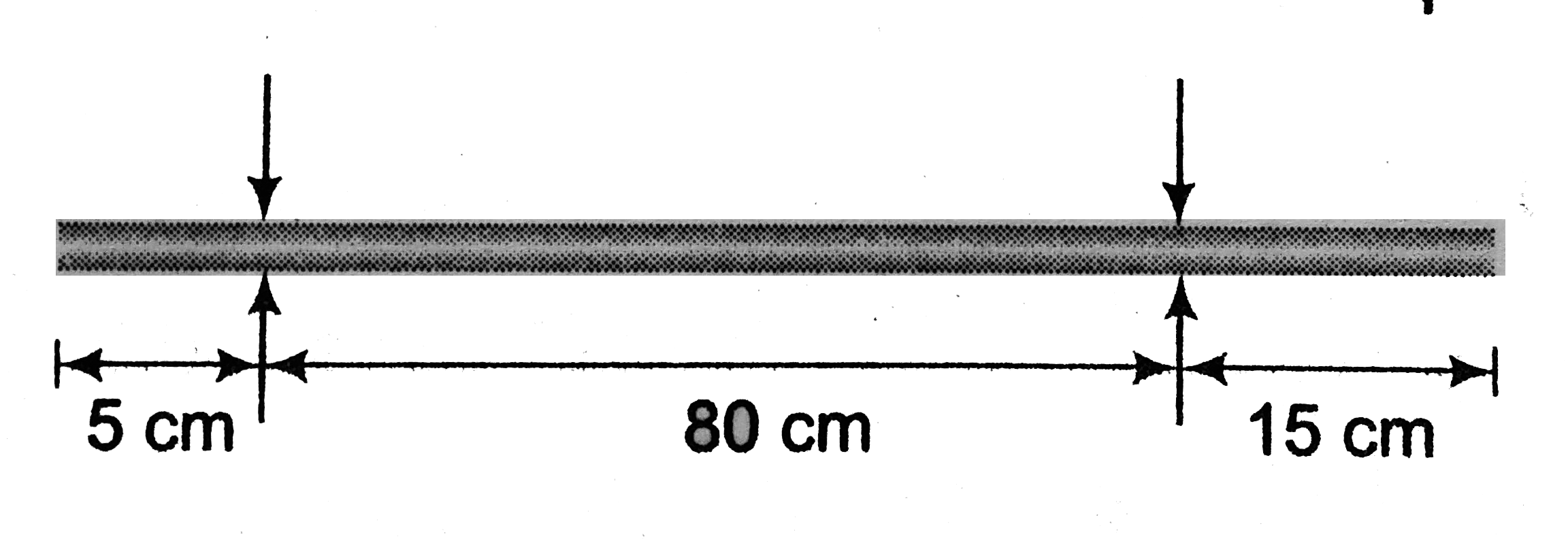 View Solution
View SolutionA rectangular metallic bar one metre long, one cm deep and one cm broad is placed on a smooth table. The Young's modulus and modulus of rigidity of metal of the bar are 2×1011N/m2 and 8×1010N/m2 respectively.
If the bar is rigidity clamped at one end is pulled at the other end with a force 5000 N applied normaly to its end cross-section, calculate the elongation of the bar and the work done in elongating the bar.View Solution
