A detector at x=0 receives waves from three sources each of amplitude A and frequencies f+2,f and f−2.
If I0∝A2, then the value of maximum intensity,is
2I0
3I0
4I0
9I0
The correct Answer is:D
To solve the problem, we need to determine the maximum intensity at the detector located at x=0 when it receives waves from three sources with given amplitudes and frequencies.
1. Identify the Frequencies and Amplitudes:
- The three sources have frequencies f+2, f, and f−2.
- Each source has an amplitude A.
2. Understand the Superposition of Waves:
- When waves from different sources interfere, the resultant amplitude can be calculated using the principle of superposition.
- The intensity I of a wave is proportional to the square of its amplitude: I∝A2.
3. Calculate the Resultant Amplitude:
- The resultant amplitude Ar when three waves interfere can be calculated as follows:
Ar=A1+A2+A3
- Since all sources have the same amplitude A, we have:
Ar=A+A+A=3A
4. Calculate the Maximum Intensity:
- The maximum intensity Imax is given by:
Imax∝(Ar)2=(3A)2=9A2
5. Relate Maximum Intensity to Initial Intensity:
- The initial intensity I0 is proportional to A2:
I0∝A2
- Therefore, we can express Imax in terms of I0:
Imax=9×I0
6. Conclusion:
- The maximum intensity at the detector is 9I0.
Final Answer:
The value of maximum intensity is 9I0.
---
- The three sources have frequencies f+2, f, and f−2.
- Each source has an amplitude A.
- When waves from different sources interfere, the resultant amplitude can be calculated using the principle of superposition.
- The intensity I of a wave is proportional to the square of its amplitude: I∝A2.
- The resultant amplitude Ar when three waves interfere can be calculated as follows:
Ar=A1+A2+A3
- Since all sources have the same amplitude A, we have:
Ar=A+A+A=3A
- The maximum intensity Imax is given by:
Imax∝(Ar)2=(3A)2=9A2
- The initial intensity I0 is proportional to A2:
I0∝A2
- Therefore, we can express Imax in terms of I0:
Imax=9×I0
- The maximum intensity at the detector is 9I0.
The value of maximum intensity is 9I0.
Topper's Solved these Questions
Similar Questions
A detector at x=0 receives waves from three sources each of amplitude A and frequencies f+2,f and f−2.
The time at which intensity is maximum, isView SolutionA detector at x=0 receives waves from three sources each of amplitude A and frequencies f+2,f and f−2.
The equation of waves are : y1=Asin[2π(f+2),y2=Asin2πft and y3=Asin[2π(f−2)t]. The time at which intensity is minimum isView Solution
Knowledge Check
- Question 1 - Select One
Two sounds A and B are of same amplitudes, same wave forms but of frequencies f and 2f respectively. Then :
AB differ in quality from ABB is grave, A is shrillCB is shrill, A is graveDB is louder than A.
Y=2Acos2(kx−ωt) represents a wave with amplitude A´ and frequency f. The value of A´ and f are:
View SolutionIf f''(x)=sec2x and f (0) = f '(0) = 0 then:
View SolutionIf f'(x)=tan−1(Secx+tanx),x∈(−π2,π2) and f(0)=0 then the value of f(1) is
View SolutionIf f(0)=f'(0)=0 and f''(x)=tan2x then f(x) is
View SolutionIf f0 and ff represent the carrier wave frequencies for amplitude and frequency modulations respectively, then
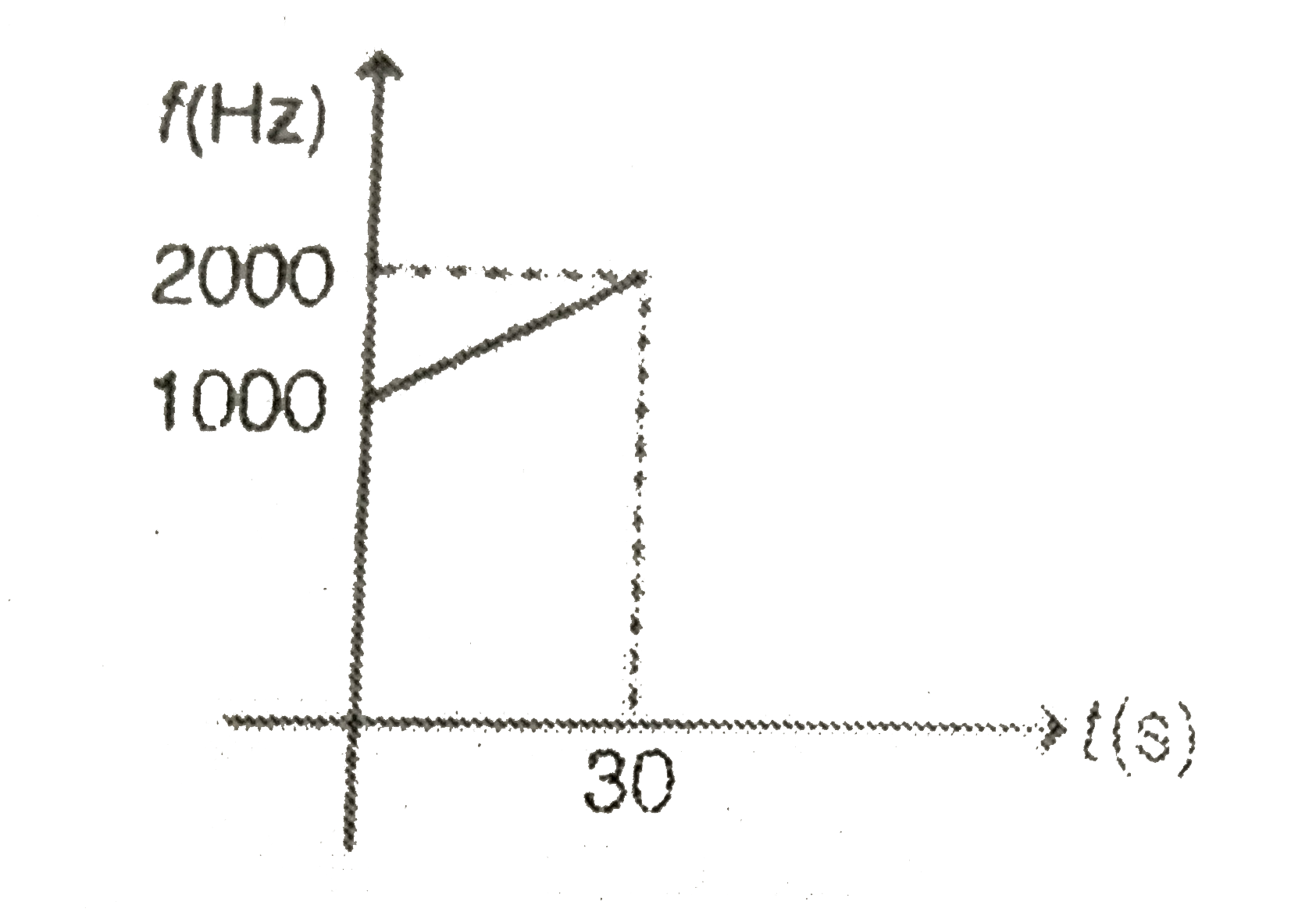
View SolutionA detector is released from rest over a source of sound of frequency f0=103Hz. The frequency observed by the detector at time t is plotted in the graph. The speed of sound in air is (g=10m/s2)
 View Solution
View SolutionTwo radio stations broadcast their programmes at the same amplitude A and at slightly different frequencies ω1 and ω2 respectively, where ω1−ω2=103Hz. A detector receives the signals from the two stations simultaneously, it can only detect signals of intensity ≥2A2.
(i) Find the time interval between successive maxima of the intensity of the signal received by the detector.
(ii) Find the time for which the detector remains idle in each cycle of the intensity of the signal.View Solution

