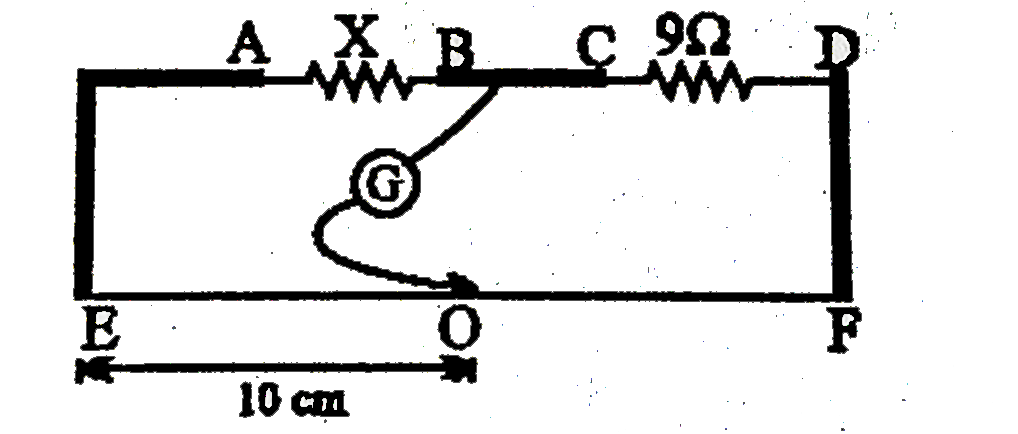
Consider the MB shown in the diagram, let the resistance X have temperature coefficient $α_{1}$ and the resistance from the RB have the temperature coefficient $α_{2}$ . Let the reading of the meter scale be 10 cm from the LHS. if the temperature of the two resistance increase by small temperature $Δ T$ then what is the shift in the position of the null point ? Neglect all the other changes in the bridge due to temperature rise.

A

$9 (α_{1} - α_{2}) Δ T$

B

$9 (α_{1} + α_{2}) Δ T$

C

$1 / 9 (α_{1} + α_{2}) Δ T$

D

$1 / 9 (α_{1} - α_{2}) Δ T$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

$\frac{X}{10} = \frac{90}{90} \Rightarrow X = 10 Ω$
$\Rightarrow \frac{10 (1 + α_{1} Δ T)}{10 + Δ l} = \frac{90 (1 + α_{2} Δ T)}{90 - Δ l}$
$\Rightarrow (1 + α_{1} Δ T) {(1 + \frac{Δ l}{10})}^{- 1} = (1 + α_{2} Δ T) (1 - \frac{Δ l}{90})$
$\Rightarrow (1 + α_{1} Δ T) (1 - \frac{Δ T}{10}) = (1 + α_{2} Δ T) (1 + \frac{Δ l}{90})$
$\Rightarrow 1 + α_{1} Δ T - \frac{Δ l}{10} = 1 + α_{2} Δ T + \frac{Δ l}{90}$
$\Rightarrow (α_{1} - α_{2}) Δ T = \frac{Δ l}{9} \Rightarrow Δ l = 9 (α_{1} - α_{2}) Δ T$

Updated on:21/07/2023

|

Class 11 PHYSICS DIMENSION AND MEASUREMENT

Topper's Solved these Questions

Similar Questions

ALLEN-ERROR AND MEASUREMENT-Part-2(Exercise-1)

Home
Ask Doubt
Profile