Two indentical balls A and B moving with velocities uA and uB In the same direction collide. Coefficient of restitution is e.
(a) Deduce expression for velocities of the balls after the collision.
(b) If collision is perfectly elastic, what do you observe?
To solve the problem, we will break it down into two parts as stated in the question.
Part (a): Deduce expression for velocities of the balls after the collision.
1. Identify the Initial Conditions:
- Let the initial velocities of balls A and B be uA and uB, respectively.
- Let the final velocities after the collision be vA and vB.
2. Conservation of Linear Momentum:
- The total initial momentum before the collision is given by:
Pinitial=mAuA+mBuB
- After the collision, the total momentum is:
Pfinal=mAvA+mBvB
- Since the masses of the balls are identical, we can denote them as m. Thus, we have:
muA+muB=mvA+mvB
- Dividing through by m gives:
uA+uB=vA+vB(Equation 1)
3. Coefficient of Restitution:
- The coefficient of restitution e is defined as:
e=vB−vAuA−uB
- Rearranging this gives:
e(uA−uB)=vB−vA(Equation 2)
4. Solving the Equations:
- We have two equations (Equation 1 and Equation 2). We can solve these simultaneously.
- From Equation 1, we can express vB in terms of vA:
vB=uA+uB−vA
- Substitute this into Equation 2:
e(uA−uB)=(uA+uB−vA)−vA
- Simplifying gives:
e(uA−uB)=uA+uB−2vA
- Rearranging for vA:
2vA=uA+uB−e(uA−uB)
vA=(1−e)uA+(1+e)uB2
5. Finding vB:
- Substitute vA back into Equation 1:
vB=uA+uB−vA
- This gives:
vB=uA+uB−(1−e)uA+(1+e)uB2
- Simplifying yields:
vB=(1+e)uA+(1−e)uB2
Thus, the final expressions for the velocities after the collision are:
vA=(1−e)uA+(1+e)uB2
vB=(1+e)uA+(1−e)uB2
Part (b): If the collision is perfectly elastic, what do you observe?
1. Condition for Perfectly Elastic Collision:
- In a perfectly elastic collision, the coefficient of restitution e=1.
2. Substituting e=1:
- Substitute e=1 into the expressions derived for vA and vB:
vA=(1−1)uA+(1+1)uB2=0+2uB2=uB
vB=(1+1)uA+(1−1)uB2=2uA+02=uA
3. Conclusion:
- After a perfectly elastic collision, the two identical balls exchange their velocities:
vA=uBandvB=uA
Summary of Results:
- The velocities after collision are:
vA=(1−e)uA+(1+e)uB2,vB=(1+e)uA+(1−e)uB2
- In case of a perfectly elastic collision (e=1):
vA=uB,vB=uA
- Let the initial velocities of balls A and B be uA and uB, respectively.
- Let the final velocities after the collision be vA and vB.
- The total initial momentum before the collision is given by:
Pinitial=mAuA+mBuB
- After the collision, the total momentum is:
Pfinal=mAvA+mBvB
- Since the masses of the balls are identical, we can denote them as m. Thus, we have:
muA+muB=mvA+mvB
- Dividing through by m gives:
uA+uB=vA+vB(Equation 1)
- The coefficient of restitution e is defined as:
e=vB−vAuA−uB
- Rearranging this gives:
e(uA−uB)=vB−vA(Equation 2)
- We have two equations (Equation 1 and Equation 2). We can solve these simultaneously.
- From Equation 1, we can express vB in terms of vA:
vB=uA+uB−vA
- Substitute this into Equation 2:
e(uA−uB)=(uA+uB−vA)−vA
- Simplifying gives:
e(uA−uB)=uA+uB−2vA
- Rearranging for vA:
2vA=uA+uB−e(uA−uB)
vA=(1−e)uA+(1+e)uB2
- Substitute vA back into Equation 1:
vB=uA+uB−vA
- This gives:
vB=uA+uB−(1−e)uA+(1+e)uB2
- Simplifying yields:
vB=(1+e)uA+(1−e)uB2
vA=(1−e)uA+(1+e)uB2
vB=(1+e)uA+(1−e)uB2
- In a perfectly elastic collision, the coefficient of restitution e=1.
- Substitute e=1 into the expressions derived for vA and vB:
vA=(1−1)uA+(1+1)uB2=0+2uB2=uB
vB=(1+1)uA+(1−1)uB2=2uA+02=uA
- After a perfectly elastic collision, the two identical balls exchange their velocities:
vA=uBandvB=uA
- The velocities after collision are:
vA=(1−e)uA+(1+e)uB2,vB=(1+e)uA+(1−e)uB2
- In case of a perfectly elastic collision (e=1):
vA=uB,vB=uA
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
A ball collides with a frictionless wall with velocity u as shown in the figure. Coefficient of restitution for the impact is e.
(a) Find expression for the velocity of the ball immediately after the impact.
(b) If impact is perfectly elastic what do you observe?
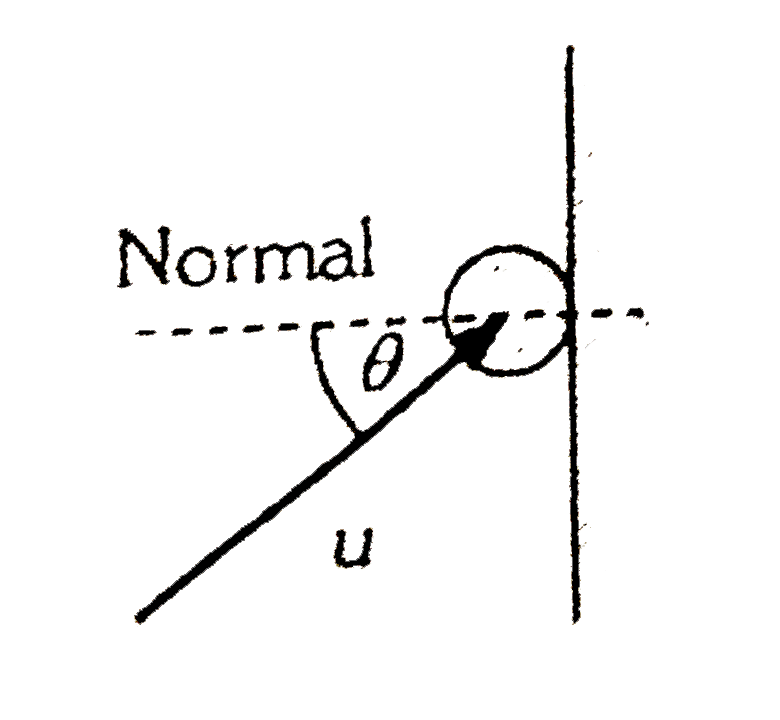
View SolutionA ball P is moving with a certain velocity v, collides head-on with another ball Q of same mass at rest. The coefficient of restitution is 1/4, then ratio of velocity of P and Q just after the collision is
View Solution
Knowledge Check
A ball of mass m moving with a speed 2v0 collides head-on with an identical ball at rest. If e is the coefficient of restitution, then what will be the ratio of velocity of two balls after collision?
A1−e1+eBe−1e+1C1+e1−eDe+1e−1A ball of mass m moving with a speed 2v0 collides head-on with an identical ball at rest. If e is the coefficient of restitution, then what will be the ratio of velocity of two balls after collision?
A1−e1+eB1+e1−eCe−1e+1De+1e−1
The first ball of mass m moving with the velocity υ collides head on with the second ball of mass m at rest. If the coefficient of restitution is e, then the ratio of the velocities of the first and the second ball after the collision is
View SolutionA ball moving with velocity 2ms−1 collides head on with another stationary ball of double the mass. If the coefficient of restitution is 0.5, then their velocities (in ms−1) after collision will be
View SolutionA ball of mass 2kg moving with speed 5m/s collides directly with another ball of mass 3kg moving in the same direction with speed 4m/s. The coefficient of restitution is 2/3. Find the velocities of both ball after the collision.
View SolutionA ball of mass m moving with velocity u collides head-on which the second ball of mass m at rest. If the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View SolutionA ball of mass m moving with velocity v collides head-on which the second ball of mass m at rest. I the coefficient of restitution is e and velocity of first ball after collision is v1 and velocity of second ball after collision is v2 then
View SolutionA glass ball collides with an identical ball at rest with v0=2 m/sec. If the coefficient of restitution of collision is e = 0.5, find the velocities of the glass balls just after the collision.
View Solution
