An man is riding on a cart travelling at a speed of on a frictionless horizontal plane jumps off the cart, such that, his velocity just after is zero with respect to ground. The work done by him on th esystem during his jump is given as (Ain integer). Find the value of A.
The correct Answer is:3
To solve the problem, we will follow these steps:
Step 1: Understand the system
We have a man with a mass of m1=80kg and a cart with a mass of m2=40kg. The initial speed of the system (man + cart) is u=2.5m/s.
Step 2: Apply the conservation of momentum
Before the man jumps off, the total momentum of the system is given by:
Initial Momentum=(m1+m2)⋅u=(80+40)⋅2.5=120⋅2.5=300kg m/s
After the man jumps off, his velocity with respect to the ground is 0m/s. Let the velocity of the cart after the man jumps off be v.
The momentum after the jump is:
Final Momentum=m1⋅0+m2⋅v=0+40v=40v
By conservation of momentum:
Initial Momentum=Final Momentum
300=40v
Solving for v:
v=30040=7.5m/s
Step 3: Calculate the change in kinetic energy
The change in kinetic energy (work done by the man on the system) can be calculated using the formula:
ΔKE=KEfinal−KEinitial
Initial Kinetic Energy:
KEinitial=12(m1+m2)u2=12⋅120⋅(2.5)2=12⋅120⋅6.25=375J
Final Kinetic Energy:
KEfinal=12m2v2=12⋅40⋅(7.5)2=12⋅40⋅56.25=1125J
Step 4: Calculate the change in kinetic energy
ΔKE=KEfinal−KEinitial=1125−375=750J
Step 5: Convert Joules to Kilojoules
Since 1KJ=1000J:
ΔKE=750J=0.75KJ
Step 6: Find the value of A
According to the problem, the work done by the man is given as A4KJ:
A4=0.75⟹A=0.75×4=3
Thus, the value of A is 3.
Final Answer
3
We have a man with a mass of m1=80kg and a cart with a mass of m2=40kg. The initial speed of the system (man + cart) is u=2.5m/s.
Before the man jumps off, the total momentum of the system is given by:
Initial Momentum=(m1+m2)⋅u=(80+40)⋅2.5=120⋅2.5=300kg m/s
Final Momentum=m1⋅0+m2⋅v=0+40v=40v
Initial Momentum=Final Momentum
300=40v
Solving for v:
v=30040=7.5m/s
The change in kinetic energy (work done by the man on the system) can be calculated using the formula:
ΔKE=KEfinal−KEinitial
KEinitial=12(m1+m2)u2=12⋅120⋅(2.5)2=12⋅120⋅6.25=375J
KEfinal=12m2v2=12⋅40⋅(7.5)2=12⋅40⋅56.25=1125J
ΔKE=KEfinal−KEinitial=1125−375=750J
Since 1KJ=1000J:
ΔKE=750J=0.75KJ
According to the problem, the work done by the man is given as A4KJ:
A4=0.75⟹A=0.75×4=3
3
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
A man of mass 80 kg is riding on a small cart of mass 40 kg which is rolling along a level floor at a speed 2 m/s . He is running on the cart , so that his velocity relative to the cart is 3 m/s in the direction opposite to the motion of cart . What is the speed of the centre of the mass of the system ?
View SolutionA girl of mass 40kg jumps with a horizontal velocity of 5m/s onto a stationary cart with frictionless wheels. The mass of the cart is 3kg. What is her velocity as the cart starts moving? Assume that there is no external unbalanced force working in the horizontal direction.
View Solution
Knowledge Check
A man weighing 60 kg is standing on a trolley weighing 240 kg. The trolley is resting on a frictionless horizontal rails. If the man starts walking on the trolley along the rails at speed 1m/s, then after 4 seconds, his displacement relative to the ground will be
A6 mB4.8 mC3.2 mD2.4 m
A monkey of mass 20 kg rides on a 40 kg trolley moving at a constant speed of 8 m/s along a horizontal track. Frictional force can be neglected. If the monkey jumps vertically, with respect to the moving trolley, the catch the overhanging branch of tree, the speed of the trolley after the monkey has jumped off is :-
View SolutionA monkey of mass 20 kg rides on a 40 kg trolley moving at a constant speed of 8 m/s along a horizontal track. Frictional force can be neglected. If the monkey jumps vertically, with respect to the moving trolley, the catch the overhanging branch of tree, the speed of the trolley after the monkey has jumped off is :-
View SolutionA man moves on a straight horizontal road with a block of mass 2 kg in his hand. If he covers a distance of 340 m with an acceleration of 0.5ms2 find the work done by the man on the block during the motion.
View SolutionA man weighing 80kg is standing on a trolley weighting 320kg. The trolley is resting on frictionless horizontal rails. If the man starts walking on the trolley along the rails at speed 1m/s (w.r.t. to trolley) then after 4s his displacement relative to the ground will be :
View SolutionA man of mass 50kg is runing on a plank of mass 150kg with speed of 8m/s relative to plank as shows in the figure (both were initially at rest and velocity of man with respect to ground any how remains constant). Plank is placed on smooth horizontal surface. The man, while runing, whistle with frequency fo. A detected (D) placed on plank detects frequency. The man jumps off with same velocity (w.r.t. to groung) from point D and slides on the smooth horizontal surface [Assume coefficient of friction between man and horizontal is zero]. The speed of sound in still medium is 330m/s. Answer the following questions on the basis of above situations.
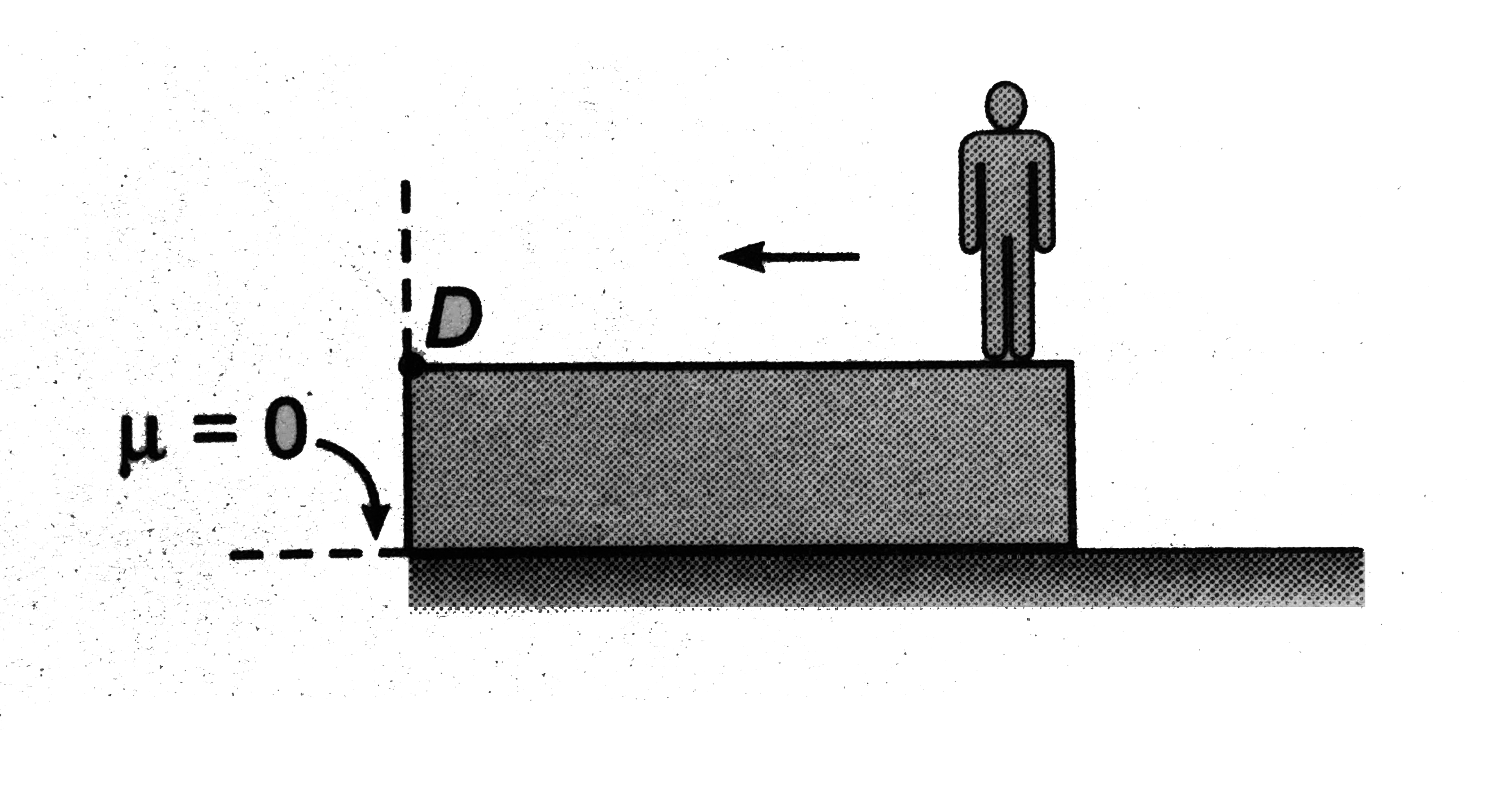
The frequency of sound detected by D, after man jumps off the plank is
View SolutionA man of mass 50kg is runing on a plank of mass 150kg with speed of 8m/s relative to plank as shows in the figure (both were initially at rest and velocity of man with respect to ground any how remains constant). Plank is placed on smooth horizontal surface. The man, while running, whistle with frequency fo. A detected (D) placed on plank detects frequency. The man jumps off with same velocity (w.r.t. to ground) from point D and slides on the smooth horizontal surface [Assume coefficient of friction between man and horizontal is zero]. The speed of sound in still medium is 330m/s. Answer the following questions on the basis of above situations.
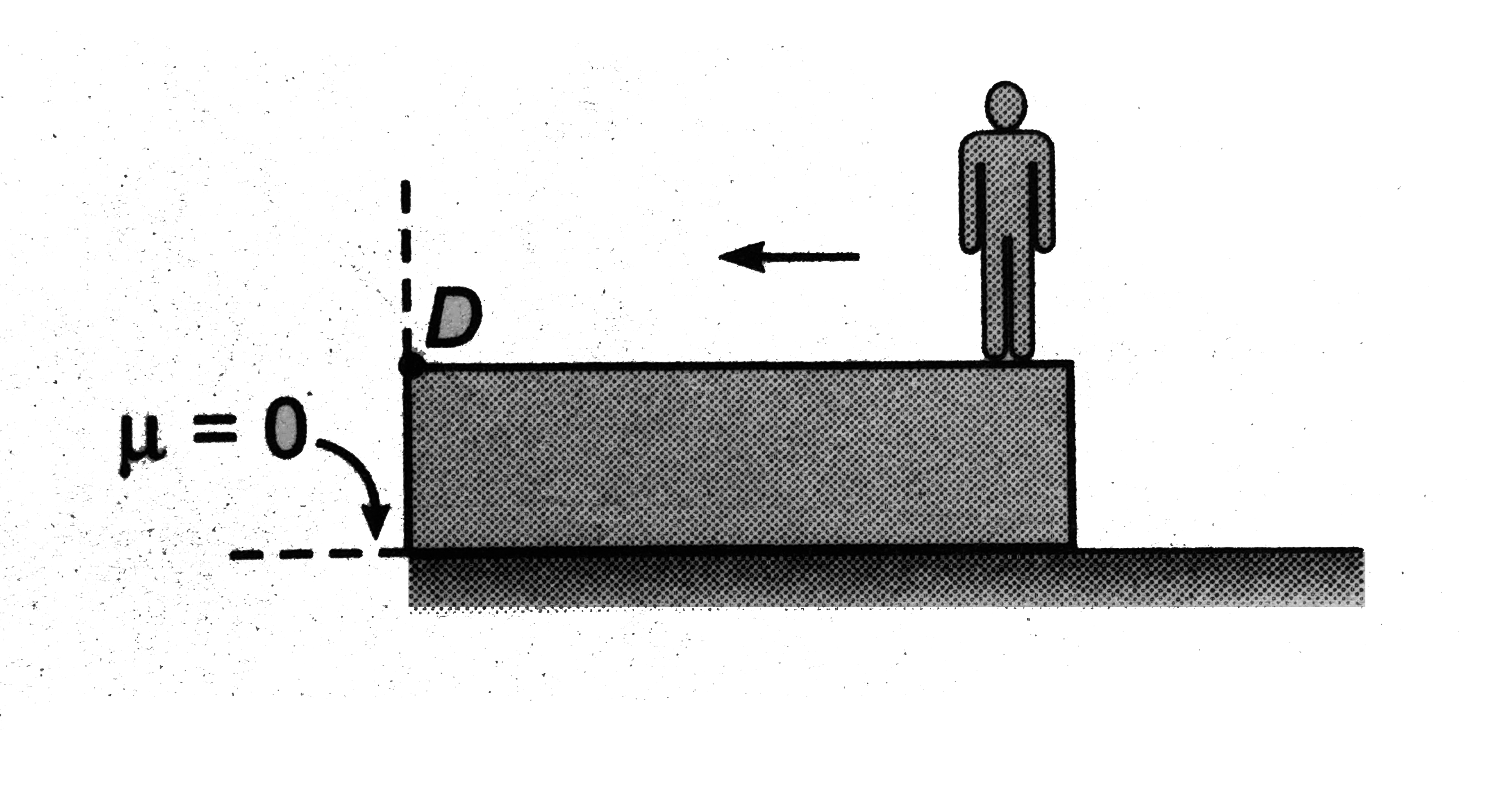
The frequency of sound detected by detector D, before man jumps of the plank is
View SolutionA man of mass 50kg is runing on a plank of mass 150kg with speed of 8m/s relative to plank as shows in the figure (both were initially at rest and velocity of man with respect to ground any how remains constant). Plank is placed on smooth horizontal surface. The man, while runing, whistle with frequency fo. A detected (D) placed on plank detects frequency. The man jumps off with same velocity (w.r.t. to groung) from point D and slides on the smooth horizontal surface [Assume coefficient of friction between man and horizontal is zero]. The speed of sound in still medium is 330m/s. Answer the following questions on the basis of above situations.
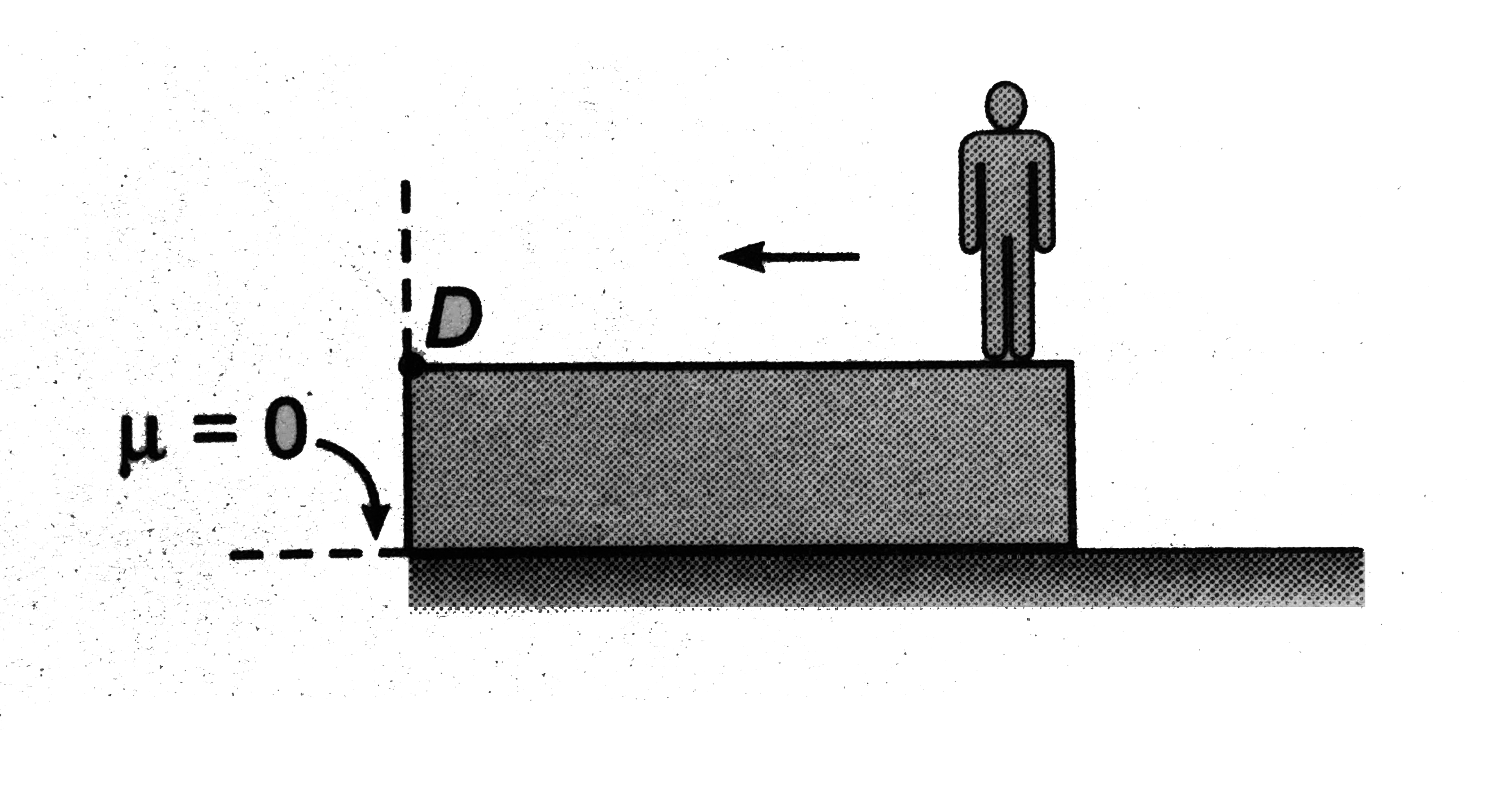
The frequency of sound detected by detector D, before man jumps of the plank is
View Solution
