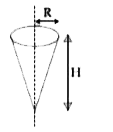
Distance of the centre of mass of a solid uniform cone from its vertex is $Z_{0}$ . If the radius of its base is R and its height is h then $Z_{0}$ is equal to :

A

$\frac{5 h}{80}$

B

$\frac{3 h^{2}}{8 R}$

C

$\frac{h^{2}}{4 R}$

D

$\frac{3 h}{4}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

for solid cone c.m. is $\frac{h}{4}$ from base
so $z_{0} = h - \frac{h}{4} = \frac{3 h}{4}$

|

Updated on:21/07/2023

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19videos
CENTRE OF MASS
BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-IV B
14videos
CENTRE OF MASS
BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11videos
BASIC MATHEMATICS USED IN PHYSICS &VECTORS
BOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS

Similar Questions

Knowledge Check

Question 1 - Select One
The centre of gravity of a hollow cone of height h is at distance x from its vertex where the value of x is:
A $h / 3$
B $h / 4$
C $2 h / 3$
D $3 h / 4$

ALLEN-CENTRE OF MASS-EXERCISE-V A

Home
Ask Doubt
Profile