Shown in the figure is a hollow ice-cream cone (it is open at the top). If its mass is M, radius of its top , R and height , H, then its moment of inertia its axis is :
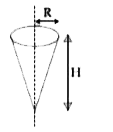
MR22
M(R2+H2)4
MH23
MR23
Step by step video & image solution for Shown in the figure is a hollow ice-cream cone (it is open at the top). If its mass is M, radius of its top , R and height , H, then its moment of inertia its axis is : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
On meltng of ice on the pole of the earth, its moment of inertia will :-
View SolutionThe radius of base of a cone is r and height is h. Find its volume.
View SolutionThe moment of inertia of a ring about its geometrical axis is I, then its moment of inertia about its diameter will be
View SolutionDistance of the centre of mass of a solid uniform cone from its vertex is z0. It the radius of its base is R and its height is h then z0 is equal to:
View SolutionFind the total surface area of a cone, if its slant height is 9m and the radius of its base is 12m.
View SolutionA wheel comprises a ring of radius R and mass M and three spokes of mass m each. The moment of inertia of the wheel about its axis is

View SolutionMoment of inertia of a cylindrical shell of mass M, radius R and length L about its geometrical axis would be -
View SolutionIf a disc of moment of inertia I and radius r is reshaped into a ring of radius nr, keeping its mass same, its moment of inertia becomes
View SolutionA cylindrical tank of height H is open at the top end and it has a radius r. Water is filled in it up to a height of h. The time taken to empty the tank through a hole of radius r' in its bottom is
View SolutionA solid sphere of mass M, radius R and having moment of inertia about as axis passing through the centre of mass as I, is recast into a disc of thickness t, whose moment of inertia about an axis passing through its edge and perpendicular to its plance remains I. Then, radius of the disc will be.
View Solution
