A sports car accelerations from zero to a certain speed in t seconds. How long does it take for it to accelerate to twice that speed starting from rest, assuming the power of the engine to be constant (independent of velocity) and neglecting any resistance to motion?
√2tsec
2tsec
3tsec
4tsec
The correct Answer is:D
To solve the problem, we need to analyze the relationship between power, time, and speed for the sports car under constant power conditions.
1. Understanding the Problem:
- The sports car accelerates from rest (initial speed = 0) to a speed v in time t.
- We need to find the time t′ it takes to accelerate to a speed of 2v starting from rest.
2. Using the Power Formula:
- The power P is defined as the rate of doing work. The work done on the car to accelerate it to speed v is given by the kinetic energy formula:
Work=12mv2
- The power can also be expressed in terms of time:
P=Workt=12mv2t
- Rearranging gives us:
P⋅t=12mv2(1)
3. Calculating Work for Speed 2v:
- Now, we need to find the work done to accelerate the car to speed 2v:
Work=12m(2v)2=12m⋅4v2=2mv2
- The power in terms of the new time t′ is:
P⋅t′=2mv2(2)
4. Relating the Two Equations:
- From equation (1), we have:
P⋅t=12mv2
- From equation (2), we can express 2mv2 in terms of P⋅t:
P⋅t′=2mv2=4(12mv2)=4P⋅t
- Thus, we can equate:
P⋅t′=4P⋅t
5. Solving for t′:
- Dividing both sides by P (assuming P≠0):
t′=4t
6. Conclusion:
- Therefore, the time taken to accelerate to twice the speed 2v starting from rest is:
t′=4t
Final Answer:
The time taken to accelerate to twice the speed is 4t.
- The sports car accelerates from rest (initial speed = 0) to a speed v in time t.
- We need to find the time t′ it takes to accelerate to a speed of 2v starting from rest.
- The power P is defined as the rate of doing work. The work done on the car to accelerate it to speed v is given by the kinetic energy formula:
Work=12mv2
- The power can also be expressed in terms of time:
P=Workt=12mv2t
- Rearranging gives us:
P⋅t=12mv2(1)
- Now, we need to find the work done to accelerate the car to speed 2v:
Work=12m(2v)2=12m⋅4v2=2mv2
- The power in terms of the new time t′ is:
P⋅t′=2mv2(2)
- From equation (1), we have:
P⋅t=12mv2
- From equation (2), we can express 2mv2 in terms of P⋅t:
P⋅t′=2mv2=4(12mv2)=4P⋅t
- Thus, we can equate:
P⋅t′=4P⋅t
- Dividing both sides by P (assuming P≠0):
t′=4t
- Therefore, the time taken to accelerate to twice the speed 2v starting from rest is:
t′=4t
The time taken to accelerate to twice the speed is 4t.
Topper's Solved these Questions
Similar Questions
A car of mass 1000kg accelerates uniformly from rest to a velocity of 54km/h in 5 seconds. Calculate (i) its acceleration (ii) its gain in KE (iii) average power of the engine during this period.
View SolutionA graph of acceleration versus time of a particle starting from rest at t=0 is as shown in figure. The speed of the particle at t=14s is
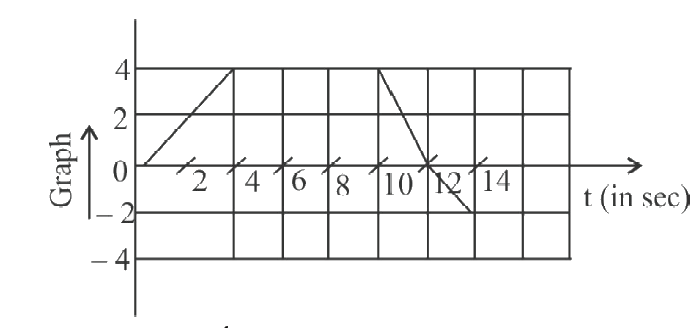 View Solution
View SolutionA particle starting from rest moves along a straight line with constant acceleration for this velocity displacement graph will have the form-
View SolutionA car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute the maximum velocity aquired by the car is
View SolutionAn automobile of mass m accelerates from rest. If the engine supplies a constant power P, the velocity at time t is given by -
View SolutionA body starting from rest gains an angular speed of 540 r.p.m in 6 second. The angular acceleration of the body is
View SolutionEngine of car of mass m supplies a constant power P. Starting from rest at an instant of time, then
View SolutionA car of mass m starta from rest and accelerates so that the instyantaneous power delivered to the car has a constant magnitude P0. The instaneous velocity of this car is proportional to
View SolutionA car starts from erest accelerates uniform by for 4 second and then moves with uniform velocity which of the x-t graph represent th motion of the car:-
View SolutionA particle of mass M starting from rest undergoes uniform acceleration. If the speed acquired in time T is V, the power delivered to the particle is -
View Solution

