A ball A moving with a velocity 5 m/s collides elastically with another identical ball at rest such that the velocity of A makes an angle of 30∘ with the line joining the centres of the balls. Then consider following statements :
(A) Balls A an B move at right angles after collision
(B) kinetic energy is conserved
(C) speed of A after collision is (5/2) m/s
(D) speed of B after collision is (5√3/2) m/s
Select correct alternative :-
Only (A) and (B)
Only (A), (B) and (C)
Only (A), (B) and (D)
All are correct
The correct Answer is:A
To solve the problem, we will analyze the elastic collision between the two identical balls, A and B.
Step 1: Understand the Initial Conditions
- Ball A is moving with a velocity of 5m/s at an angle of 30∘ to the line joining the centers of the balls.
- Ball B is initially at rest.
Step 2: Break Down the Velocity of Ball A
We can resolve the velocity of Ball A into two components:
- Along the line of impact (x-direction):
VAx=VAcos(30∘)=5⋅√32=5√32m/s
- Perpendicular to the line of impact (y-direction):
VAy=VAsin(30∘)=5⋅12=52m/s
Step 3: Analyze the Collision
In an elastic collision between identical balls:
- The components of the velocities along the line of impact are exchanged.
- The perpendicular components remain unchanged.
Step 4: Determine the Final Velocities
- After the collision:
- The velocity of Ball A along the line of impact becomes 0 (it transfers its momentum to Ball B).
- The velocity of Ball B along the line of impact becomes VAx:
VBx=VAx=5√32m/s
- The perpendicular component of Ball A remains the same:
VAy=52m/s
- The perpendicular component of Ball B remains 0.
Step 5: Calculate the Final Speeds
- The speed of Ball A after the collision is:
VA=√V2Ax+V2Ay=√02+(52)2=52m/s
- The speed of Ball B after the collision is:
VB=√V2Bx+02=5√32m/s
Summary of Results
- Speed of A after collision: 52m/s
- Speed of B after collision: 5√32m/s
Step 6: Evaluate the Statements
- (A) Balls A and B move at right angles after collision: True (since one moves along the line of impact and the other perpendicular).
- (B) Kinetic energy is conserved: True (in elastic collisions, kinetic energy is conserved).
- (C) Speed of A after collision is 52m/s: True.
- (D) Speed of B after collision is 5√32m/s: True.
Conclusion
All statements (A), (B), (C), and (D) are correct.
- Ball A is moving with a velocity of 5m/s at an angle of 30∘ to the line joining the centers of the balls.
- Ball B is initially at rest.
We can resolve the velocity of Ball A into two components:
- Along the line of impact (x-direction):
VAx=VAcos(30∘)=5⋅√32=5√32m/s
- Perpendicular to the line of impact (y-direction):
VAy=VAsin(30∘)=5⋅12=52m/s
In an elastic collision between identical balls:
- The components of the velocities along the line of impact are exchanged.
- The perpendicular components remain unchanged.
- After the collision:
- The velocity of Ball A along the line of impact becomes 0 (it transfers its momentum to Ball B).
- The velocity of Ball B along the line of impact becomes VAx:
VBx=VAx=5√32m/s
- The perpendicular component of Ball A remains the same:
VAy=52m/s
- The perpendicular component of Ball B remains 0.
- The speed of Ball A after the collision is:
VA=√V2Ax+V2Ay=√02+(52)2=52m/s
- The speed of Ball B after the collision is:
VB=√V2Bx+02=5√32m/s
- Speed of A after collision: 52m/s
- Speed of B after collision: 5√32m/s
- (A) Balls A and B move at right angles after collision: True (since one moves along the line of impact and the other perpendicular).
- (B) Kinetic energy is conserved: True (in elastic collisions, kinetic energy is conserved).
- (C) Speed of A after collision is 52m/s: True.
- (D) Speed of B after collision is 5√32m/s: True.
All statements (A), (B), (C), and (D) are correct.
Topper's Solved these Questions
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLENCHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
A ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionA ball A collides elasticlly with an another identical bass B with velocity 10 m//s at an angle of 30∘ from the line joining their centres C1 and C2 Select the correct alternative (s).
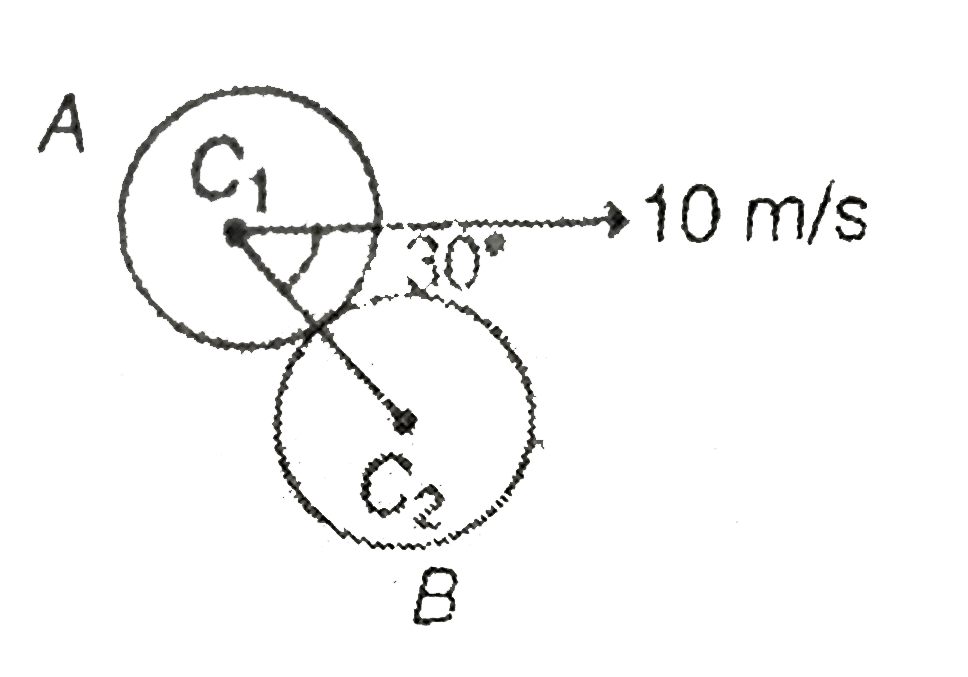
View Solution
Knowledge Check
A ball of mass m moving with a speed 2v0 collides head-on with an identical ball at rest. If e is the coefficient of restitution, then what will be the ratio of velocity of two balls after collision?
A1−e1+eBe−1e+1C1+e1−eDe+1e−1A ball of mass m moving with a speed 2v0 collides head-on with an identical ball at rest. If e is the coefficient of restitution, then what will be the ratio of velocity of two balls after collision?
A1−e1+eB1+e1−eCe−1e+1De+1e−1
A ball moving with a speed of 9ms−1 strikes an identical stationary ball such that after the collision the direction of each ball makes an angle of 30∘ with the original line of motion. The speeds of two balls after the collision is
View SolutionA heavy ball of mass 2M moving with a velocity v0 collides elastically head on with a cradle of three identical ball each of mass M as shown in figure. Determine the velocity of each ball after collision.

View SolutionA body of mass 'm' moving with certain velocity collides with another identical body at rest. If the collision is perfectly elastic and after the collision both the bodies moves
View SolutionA ball A of mass M collides elastically with a similar ball B at rest as shown in figure. Initially velocity of ball A is u m/s. After collision,

View SolutionTwo identical balls each moving with speed v at right angle to each other collide perfectly inelastically. Their speed after collision is
View SolutionA smooth ball 'A' moving with velocity 'V' collides with another smooth identical ball at rest. After collision both the balls move with same speed with angle between their velocities 60∘. No external force acts on the system of balls.

The speed of each ball after the collision is
View Solution
