A driver travelling at speed 36kmh−1 sees the light trun red at the intersection. If his reaction time is 0.6s, and then the car can deaccelerate at 4ms−2. Find the stopping distance of the car.
The correct Answer is:18.5 m
To find the stopping distance of the car, we will break down the problem into several steps:
Step 1: Convert the speed from km/h to m/s
The speed of the car is given as 36km/h. We need to convert this speed into meters per second (m/s).
Speed in m/s=36×518=10m/s
Step 2: Calculate the distance traveled during the reaction time
The driver's reaction time is given as 0.6s. During this time, the car continues to travel at its initial speed of 10m/s.
Distance during reaction time=Speed×Time=10m/s×0.6s=6m
Step 3: Determine the deceleration phase
After the reaction time, the car begins to decelerate at 4m/s2. The initial velocity at this point is still 10m/s, and the final velocity when the car stops is 0m/s.
Step 4: Use the kinematic equation to find the stopping distance
We will use the kinematic equation:
v2=u2+2as
Where:
- v is the final velocity (0 m/s),
- u is the initial velocity (10 m/s),
- a is the acceleration (-4 m/s², since it is deceleration),
- s is the distance we want to find.
Rearranging the equation to solve for s:
0=(10)2+2×(−4)×s
This simplifies to:
0=100−8s
Solving for s:
8s=100⟹s=1008=12.5m
Step 5: Calculate the total stopping distance
The total stopping distance is the sum of the distance traveled during the reaction time and the distance traveled while decelerating.
Total stopping distance=Distance during reaction time+Distance while decelerating
Total stopping distance=6m+12.5m=18.5m
Final Answer
The stopping distance of the car is 18.5m.
---
The speed of the car is given as 36km/h. We need to convert this speed into meters per second (m/s).
The driver's reaction time is given as 0.6s. During this time, the car continues to travel at its initial speed of 10m/s.
After the reaction time, the car begins to decelerate at 4m/s2. The initial velocity at this point is still 10m/s, and the final velocity when the car stops is 0m/s.
We will use the kinematic equation:
- v is the final velocity (0 m/s),
- u is the initial velocity (10 m/s),
- a is the acceleration (-4 m/s², since it is deceleration),
- s is the distance we want to find.
The total stopping distance is the sum of the distance traveled during the reaction time and the distance traveled while decelerating.
Total stopping distance=6m+12.5m=18.5m
The stopping distance of the car is 18.5m.
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-04[B]
14 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]
11 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Comprehension#7
3 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
A heavy ball is suspended from the ceiling of a motor car through a light string. A transverse pulse travels at a speed of 60cms−1 on the string when the car is at rest and 62cms−1 when the car accelerates on a horizontal road. Find the acceleration of the car. Take g=10ms−2
View SolutionA car is moving with a velocity of 10 m/s. Suddenly driver sees a child at a distance of 30 m and he applies the brakes, it produced a uniform retardation in car and car stops in 5 second. Find (mass of car=1000 kg)
the stopping distance of car
will the car hit the child
the retardation in car
resistive force on car
View Solution
Knowledge Check
A car starts from rest, attain a velocity of 36kmh−1 with an acceleration of 0.2ms−2, travels 9 km with this uniform velocity and then comes to halt with a uniform deaceleration of 0.1 ms−2. The total time of travel of the car is
A1050 sB1000 sC950 sD900 s
A driver takes 0.20 s to apply the brakes after he sees a need for it. This is called the reaction time of the driver. If he ils driving a car at a speed of 54 km/h and the brakes cause a deceleration of 6.0ms2, find the distance travelled by the car after he sees the need to put the brakes on.
View SolutionThe driver of a car moving at 30ms(−1) suddenly sees a truck that is moving in the same direction at 10ms−1 and is 60m head. The maximum deceletation of the car is 5ms(−2).
a. Will the collision occur if the driver's reaction time is zero ? If so. then?
b. If the car driver's reaction time of 0.5s included, what is the minimum deceleration required to avoied the collision?.
View SolutionThe driver of a car moving with a speed of 10ms−1 sees a red light ahead, applies brakes and stops after covering 10 m distance. If the same car were moving with a speed of 20ms−1 the same driver would have stopped the car after covering 30 m distance. Within what distance the car can be stopped if traveling with a velocity of 15ms−1 Assume the same reaction time and the same deceleration in each case
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
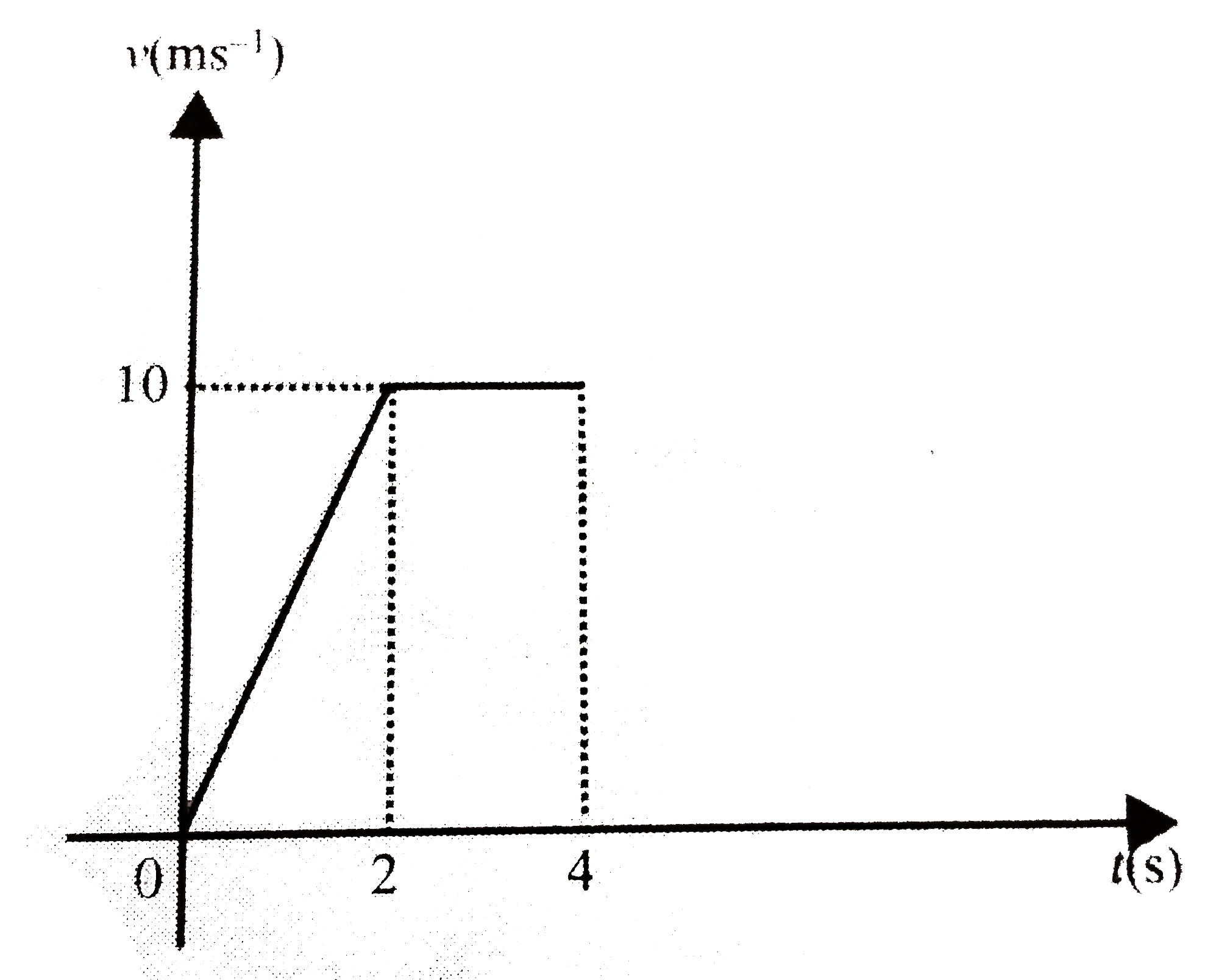
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View SolutionA car 2 m long travelling at 30ms−1 overtakes another car 4 m long travelling at 20ms−1. The time taken by the first car to pass the second car is
View SolutionAt the instant, the traffic light turns green, a car that has been waiting at an intersection starts ahead with a constant acceleration of 3.20ms−2, At the some instant, a truck travelling with a constant speed of 20.0ms−1, overtakes and passes the car.
a. At what distance from its starting point does the car overtake the truck?
b. Calculate the speed of the car when it overtakes the truck.
c. Sketch an x−t graph of themotion of both vehicles.
Take x−0 at the intersection.
d. Sketch a vx−t graph of the motion of both vehicles.
View SolutionThe driver of a three wheeler moving with a speed of 36km/h sees a child standing in the middle of the road and brings his vehicle to rest in 4 s just in time to save the child What is the average retarding force on the vehicle ? The mass of three wheeler is 400 kg and mass of the driver is 65 kg.
View Solution
