A particle is projected from a point O with an initial speed of 30ms−1 to pass through a point which is 40m from O horizontally and 10m above O. There are two angles of projection for which this is possible. These angles are α and β. The value of |tan(α+β)| is

The correct Answer is:4

y=xtanθ−9x22u2cos2θ
10=40tanθ−10×1600(1+tan2θ)2×900
10=40tanθ−809−809tan2θ
89tan2θ−4tanθ+179=0
8tan2θ−36tanθ+17=0
tanα+tanβ=368
tanα+tanβ=178
tan(α+β)=tanα+tanβ1−tanαtanβ
=36/81−178=36/8−9/8=−4
So |tan(α+β)|=|−4|=4
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-04[B]
14 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]
11 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Comprehension#7
3 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
Knowledge Check
A particle is projected from the ground with an initial speed v at an angle θ with horizontal. The average velocity of the particle between its point of projection and highest point of trajectory is [EAM2013]
AucosθBu2√1+cos2θCu2√1+2cos2θDu2√1+3cos2θ
A particle is projected from a point O with velocity u in a direction making an angle α upward with the horizontal. AT P , it is moving at right angles to its initial direction of projection . Its velocity at P is .
View SolutionA particle of mass m is projected from the ground with an initial speed u at an angle α. Find the magnitude of its angular momentum at the highest point of its trajector about the point of projection.
View SolutionA particle of mass m is projected from the ground with an initial speed u at an angle α. Find the magnitude of its angular momentum at the highest point of its trajector about the point of projection.
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
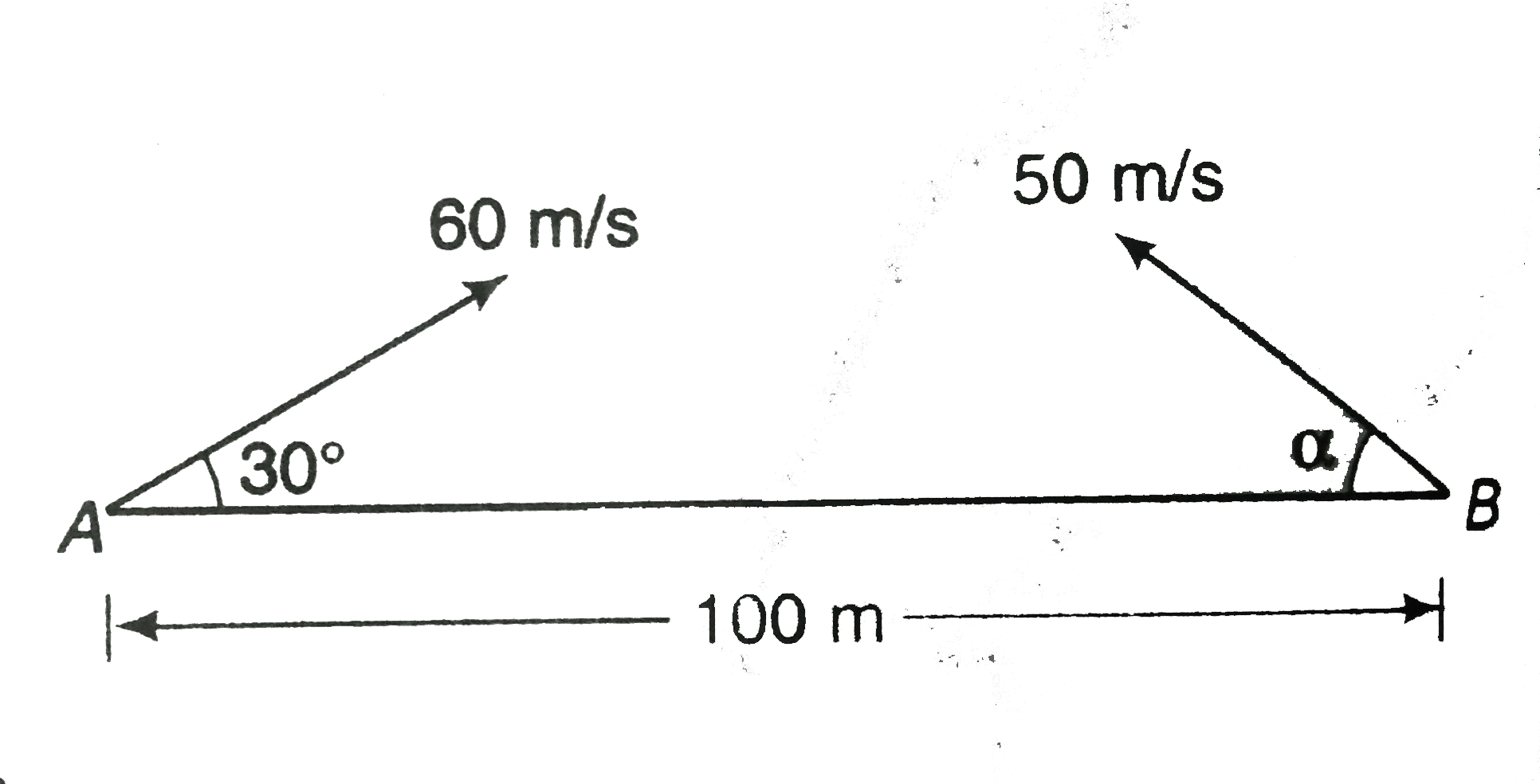
View SolutionA ball is projected form ground with a speed of 20ms−1 at an angle of 45∘ with horizontal.There is a wall of 25m height at a distance of 10m from the projection point. The ball will hit the wall at a height.
View SolutionA body is projected at an angle of 30∘ with the horizontal and with a speed of 30ms−1. What is the angle with the horizontal after 1.5s ? (g=10ms−2).
View SolutionA particle is projected with speed 10m/s at angle 60∘ with the horizontal. Then the time after which its speed becomes half of initial.
View Solution
