When two plane mirrors subtend an angle θ between them, then a ray of light incident parallel to one of them retraces its path afer n reflections such that nθ=c (where c is constant)
If the angle between mirrors is 60∘ . Then light ray retraces its path after n reflections where n is
3
4
2
None of these
The correct Answer is:D
To solve the problem, we need to find the value of n when two plane mirrors subtend an angle of θ=60∘ between them, and the condition for the ray of light to retrace its path is given by nθ=c, where c is a constant.
1. Understanding the Geometry:
- We have two plane mirrors forming an angle θ=60∘.
- A ray of light is incident parallel to one of the mirrors.
2. Finding the Condition for Retracing the Path:
- For the ray to retrace its path after n reflections, the relationship nθ=c must hold true.
- Here, c is a constant that represents the total angular deviation after n reflections.
3. Calculating the Total Angle:
- Since the angle between the mirrors is 60∘, we can express c in terms of n and θ:
c=n⋅60∘
4. Finding the Value of n:
- To find n, we need to determine how many reflections are required for the ray to return to its original path.
- Each reflection changes the direction of the ray by 2θ (since it reflects off both mirrors).
- After n reflections, the total angular deviation will be 2n⋅60∘.
- For the ray to retrace its path, this total deviation must equal 360∘ (a full circle):
2n⋅60∘=360∘
5. Solving for n:
- Rearranging the equation gives:
2n⋅60=360
120n=360
n=360120=3
6. Conclusion:
- The value of n, which is the number of reflections required for the ray to retrace its path, is 3.
Final Answer:
The ray retraces its path after n=3 reflections.
- We have two plane mirrors forming an angle θ=60∘.
- A ray of light is incident parallel to one of the mirrors.
- For the ray to retrace its path after n reflections, the relationship nθ=c must hold true.
- Here, c is a constant that represents the total angular deviation after n reflections.
- Since the angle between the mirrors is 60∘, we can express c in terms of n and θ:
c=n⋅60∘
- To find n, we need to determine how many reflections are required for the ray to return to its original path.
- Each reflection changes the direction of the ray by 2θ (since it reflects off both mirrors).
- After n reflections, the total angular deviation will be 2n⋅60∘.
- For the ray to retrace its path, this total deviation must equal 360∘ (a full circle):
2n⋅60∘=360∘
- Rearranging the equation gives:
2n⋅60=360
120n=360
n=360120=3
- The value of n, which is the number of reflections required for the ray to retrace its path, is 3.
The ray retraces its path after n=3 reflections.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 04 (A)
47 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 04 (B)
15 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - ASSERTION-REASON
36 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
When two plane mirrors subtend an angle θ between them, then a ray of light incident parallel to one of them retraces its path afer n reflections such that nθ=c (where c is constant)
What is the angle of c?
View SolutionWhen two plane mirrors subtend an angle θ between them, then a ray of light incident parallel to one of them retraces its path afer n reflections such that nθ=c (where c is constant)
The graph between θ and n will
View SolutionIn terms of θ find the value of i, show that ray of light retraces its path after third reflection
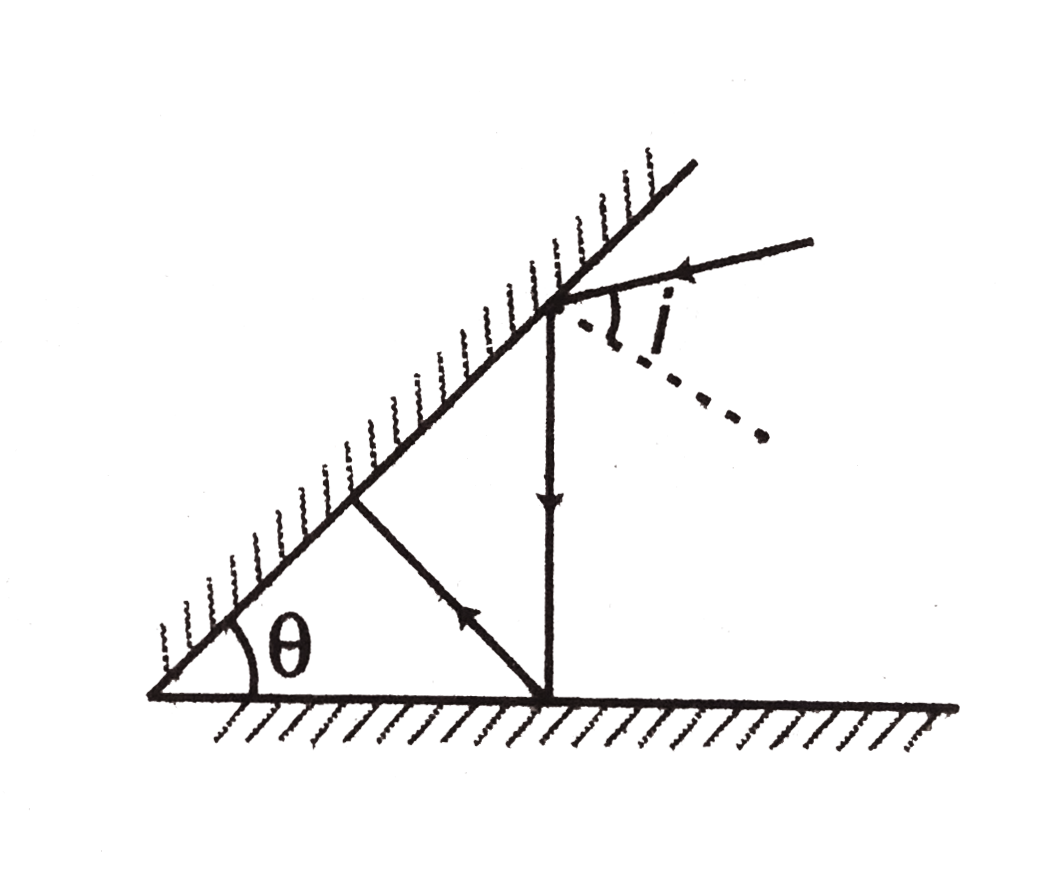
View SolutionTwo mirrors are inclined at angle θ as shown in figure. Light rays are incident parallel to one of mirrors. Light will start retracing its path after the reflection if

View SolutionTwo plans mirrors are inclined to each other at some angle .A ray of light incident at 30∘on one,after reflection form the other retraces its path .The angles between the mirrors is:
View SolutionA mirror is inclined at an angle of θ with the horizontal. If a ray light is incident at an angle θ as shown , then the angle made by reflected ray with the horizontal is
_E01_002_Q01.png)
View SolutionIn the shown figure, mirror is rotated by an angle theta. Find theta if
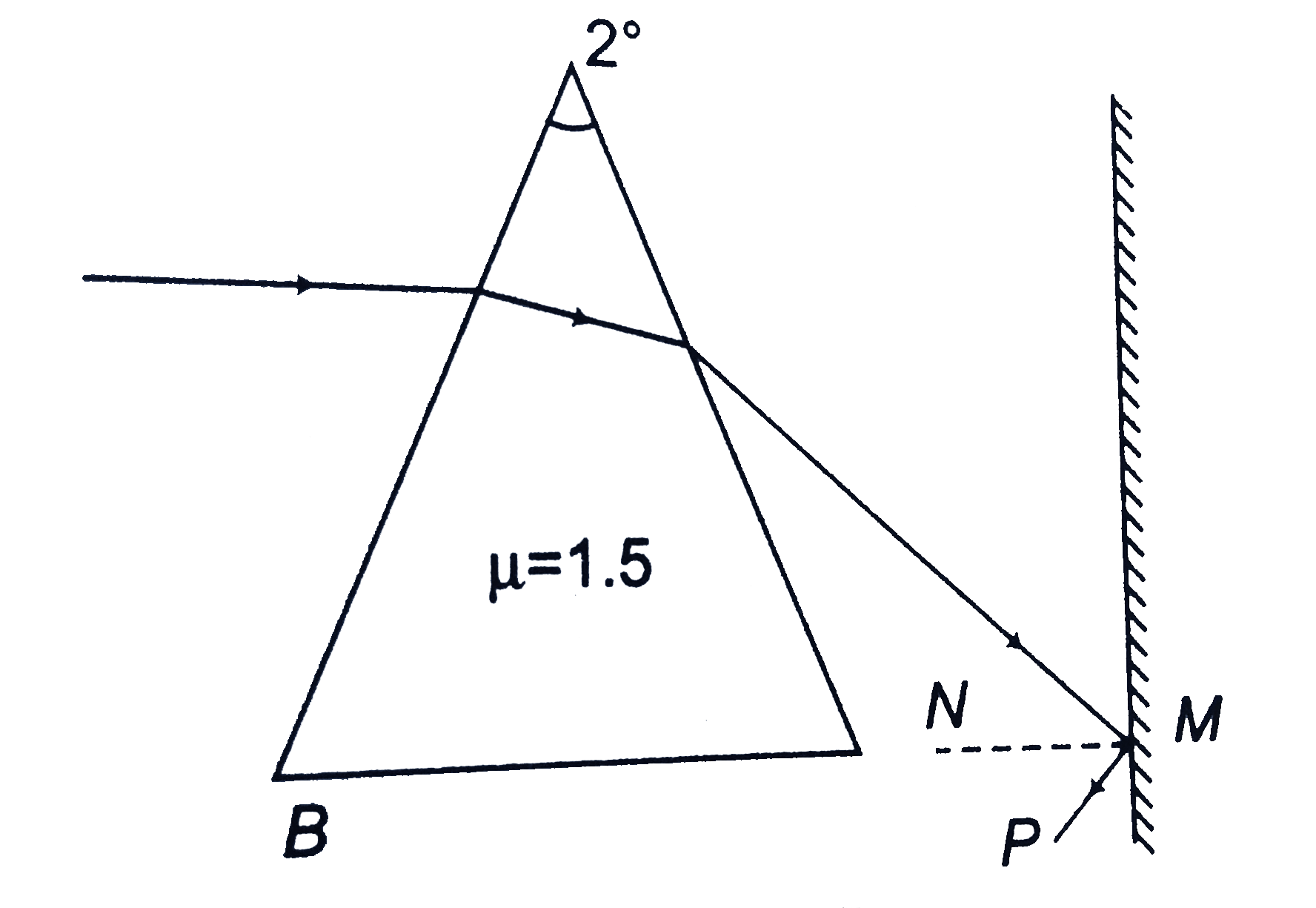
ray of light retraces its path after reflection from M
ray of light MP turns in the direction of MN.
View SolutionTwo plane mirrror are inclined to each other at 70∘. A ray of light is incident on one mirror. They ray after two reflections will undergo a total deviation of :
View SolutionTwo plane mirrors are inclined to each other at an angle 60∘ if a ray of light incident on first mirror parallel to the second mirror, it is reflected from the second mirror
View SolutionTwo plane mirrors are inclined to each other such that a ray of light incident on the first mirror and parallel to the second is reflected from the second mirror parallel to the first mirror. Determine the angle between the two mirrors:
View Solution
