The minimum mass of mixture of A2 and B2 required to produce at least 1 kg of each product is : (Given At. Mass of 'A' = 10 : At. Mass of 'B' = 120 )
2120 gm
1060 gm
560 gm
1660 gm
The correct Answer is:A
To solve the problem of finding the minimum mass of the mixture of A2 and B2 required to produce at least 1 kg of each product AB2 and A2B, we can follow these steps:
Step 1: Determine the Molar Masses
- The molar mass of A2:
- Atomic mass of A = 10 g/mol
- Molar mass of A2=2×10=20 g/mol
- The molar mass of B2:
- Atomic mass of B = 120 g/mol
- Molar mass of B2=2×120=240 g/mol
- The molar mass of AB2:
- Molar mass of AB2=10+(2×120)=250 g/mol
- The molar mass of A2B:
- Molar mass of A2B=(2×10)+120=140 g/mol
Step 2: Calculate Moles Required for Each Product
- For AB2:
- To produce 1 kg (1000 g) of AB2:
Moles of AB2=1000 g250 g/mol=4 moles
- For A2B:
- To produce 1 kg (1000 g) of A2B:
Moles of A2B=1000 g140 g/mol≈7.14 moles
Step 3: Determine Moles of A2 and B2 Required
- From the stoichiometry of the reaction:
- The reaction is 5 mol A2+2 mol B2→2 mol AB2+4 mol A2B.
- For 4 moles of AB2:
- Moles of A2 required = 52×4=10 moles
- Moles of B2 required = 22×4=4 moles
- For 7.14 moles of A2B:
- Moles of A2 required = 54×7.14≈8.925 moles
- Moles of B2 required = 24×7.14≈3.57 moles
Step 4: Determine the Maximum Requirement
- The maximum requirement for A2 is 10 moles (from AB2).
- The maximum requirement for B2 is 4 moles (from AB2).
Step 5: Calculate the Mass of Each Component
- Mass of A2:
Mass of A2=10 moles×20 g/mol=200 g
- Mass of B2:
Mass of B2=4 moles×240 g/mol=960 g
Step 6: Calculate Total Mass of the Mixture
- Total mass of the mixture:
Total mass=200 g+960 g=1160 g
Final Answer
The minimum mass of the mixture of A2 and B2 required to produce at least 1 kg of each product is 1160 g.
- The molar mass of A2:
- Atomic mass of A = 10 g/mol
- Molar mass of A2=2×10=20 g/mol
- Atomic mass of B = 120 g/mol
- Molar mass of B2=2×120=240 g/mol
- Molar mass of AB2=10+(2×120)=250 g/mol
- Molar mass of A2B=(2×10)+120=140 g/mol
- For AB2:
- To produce 1 kg (1000 g) of AB2:
Moles of AB2=1000 g250 g/mol=4 moles
- To produce 1 kg (1000 g) of A2B:
Moles of A2B=1000 g140 g/mol≈7.14 moles
- From the stoichiometry of the reaction:
- The reaction is 5 mol A2+2 mol B2→2 mol AB2+4 mol A2B.
- Moles of A2 required = 52×4=10 moles
- Moles of B2 required = 22×4=4 moles
- Moles of A2 required = 54×7.14≈8.925 moles
- Moles of B2 required = 24×7.14≈3.57 moles
- The maximum requirement for A2 is 10 moles (from AB2).
- The maximum requirement for B2 is 4 moles (from AB2).
- Mass of A2:
Mass of A2=10 moles×20 g/mol=200 g
Mass of B2=4 moles×240 g/mol=960 g
- Total mass of the mixture:
Total mass=200 g+960 g=1160 g
The minimum mass of the mixture of A2 and B2 required to produce at least 1 kg of each product is 1160 g.
Topper's Solved these Questions
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - O-II
11 Videos
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - O-II (Assertion Reason:)
2 Videos
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - S-II
18 Videos
- METALLURGYBOOK - ALLENCHAPTER - METALLURGYEXERCISE - EXERCISE-05 [B]
26 Videos
- p-Block Element BOOK - ALLENCHAPTER - p-Block Element EXERCISE - All Questions
20 Videos
Similar Questions
Calculate the mass of limestone required to produce 112 kg of quicklime by burning it.
View SolutionHow much force is required to produce an acceleration of 2ms−2 in a body of mass 0.8 kg ?
View SolutionP4S3+8O2→P4O10+3SO2
Calculate mass of P4S3 is required at least 9.6 gm of each product.
View SolutionWhat is the mass of oxygen that is required for the complete combustion of 2.8 kg ethylene :-
View SolutionA chemist wishes to provide his consumers, at least cost, the minimum daily requirements of two vitamins A, B by using a mixture of two products M and N. The amount of each vitamin in one gram of each product, cost per gram of each product and the minimum daily requirement are given below:
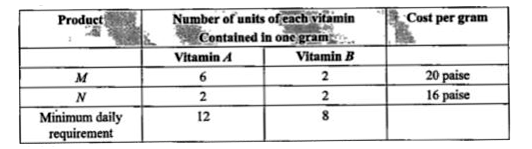
Find the least expensive combination which provides the minimum requirement of the two vitamins.
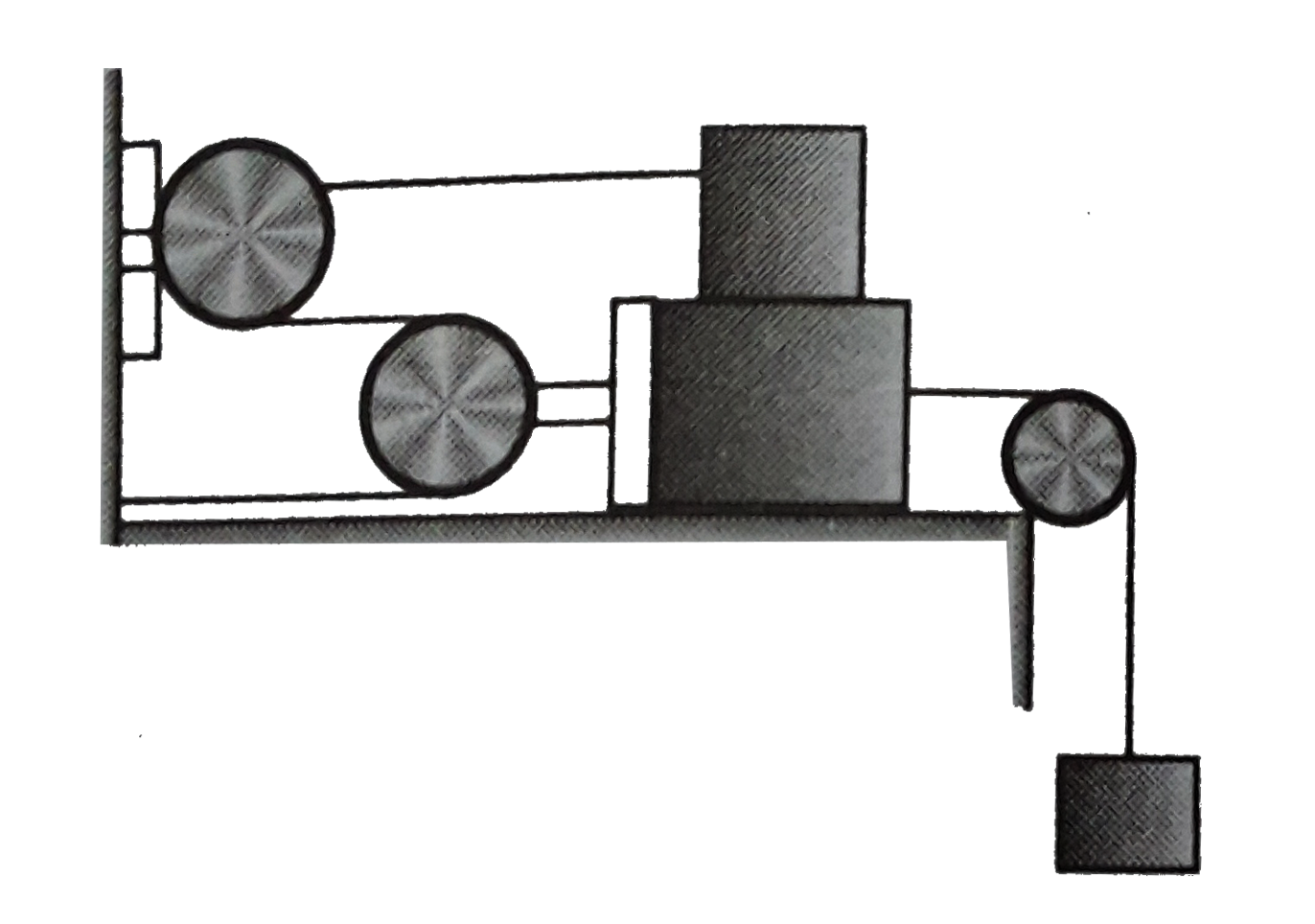
View SolutionThe maximum value of mass of block C so that neither A nor B moves is (given that mass of A is 100kg and the of B 140kg. Pulleys are smooth and friction coefficient between A and B and horizontal surface μ=0.3)(Takingg=10ms−2)
View SolutionThe atomic mass of .7N15 is 15.000108amu and that of .8O16 is 15.994915amu. The minimum energy required to remove the least tightly bound proton is ( mass of proton is 1.007825amu)
View SolutionCalculate the mass of urea (NH2CONH2) required in making 2.5 kg of 0.25 molar aqueous solution.
We know that molarity (m) =Moles of soluteMass of solvent in kg
and moles of soute =Mass of soluteMolar mass of solute
So, find the molar mass of solute by adding atomic masses of different element present in it and mass by using the formula,
Molality =Mass of solute/molar mass of soluteMass of solvent in kg
View SolutionThe centre of mass of three particles of masses 1 kg, 2 kg and 3 kg is at (2,2, 2). The position of the fourth mass of 4 kg to be placed in the system as that the new centre of mass is at (0, 0, 0) is
View SolutionRatio of ΔTb/Kbof6%AB2and9%A2B(AB2andA2B both are non-electrolytes ) is 1 mol/kg in both cases. Hence atomic masses of A and B are respetively.
View Solution
