The position vector of a particle is given by →r = →r0 (1-at)t, where t is the time and a as well as →r0 are constantwhat will be the velocity of the particle when it returns to the starting point?
→r0
−→r0
2→r0
−2→r0
The correct Answer is:B
To solve the problem step by step, we will analyze the given position vector of the particle and determine its velocity when it returns to the starting point.
Step 1: Write down the position vector
The position vector of the particle is given by:
→r(t)=→r0(1−at)t
where →r0 is a constant vector, a is a constant, and t is the time.
Step 2: Find the time when the particle returns to the starting point
To find when the particle returns to the starting point, we set the position vector equal to zero:
→r(t)=0
This gives us:
→r0(1−at)t=0
Since →r0 is not zero, we can focus on the scalar part:
(1−at)t=0
This equation holds true if either t=0 or 1−at=0. Solving for t:
1. t=0 (the initial position)
2. 1−at=0 leads to at=1 or t=1a
Thus, the particle returns to the starting point at t=0 and again at t=1a.
Step 3: Differentiate the position vector to find the velocity vector
Next, we differentiate the position vector with respect to time t to find the velocity vector:
→v(t)=d→rdt
Substituting the expression for →r(t):
→v(t)=ddt(→r0(1−at)t)
Using the product rule:
→v(t)=→r0(ddt(1−at)⋅t+(1−at)⋅ddt(t))
Calculating the derivatives:
ddt(1−at)=−aandddt(t)=1
Thus, we have:
→v(t)=→r0(−at+(1−at)⋅1)
Simplifying this:
→v(t)=→r0(1−2at)
Step 4: Evaluate the velocity at t=1a
Now, we substitute t=1a into the velocity equation:
→v(1a)=→r0(1−2a⋅1a)
This simplifies to:
→v(1a)=→r0(1−2)=−→r0
Final Answer
Thus, the velocity of the particle when it returns to the starting point is:
→v=−→r0
The position vector of the particle is given by:
→r(t)=→r0(1−at)t
where →r0 is a constant vector, a is a constant, and t is the time.
To find when the particle returns to the starting point, we set the position vector equal to zero:
→r(t)=0
This gives us:
→r0(1−at)t=0
Since →r0 is not zero, we can focus on the scalar part:
(1−at)t=0
This equation holds true if either t=0 or 1−at=0. Solving for t:
1. t=0 (the initial position)
2. 1−at=0 leads to at=1 or t=1a
Next, we differentiate the position vector with respect to time t to find the velocity vector:
→v(t)=d→rdt
Substituting the expression for →r(t):
→v(t)=ddt(→r0(1−at)t)
Using the product rule:
→v(t)=→r0(ddt(1−at)⋅t+(1−at)⋅ddt(t))
Calculating the derivatives:
ddt(1−at)=−aandddt(t)=1
Thus, we have:
→v(t)=→r0(−at+(1−at)⋅1)
Simplifying this:
→v(t)=→r0(1−2at)
Now, we substitute t=1a into the velocity equation:
→v(1a)=→r0(1−2a⋅1a)
This simplifies to:
→v(1a)=→r0(1−2)=−→r0
Thus, the velocity of the particle when it returns to the starting point is:
→v=−→r0
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-340videos
MOTION IN A PALNE
- BOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE28videos
MOTION IN A PALNE
- BOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-199videos
MOTION IN A PALNE
- BOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)46videos
KINEMATICS-2D
- BOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)4videos
NEWTON'S LAWS OF MOTION & FRICTION
Similar Questions
The position vector of a particle is given by →r = →r0 (1-at)t, where t is the time and a as well as →r0 are constant. How much distance is covered by the particle in returning to the starting point?
View SolutionThe position vector of a particle is given by →r=→r0 (1-at)t, where t is the time and a as well as →r0 are constant. After what time the particle retursn to the starting point?
View Solution
Knowledge Check
- Question 1 - Select One
The position of a particle is given by →r=3tˆi+2t2ˆj+5ˆk, where t is in seconds and the coefficients have the proper units for →r to be in meters. The direction of velocity of the particle at t=1 s is
A53∘ with x-axisB37∘ with x-axisC30∘ with y-axisD60∘ with y-axis
The position of a particle is given by →r=(8tˆi+3t2ˆj+5ˆk)m where t is measured in second and →r in meter. Calculate, the velocity and the acceleration of the particle.
View SolutionThe position of a particle is given by →r=(8tˆi+3t2ˆj+5ˆk)m where t is measured in second and →r in meter. Calculate, the velocity and the acceleration of the particle.
View SolutionThe position vector of a particle is given by the relation →r=→α(1−γt+βt2), where →α is a constant vector while, β and γ are positive constants. Which of the following statement is true ?
View SolutionThe fig. shows the v−t graph of a particle moving in straight line. Find the time when particle returns to the starting point.
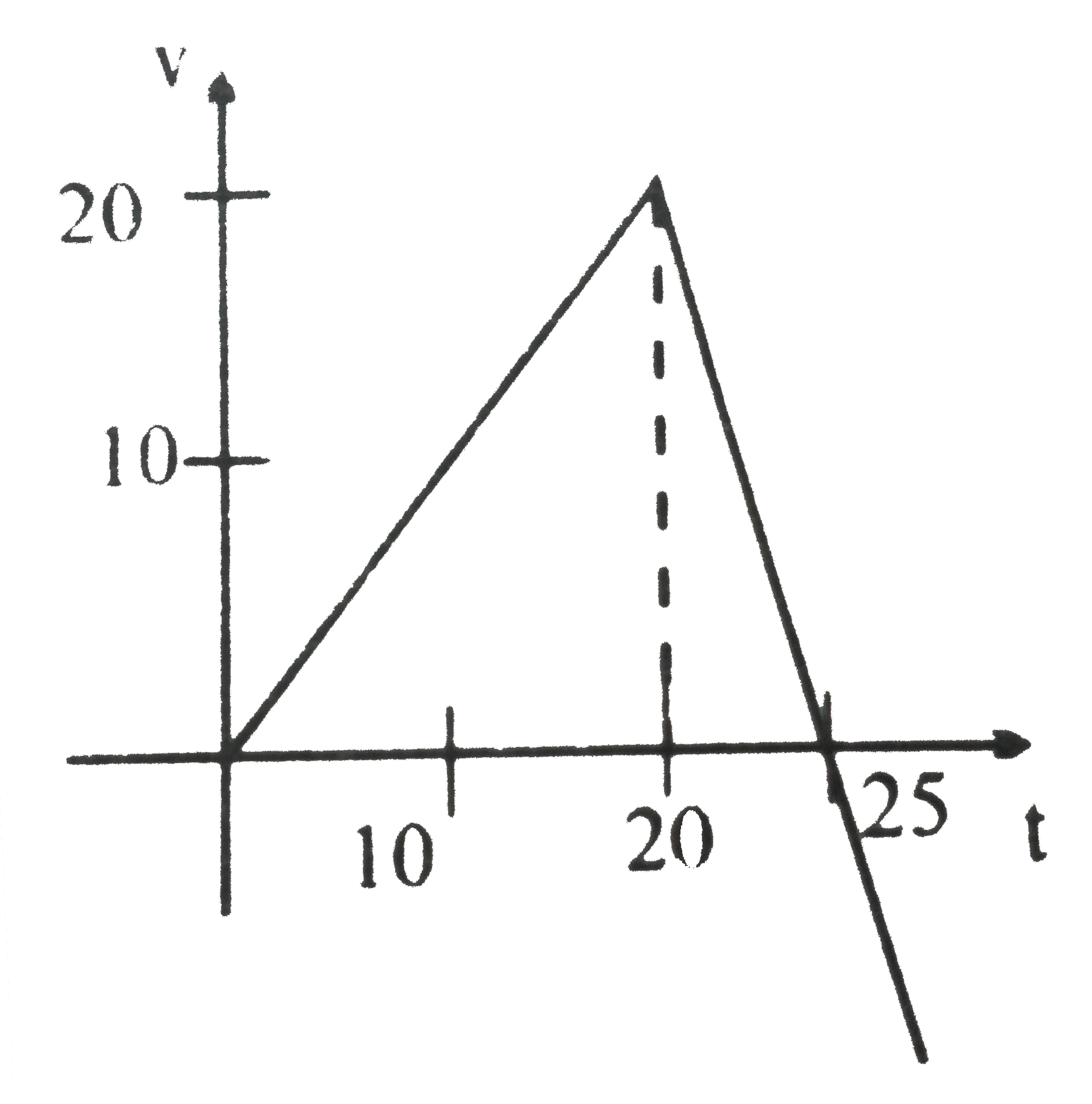 View Solution
View SolutionThe velocity time relation of a particle is given by v =(3t2−2t−1)m/s Calculate the position and acceleration of the particle when velocity of the particle is zero . Given the initial position of the particle is 5m .
View SolutionThe position vector of a aprticle is given as →r=(t2−4t+6)ˆi+(t2)ˆj. The time after which the velocity vector and acceleration vector becomes perpendicular to each other is equal to
View SolutionThe position vector of a aprticle is given as →r=(t2−4t+6)ˆi+(t2)ˆj. The time after which the velocity vector and acceleration vector becomes perpendicular to each other is equal to
View Solution

