The masses and radii of the earth an moon are M1andR1andM2,R2 respectively. Their centres are at a distacne d apart. Find the minimum speed with which the particle of mass m should be projected from a point mid-way between the two centres so as to escape to infinity.
√4G(M1+M2)d
√4GdM1M2(M1+M2)
√2Gd(M1+M2M1M2)
√2Gd(M1+M2)
The correct Answer is:A
To find the minimum speed with which a particle of mass m should be projected from a point midway between the centers of the Earth and the Moon, we can follow these steps:
Step 1: Understand the Setup
We have two bodies: the Earth with mass M1 and radius R1, and the Moon with mass M2 and radius R2. The distance between their centers is d. The midpoint between the two centers is at a distance of d2 from each body.
Step 2: Calculate the Gravitational Potential Energy
At the midpoint, we need to calculate the gravitational potential energy due to both the Earth and the Moon. The gravitational potential V at a distance r from a mass M is given by:
V=−GMr
Thus, the potential energy U of the particle of mass m at the midpoint due to the Earth is:
U1=−GM1md2=−2GM1md
And the potential energy due to the Moon is:
U2=−GM2md2=−2GM2md
Step 3: Total Gravitational Potential Energy
The total gravitational potential energy U at the midpoint is the sum of the potential energies due to both bodies:
U=U1+U2=−2GM1md−2GM2md=−2G(M1+M2)md
Step 4: Set Up the Energy Conservation Equation
To escape to infinity, the particle must have enough kinetic energy to overcome the gravitational potential energy. At infinity, the potential energy is zero, and thus the kinetic energy K at the midpoint must equal the absolute value of the total potential energy:
K=−U
The kinetic energy K is given by:
K=12mv2
Setting the kinetic energy equal to the absolute value of the potential energy, we have:
12mv2=2G(M1+M2)md
Step 5: Solve for the Minimum Speed v
We can cancel m from both sides (assuming m≠0):
12v2=2G(M1+M2)d
Multiplying both sides by 2 gives:
v2=4G(M1+M2)d
Taking the square root of both sides, we find the minimum speed:
v=√4G(M1+M2)d
Final Answer
The minimum speed with which the particle of mass m should be projected from the midpoint to escape to infinity is:
v=√4G(M1+M2)d
---
We have two bodies: the Earth with mass M1 and radius R1, and the Moon with mass M2 and radius R2. The distance between their centers is d. The midpoint between the two centers is at a distance of d2 from each body.
At the midpoint, we need to calculate the gravitational potential energy due to both the Earth and the Moon. The gravitational potential V at a distance r from a mass M is given by:
The total gravitational potential energy U at the midpoint is the sum of the potential energies due to both bodies:
To escape to infinity, the particle must have enough kinetic energy to overcome the gravitational potential energy. At infinity, the potential energy is zero, and thus the kinetic energy K at the midpoint must equal the absolute value of the total potential energy:
We can cancel m from both sides (assuming m≠0):
The minimum speed with which the particle of mass m should be projected from the midpoint to escape to infinity is:
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 2
26 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
Similar Questions
The masses and radii of the Earth and the Moon are M1,R1andM2,R2 respectively. Their centres are at a distance d apart. The minimum speed with which a particle of mass m should be projected from a point midway between the two centres so as to escape to infinity is ........
View Solution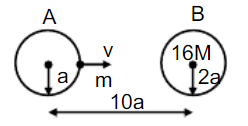
Two planets of mass M and 16M of radius a and 2a respectively, are at distance 10a. Find minimum speed of a particle of mass m at surface of smaller planet so that it can reached from smaller planet to larger planet.
View SolutionTwo concentric spherical shells of masses and radii m1,r1andm2,r2 respectively are as shown. The gravitational field intensity at point P is

View SolutionIf d is the distance between the centre of the earth of mass M1 and the moon of mass M2, then the velocity with which a body should be projected from the mid point of the line joining the earth and the moon, so that it just escape is
View SolutionConsider a system of two particles having masses m1 and m2. If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the mass centre of particles at the original position?
View SolutionConsider a sytem of two particles having masses m1 and m2. If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the mass centre of particles at the original position?
View SolutionTwo particles of masses m1 and m2 (m1>m2) are separated by a distance d. The shift in the centre of mass when the two particles are interchanged.
View SolutionTwo cars of mass m1andm2 are moving in circle of radii r1andr2 , respectively . Their speeds are such that they make complete circles in the same time t . The ratio of their centripetal acceleration is :
View SolutionTwo cars of mass m1andm2 are moving in circle of radii r1andr2 , respectively . Their speeds are such that they make complete circles in the same time t . The ratio of their centripetal acceleration is :
View Solutiona point mass (m) is located at (0,a) where should th 2m mass be kept ,so that centre of mass lies at origin?
View Solution
