When a point mass slips down a smooth incline from top, it reaches the bottom with linear speed v. If same mass in the form of disc rolls down without slipping a rough incline of identical geometry through same distance, what will be its linear velocity at the bottom ?
v√23
√v3
v
√2v3
The correct Answer is:A
To solve the problem of finding the linear velocity of a disc rolling down a rough incline, we will use the principle of conservation of mechanical energy. We will compare the scenario of a point mass sliding down a smooth incline with that of a disc rolling down a rough incline.
1. Understanding the Problem:
- A point mass slides down a smooth incline and reaches the bottom with a linear speed v.
- A disc of the same mass rolls down a rough incline of identical geometry through the same distance.
- We need to find the linear velocity of the disc at the bottom.
2. Energy Conservation for the Point Mass:
- For the point mass, the initial potential energy (PE) at the top is converted entirely into kinetic energy (KE) at the bottom.
- Initial potential energy: PEi=mgh
- Initial kinetic energy: KEi=0 (since it starts from rest)
- Final potential energy at the bottom: PEf=0
- Final kinetic energy: KEf=12mv2
- Applying conservation of energy:
mgh=12mv2
- Canceling m from both sides:
gh=12v2
- Rearranging gives:
v2=2gh⇒v=√2gh
3. Energy Conservation for the Disc:
- For the disc, the initial potential energy is the same:
PEi=mgh
- The disc rolls down, so it has both translational and rotational kinetic energy at the bottom:
- Final potential energy: PEf=0
- Final kinetic energy:
KEf=12mv′2+12Iω2
- For a disc, the moment of inertia I=12mr2 and the relationship between linear velocity v′ and angular velocity ω is v′=rω.
- Substituting ω=v′r into the kinetic energy expression:
KEf=12mv′2+12(12mr2)(v′r)2
- Simplifying gives:
KEf=12mv′2+14mv′2=34mv′2
4. Applying Conservation of Energy for the Disc:
- Setting initial potential energy equal to final kinetic energy:
mgh=34mv′2
- Canceling m from both sides:
gh=34v′2
- Rearranging gives:
v′2=43gh⇒v′=√43gh
5. Relating v′ to v:
- Since we already found v=√2gh:
v′=√43⋅√gh=√43⋅√22⋅√2gh=√86⋅v=v⋅√43
- Therefore, the linear velocity of the disc at the bottom is:
v′=v⋅√23
Final Answer:
The linear velocity of the disc at the bottom is:
v′=v⋅√23
- A point mass slides down a smooth incline and reaches the bottom with a linear speed v.
- A disc of the same mass rolls down a rough incline of identical geometry through the same distance.
- We need to find the linear velocity of the disc at the bottom.
- For the point mass, the initial potential energy (PE) at the top is converted entirely into kinetic energy (KE) at the bottom.
- Initial potential energy: PEi=mgh
- Initial kinetic energy: KEi=0 (since it starts from rest)
- Final potential energy at the bottom: PEf=0
- Final kinetic energy: KEf=12mv2
- Applying conservation of energy:
mgh=12mv2
- Canceling m from both sides:
gh=12v2
- Rearranging gives:
v2=2gh⇒v=√2gh
- For the disc, the initial potential energy is the same:
PEi=mgh
- The disc rolls down, so it has both translational and rotational kinetic energy at the bottom:
- Final potential energy: PEf=0
- Final kinetic energy:
KEf=12mv′2+12Iω2
- For a disc, the moment of inertia I=12mr2 and the relationship between linear velocity v′ and angular velocity ω is v′=rω.
- Substituting ω=v′r into the kinetic energy expression:
KEf=12mv′2+12(12mr2)(v′r)2
- Simplifying gives:
KEf=12mv′2+14mv′2=34mv′2
- Setting initial potential energy equal to final kinetic energy:
mgh=34mv′2
- Canceling m from both sides:
gh=34v′2
- Rearranging gives:
v′2=43gh⇒v′=√43gh
- Since we already found v=√2gh:
v′=√43⋅√gh=√43⋅√22⋅√2gh=√86⋅v=v⋅√43
- Therefore, the linear velocity of the disc at the bottom is:
v′=v⋅√23
The linear velocity of the disc at the bottom is:
v′=v⋅√23
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (GRAVITATION)
25 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Elasticity)
20 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (COLLISION AND CENTRE OF MASS )
12 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
A body of mass m slides down an incline and reaches the bottom with a velocity v. If the same mass were in the form of a ring which rolls down this incline, the velocity of the ring at the bottom would have been
View SolutionA body of mass m slides down an smooth incline and reaches the bottom with a velocity, Now smooth incline surface is made rough and the same mass was in the form of a ring which rolls down this incline, the velocity of the ring at the bottom would have been:
View SolutionA drum of radius R and mass M, rolls down without slipping along an inclined plane of angle θ. The frictional force-
View SolutionA thin circular ring first slips down a smooth incline then rolls down a rough incline of identical geometry from same height. Ratio of time taken in the two motion is :
View SolutionA hollow sphere and a solid sphere having same mass and same radii are rolled down a rough inclined plane.
View SolutionA body is released from the top of a smooth inclined plane of inclination θ. It reaches the bottom with velocity v. If the angle of inclina-tion is doubled for the same length of the plane, what will be the velocity of the body on reach ing the ground .
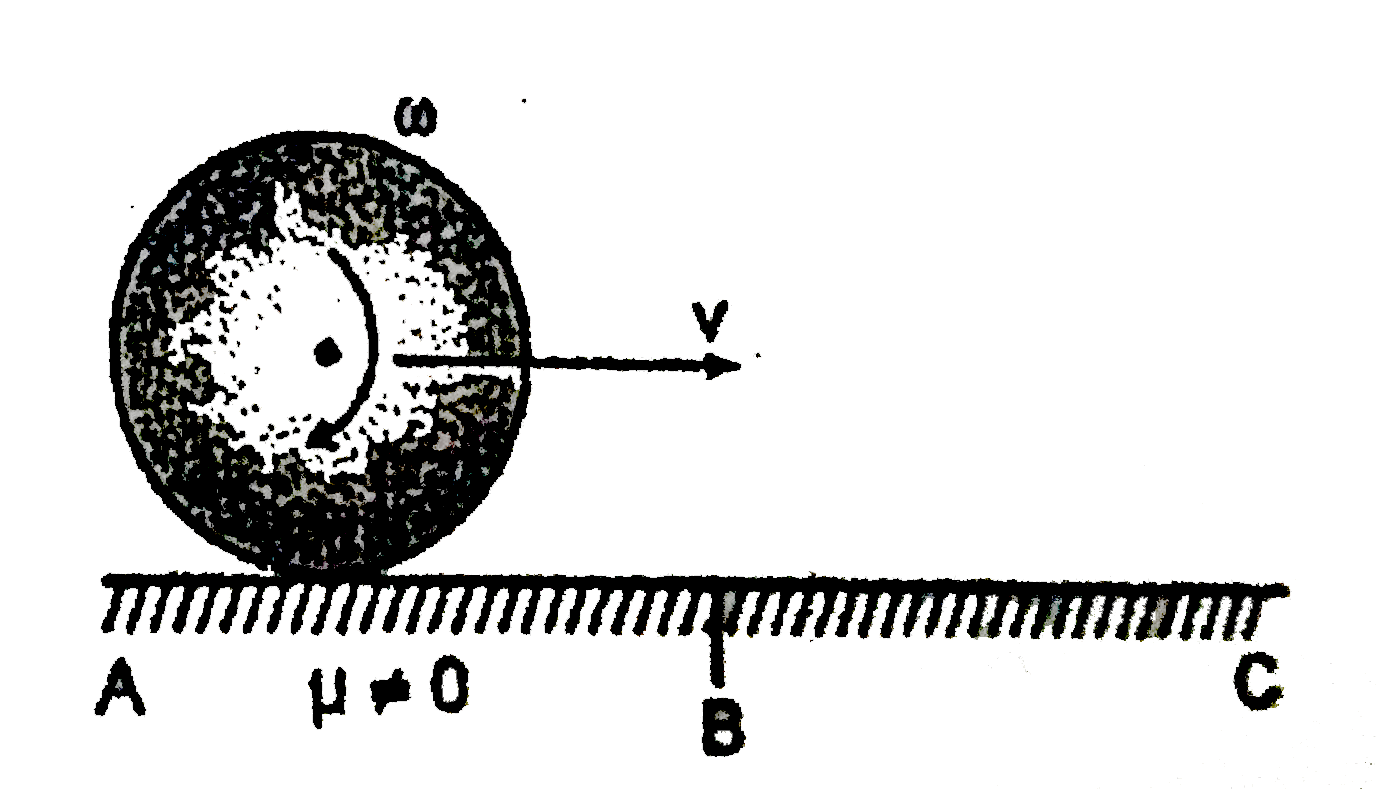
View SolutionAs shown in the figure, a disc of mass m is rolling without slipping with angular velocity ω. When it crosses point B disc will be in
View SolutionA solid cylinder of mass M and radius R rolls without slipping down an inclined plane of length L and height h. What is the speed of its center of mass when the cylinder reaches its bottom
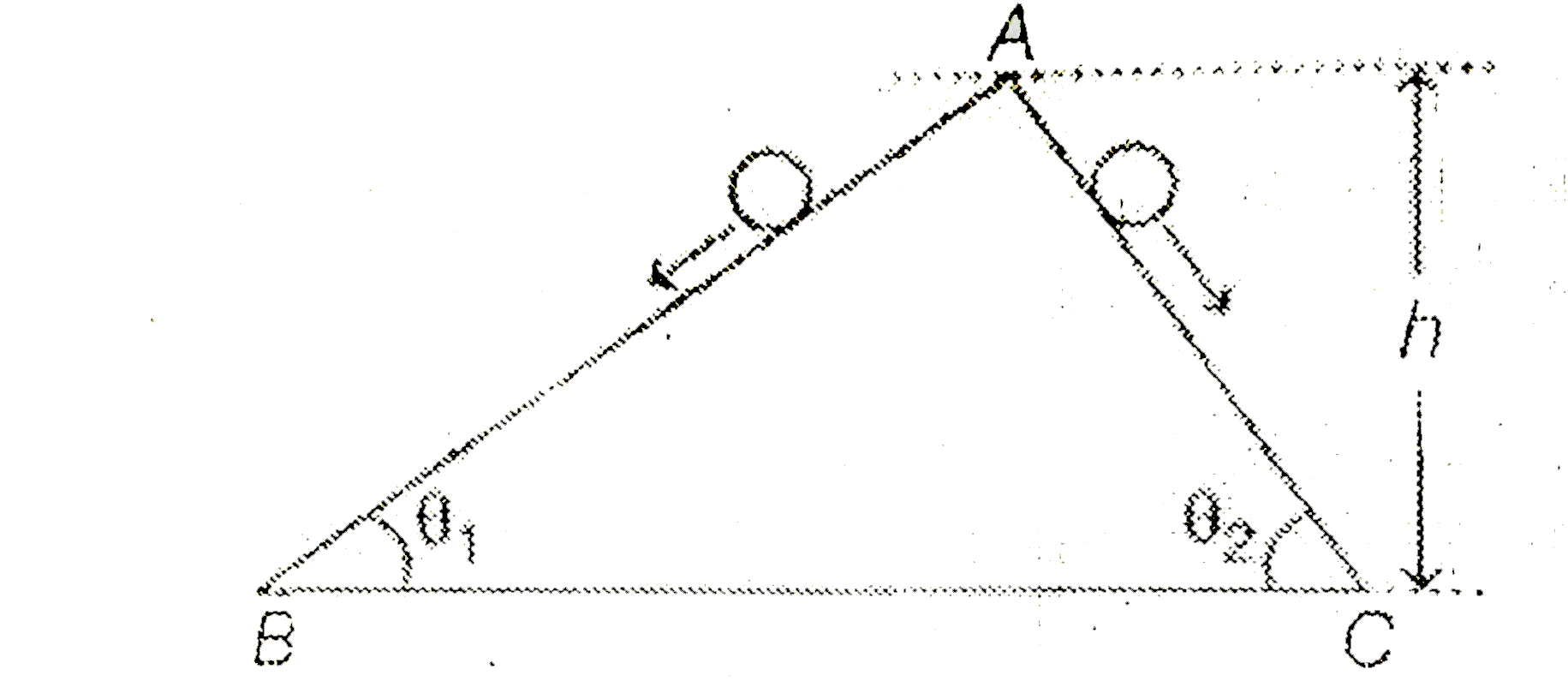
View SolutionA small disc is released from rest at A on an inclined plane AB so that it rolls down without slipping. It reaches the bottom with linear velocity v1 in time t1. Next a small ring released form rest on the inclined plane AC so that it rolls down without slipping. It reaches the bottom with linear vlocity v2 in time t2. Given θ1=30∘,θ2=60∘, and h=10m. Then,
View SolutionA hollow sphere rolls without slipping down a plane inclined at an angle of 30° to the horizontal. Its linear acceleration will be
View Solution
