The activation energies of two reactions are & with . If temperature of reacting system is increased from (rate constant are and ) to (rate constant are and ) predict which of the following alternative is correct.
The correct Answer is:B
To solve the problem, we need to analyze the effect of temperature on the rate constants of two reactions with different activation energies. Here’s a step-by-step solution:
Step 1: Understand the Arrhenius Equation
The Arrhenius equation relates the rate constant (k) of a reaction to its activation energy (Ea) and temperature (T):
k=Ae−EaRT
where:
- k = rate constant
- A = pre-exponential factor
- Ea = activation energy
- R = universal gas constant
- T = temperature in Kelvin
Step 2: Write the Rate Constants for Two Temperatures
For two reactions with activation energies E1 and E2 (where E1>E2), we can express the rate constants at two different temperatures T1 and T2:
1. For the first reaction:
k′1=A1e−E1RT2
k1=A1e−E1RT1
2. For the second reaction:
k′2=A2e−E2RT2
k2=A2e−E2RT1
Step 3: Formulate the Ratios of Rate Constants
We can form the ratios of the rate constants at the two temperatures:
1. For the first reaction:
k′1k1=e−E1RT2e−E1RT1=e−E1RT2+E1RT1=eE1R(1T1−1T2)
2. For the second reaction:
k′2k2=e−E2RT2e−E2RT1=e−E2RT2+E2RT1=eE2R(1T1−1T2)
Step 4: Compare the Ratios
Now we can compare the two ratios:
k′1k1 and k′2k2
Taking the natural logarithm of both ratios:
ln(k′1k1)=E1R(1T1−1T2)
ln(k′2k2)=E2R(1T1−1T2)
Step 5: Analyze the Effect of Activation Energies
Since E1>E2, it follows that:
E1R(1T1−1T2)>E2R(1T1−1T2)
This indicates that:
ln(k′1k1)>ln(k′2k2)
Thus, we conclude:
k′1k1>k′2k2
Conclusion
This means that the increase in the rate constant for the reaction with the higher activation energy E1 is greater than that for the reaction with the lower activation energy E2.
Final Answer
The correct alternative is that k′1k1>k′2k2.
---
The Arrhenius equation relates the rate constant (k) of a reaction to its activation energy (Ea) and temperature (T):
k=Ae−EaRT
where:
- k = rate constant
- A = pre-exponential factor
- Ea = activation energy
- R = universal gas constant
- T = temperature in Kelvin
For two reactions with activation energies E1 and E2 (where E1>E2), we can express the rate constants at two different temperatures T1 and T2:
1. For the first reaction:
k′1=A1e−E1RT2
k1=A1e−E1RT1
k′2=A2e−E2RT2
k2=A2e−E2RT1
We can form the ratios of the rate constants at the two temperatures:
1. For the first reaction:
k′1k1=e−E1RT2e−E1RT1=e−E1RT2+E1RT1=eE1R(1T1−1T2)
k′2k2=e−E2RT2e−E2RT1=e−E2RT2+E2RT1=eE2R(1T1−1T2)
Now we can compare the two ratios:
k′1k1 and k′2k2
Taking the natural logarithm of both ratios:
ln(k′1k1)=E1R(1T1−1T2)
ln(k′2k2)=E2R(1T1−1T2)
Since E1>E2, it follows that:
E1R(1T1−1T2)>E2R(1T1−1T2)
This indicates that:
ln(k′1k1)>ln(k′2k2)
Thus, we conclude:
k′1k1>k′2k2
This means that the increase in the rate constant for the reaction with the higher activation energy E1 is greater than that for the reaction with the lower activation energy E2.
The correct alternative is that k′1k1>k′2k2.
Similar Questions
The activation energies of two reactions are Ea1 and Ea2 with Ea1>Ea2. If the temperature of the reacting systems is increased from T to T', which of the following is correct?
View SolutionThe activation energies of two reactions are Ea1 and Ea2 with Ea1>Ea2. If the temperature of the reacting systems is increased from T to T', which of the following is correct?
View SolutionIf K1= Rate constant at temperature T1 and k2 rate constant at temperature T2 for a first order reaction, then which of the following relation is correct ?
View SolutionThe rate of reaction increases isgnificantly with increase in temperature. Generally, rate of reactions are doubled for every 10∘C rise in temperature. Temperature coefficient gives us an idea about the change in the rate of a reaction for every 10∘C change in temperature.
Temperature coefficient(μ)=Rate constant of(T+10)∘CRate constant atT∘C
Arrhenius gave an equation which describes aret constant k as a function of temperature
k=Ae−Ea/RT
where k is the rate constant, A is the frequency factor or pre-exponential factor, Ea is the activation energy, T is the temperature in kelvin, R is the universal gas constant.
Equation when expressed in logarithmic form becomes
logk=logA−Ea2.303RT
Activation energies of two reaction are Ea and Ea' with Ea>E'a. If the temperature of the reacting systems is increased form T1 to T2 (k' is rate constant at higher temperature).
View SolutionTwo different first order reactions have rate consants k1andk2atT1(k1>k2) . If temperature is increased form T1toT2 ,then new consant become k3andk4 respeectively. Which among the following relartions is correct
View SolutionActivation energy (Ea) and rate constants (k1 and k2) of a chemical reaction at two different temperatures (T1 and T2) are related by
View SolutionA colliison between reactant molecules must occur with a certain minimum energy before it is effective in yielding Product molecules. This minimum energy is called activation energy Ea Large the value of activation energy, smaller the value of rate constant k. Larger is the value of activation energy, greater is the effect of temperature rise on rate constant k.
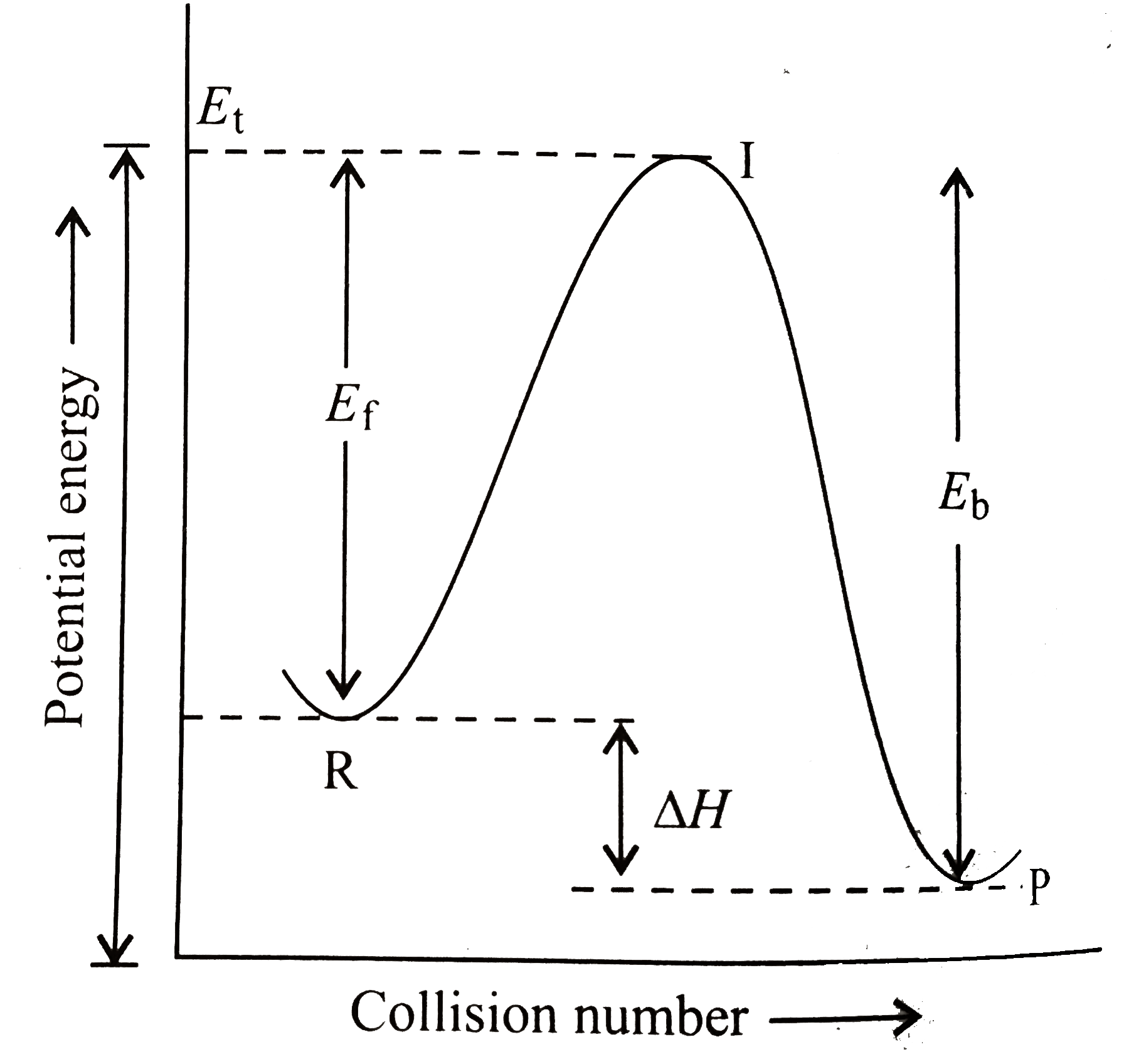
Ef= Activation energy of forward reaction
Eb= Activation energy of backward reaction
ΔH=Ef−Eb
Ef= threshold energy
For two reactions, activation energies are Ea1 and Ea2, rate constant are k1 and k2 at the same temperature. If k1>k2, then
View SolutionActivation energy (Ea) and rate constants (k1andk2) of a chemical reaction at two different temperature (T1andT2) are related by
View SolutionThe rate constants k1 and k2 of two reactions are in the ratio 2:1. The corresponding energies of acativation of the two reaction will be related by
View SolutionRate of two reaction whose rate constants arek1&k2 are equal at 300 k such that: So caculate ln(A2)A1=? Ea2−Ea1=2RT.
View Solution