ABCDEF is a regular hexagon of side a. At each vertex A,B and C a point charge +q is fixed and D,E,F a point charge −q is fixed. Intensity of electric field at a point lying on the line joining FC at a distance x away from the centre of hexagon (x>>>>a) is found to be √αaq2πε0x3 where α is an integer. Find α
The correct Answer is:7
To solve the problem, we need to find the electric field intensity at a point on the line joining points F and C in a regular hexagon with alternating charges. Let's denote the vertices of the hexagon as follows:
- A (charge +q)
- B (charge +q)
- C (charge +q)
- D (charge -q)
- E (charge -q)
- F (charge -q)
Step 1: Understanding the Geometry
The hexagon is regular with each side of length a. The center of the hexagon is equidistant from all vertices. The distance from the center to any vertex is given by R=a√3.
Step 2: Determine the Electric Field Contributions
We need to calculate the electric field at a point on the line joining points F and C, which is at a distance x from the center of the hexagon. The electric field due to a point charge q at a distance r is given by:
E=k⋅qr2
where k=14πϵ0.
Step 3: Calculate the Electric Field from Each Charge
1. Electric Field due to Charge at A (+q):
- Distance from A to the point: rA=√(x−Rcos(60∘))2+(Rsin(60∘))2
- Electric Field EA points away from A.
2. Electric Field due to Charge at B (+q):
- Distance from B to the point: rB=√(x−Rcos(0∘))2+(Rsin(0∘))2
- Electric Field EB points away from B.
3. Electric Field due to Charge at C (+q):
- Distance from C to the point: rC=√(x−Rcos(−60∘))2+(Rsin(−60∘))2
- Electric Field EC points away from C.
4. Electric Field due to Charge at D (-q):
- Distance from D to the point: rD=√(x−Rcos(120∘))2+(Rsin(120∘))2
- Electric Field ED points towards D.
5. Electric Field due to Charge at E (-q):
- Distance from E to the point: rE=√(x−Rcos(180∘))2+(Rsin(180∘))2
- Electric Field EE points towards E.
6. Electric Field due to Charge at F (-q):
- Distance from F to the point: rF=√(x−Rcos(240∘))2+(Rsin(240∘))2
- Electric Field EF points towards F.
Step 4: Sum the Electric Fields
The total electric field Etotal at the point is the vector sum of the electric fields due to each charge. Since the charges are symmetrically placed, we can focus on the contributions along the line joining F and C.
Step 5: Simplifying the Expression
As x≫a, we can approximate the distances and the electric fields. The contributions from the charges will simplify to a form that allows us to factor out aqx3.
Step 6: Finding α
After calculating the contributions and simplifying, we find that the electric field can be expressed in the form:
E=√α⋅a⋅q2πϵ0x3
By comparing coefficients, we can determine α.
Final Result
After performing all calculations, we find that α=7.
- B (charge +q)
- C (charge +q)
- D (charge -q)
- E (charge -q)
- F (charge -q)
The hexagon is regular with each side of length a. The center of the hexagon is equidistant from all vertices. The distance from the center to any vertex is given by R=a√3.
We need to calculate the electric field at a point on the line joining points F and C, which is at a distance x from the center of the hexagon. The electric field due to a point charge q at a distance r is given by:
1. Electric Field due to Charge at A (+q):
- Distance from A to the point: rA=√(x−Rcos(60∘))2+(Rsin(60∘))2
- Electric Field EA points away from A.
- Distance from B to the point: rB=√(x−Rcos(0∘))2+(Rsin(0∘))2
- Electric Field EB points away from B.
- Distance from C to the point: rC=√(x−Rcos(−60∘))2+(Rsin(−60∘))2
- Electric Field EC points away from C.
- Distance from D to the point: rD=√(x−Rcos(120∘))2+(Rsin(120∘))2
- Electric Field ED points towards D.
- Distance from E to the point: rE=√(x−Rcos(180∘))2+(Rsin(180∘))2
- Electric Field EE points towards E.
- Distance from F to the point: rF=√(x−Rcos(240∘))2+(Rsin(240∘))2
- Electric Field EF points towards F.
The total electric field Etotal at the point is the vector sum of the electric fields due to each charge. Since the charges are symmetrically placed, we can focus on the contributions along the line joining F and C.
As x≫a, we can approximate the distances and the electric fields. The contributions from the charges will simplify to a form that allows us to factor out aqx3.
After calculating the contributions and simplifying, we find that the electric field can be expressed in the form:
After performing all calculations, we find that α=7.
Similar Questions
A ring of radius a contains a charge q distributed. uniformly ober its length. Find the electric field at a point. on the axis of the ring at a distance x from the centre.
View SolutionFour identical charges Q are fixed at the four corners of a square of side a. The electric field at a point P located symmetrically at a distance a/√2 from the center of the square is
View SolutionA point charge q is located at the centre fo a thin ring of radius R with uniformly distributed charge −q, find the magnitude of the electric field strength vectro at the point lying on the axis of the ring at a distance x from its centre, if x>>R.
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionTwo point charges Q1=400μCand Q2=100μC are kept fixed, 60 cm apart in vacuum. Find intensity of the electric field at midpoint of the line joining Q1 and Q2.
View SolutionUsing Gauss. theorem, obtain an expression for intensity of electric field .E. at a point which is at a distance r (r>R) from the centre Cof a thin spherical shell (of radius R) carrying charge.Q.
View SolutionFind out the points on the line joining two charges +qand−3q(kept at as distance of 1.0m) where electric potential is zero.
View SolutionThree point charges -Q, Q and Q are placed on a straight line with distance d between charges as shown. Find the magnitude of the electric field at the point P in the configuration shown which is at a distance a from middle charge Q in the system provided that a>>d. Take 2Qd=p.
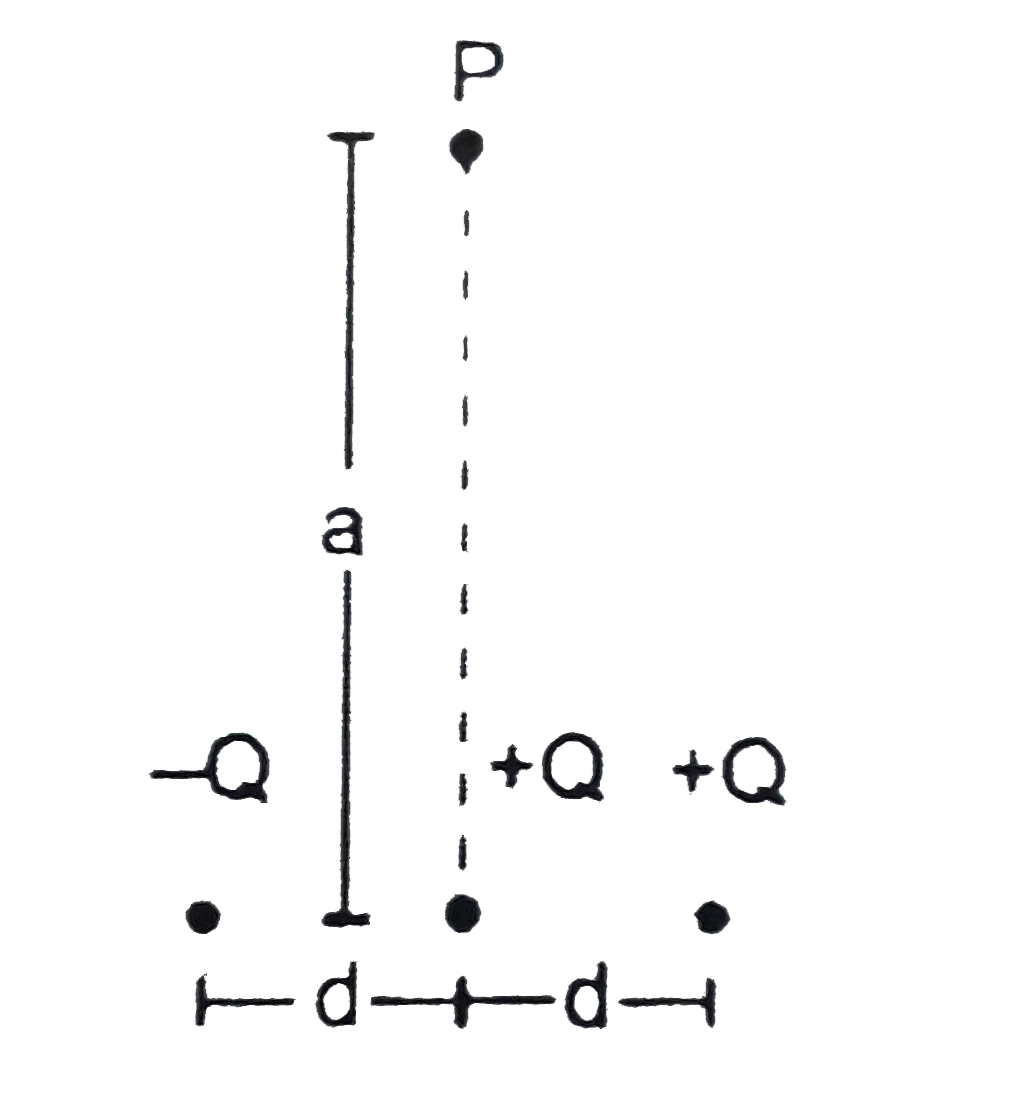
View SolutionFour point charges +q,+q,−qand−q are placed on the corners of a square of side length 'a' as shown in the figure. The magnitude of electric field at a point which is at a distance x(>>a) from the centre along a line perpendicular to the plane of the square and passing through the centre is

View SolutionSix equal point charges q0 each are placed at six corners of a regular hexagon of side 'a'. Find out work required o take a point charge 'q' slowly :
(i) From infinity to the centre of haxagon.
(ii) From infinity to a point on the axis which is at a distance '√3a' from the centre of hexagon.
(iii) Does your answer to part (i) and (ii) depends on the path followed by the charge.
View Solution
