In th circuit the key (K) is closed at t=0. Find the current in ampere through the key (K) at the instant t=102ln2 sec.
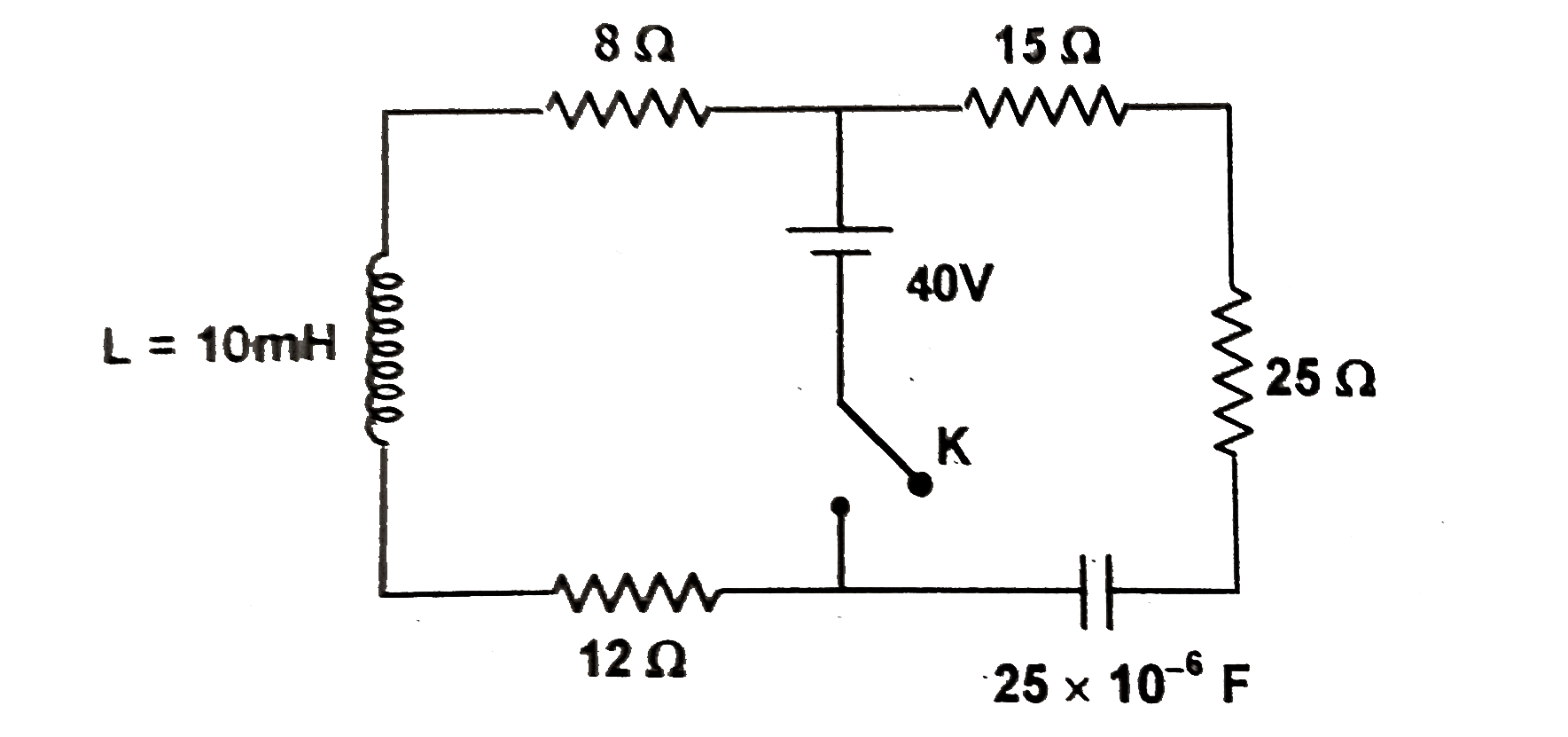
The correct Answer is:2
i1 is current in the first loop
i1=4020(1−et5×10−4)=32 amp
i2 is current in the second loop
i2=4040e−t10−3=12 amp
So, total current through key (K)
i=i1+i2
=32+12=2 amp

Similar Questions
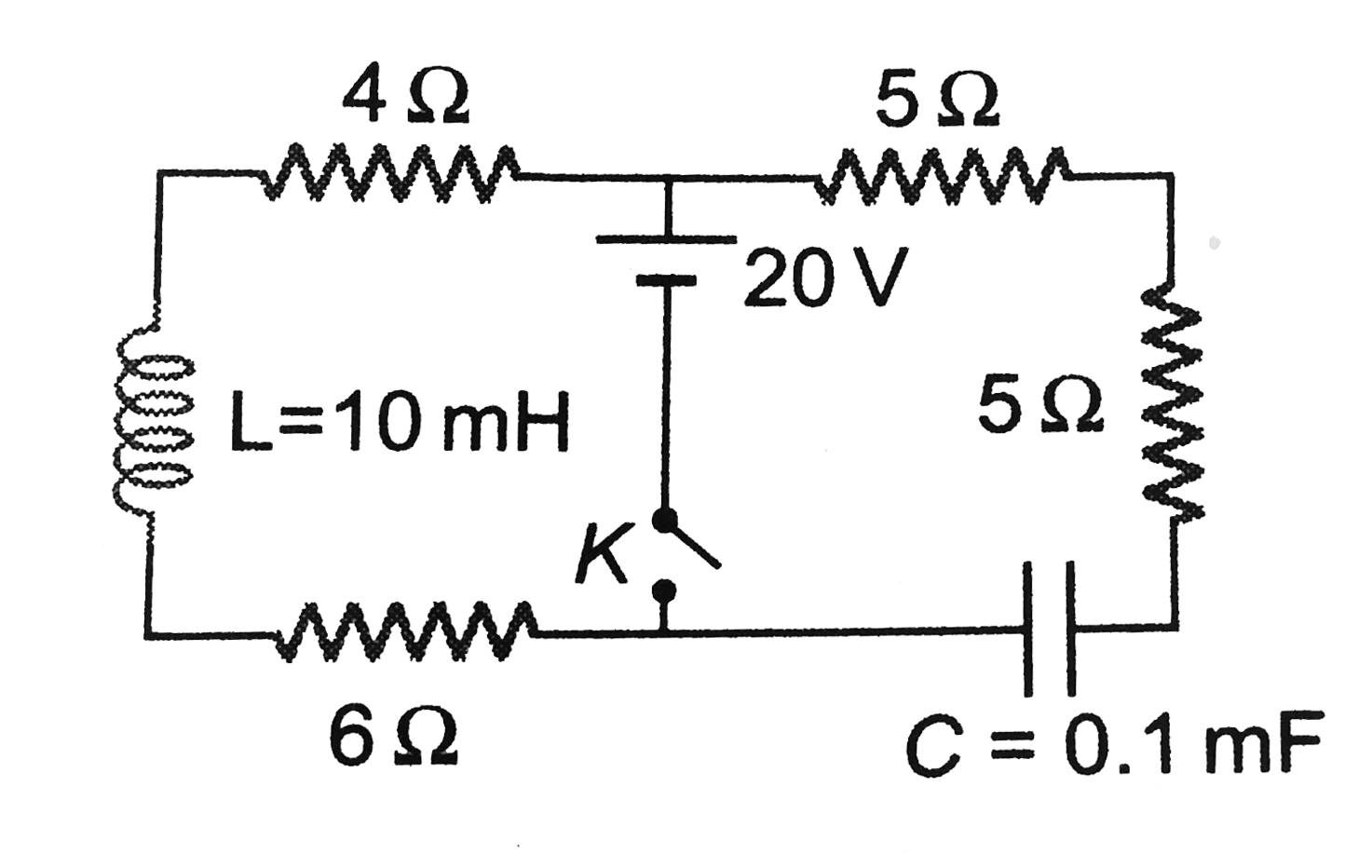
In the circuit shown the key (K) is closed at t=0, the current through the key at the instant t=10−3ln,2 is
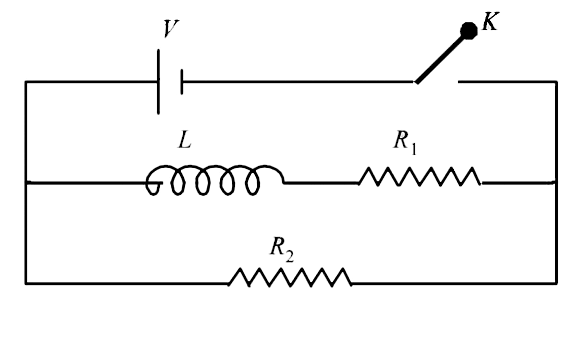
View SolutionIn the circuit shown below, the key K is closed at t =0. The current through the battery is
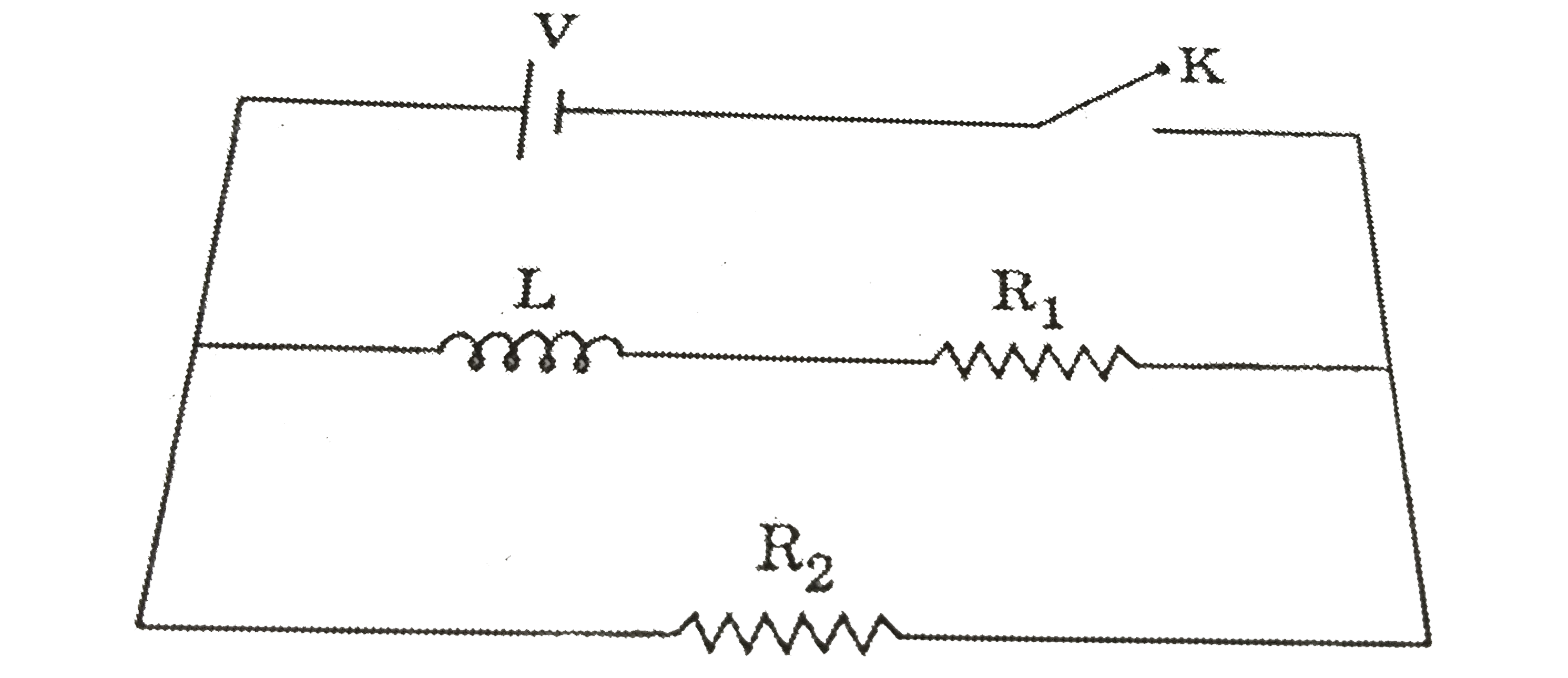
View SolutionIn the circuit shown below, the key K is closed at t = 0. The current through the battery is
View SolutionIn the circuit shown key is closed at t=0 with capacitor initially uncharged.
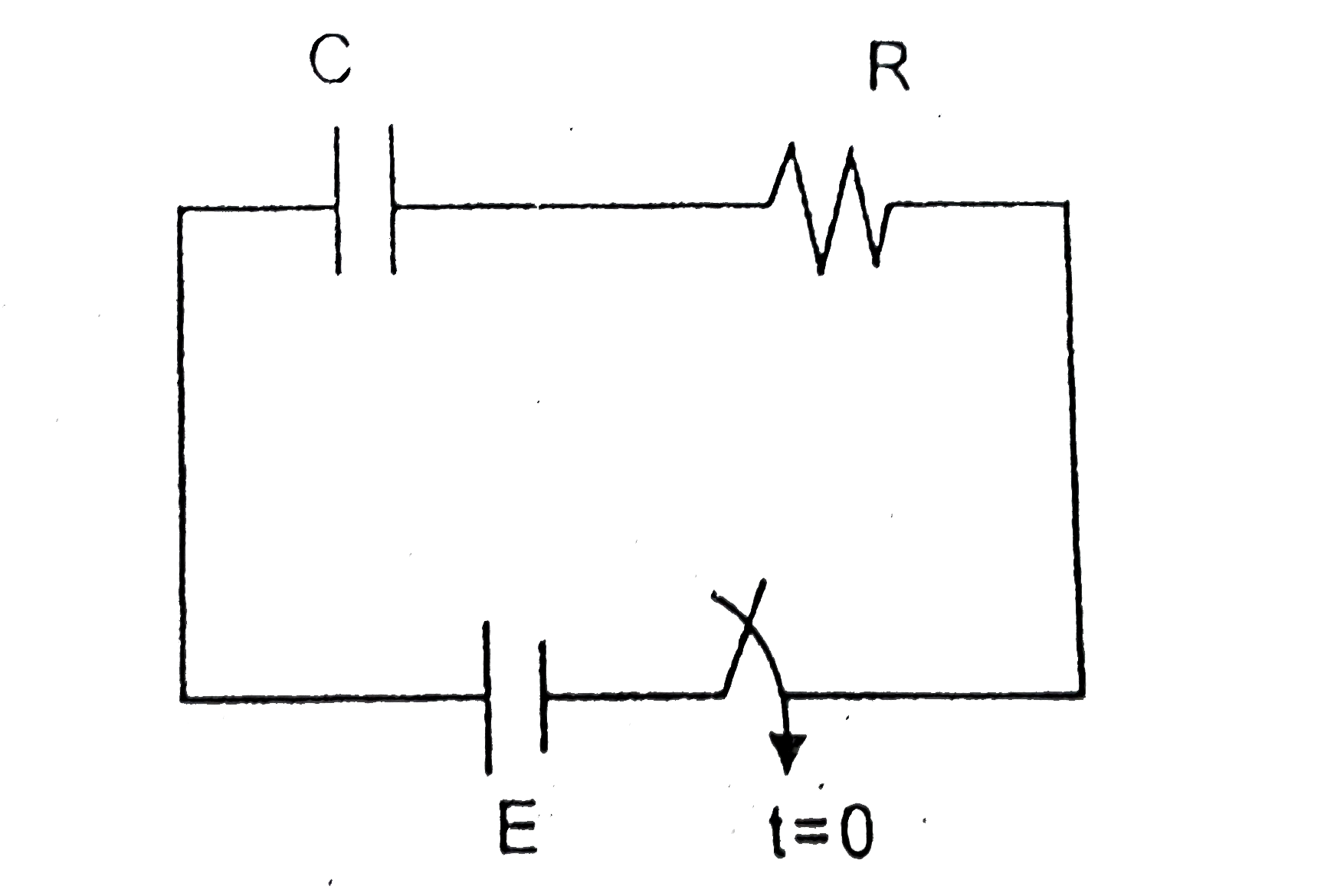
View SolutionIn the circuit shown, switch S is closed at time t=0. Find the current through the inductor as a function of time t.

View SolutionSwitch is closed at t=0 then the current in the circuit at t=L2R is
_PHY_E06_157_Q01.png)
View SolutionConsider the circuit shown. Switch is closed at t=0. Find the current through the battery as a function of time. Initially capacitor was uncharged.
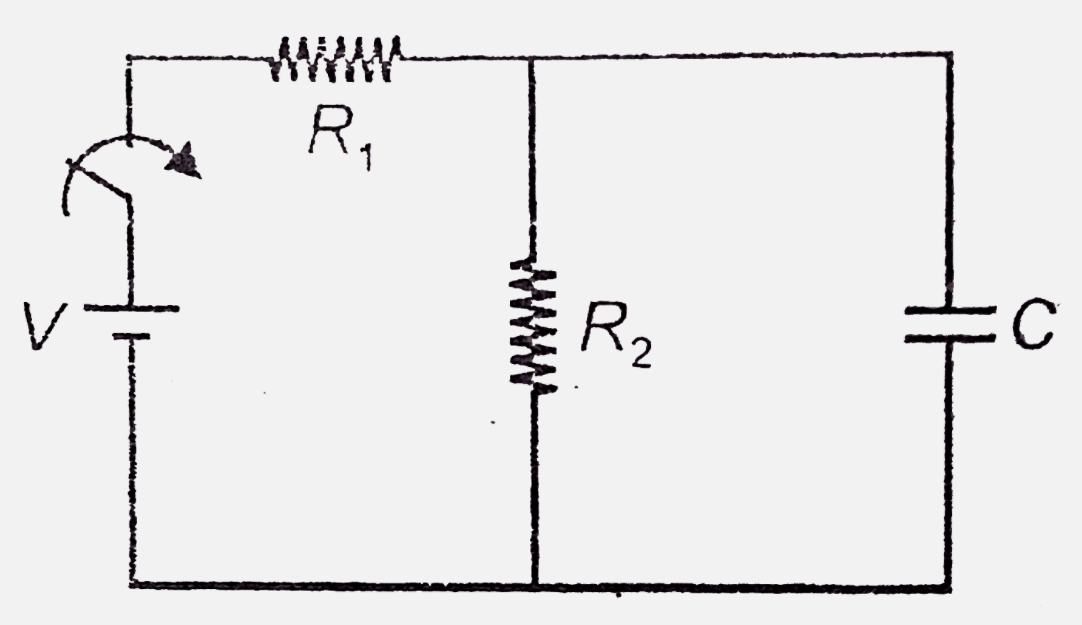
View SolutionIn the circuit shown below, the key is pressed at time t = 0. The correct statement is
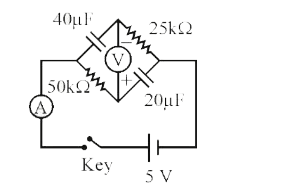
View SolutionIn the given circuit (Fig), key K is switched on the at t=0. The ratio of current i through the cell at t=0 to that at t=∞ will be

View SolutionIn the given circuit (Fig), key K is witched on the at t=0. The ratio of current i through the cell at t=0 to that at t=∞ will be

View Solution