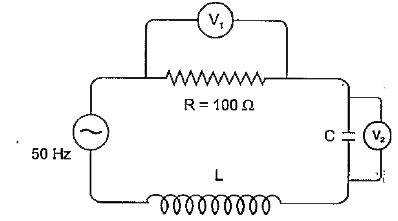
In an LCR series circuit, the reading of ideal voltmeters $V_{1}$ and $V_{2}$ are $20 \sqrt{2}$ volt and 15 volts respectively as shown in the figure (the given circuit in inductive in nature). Then

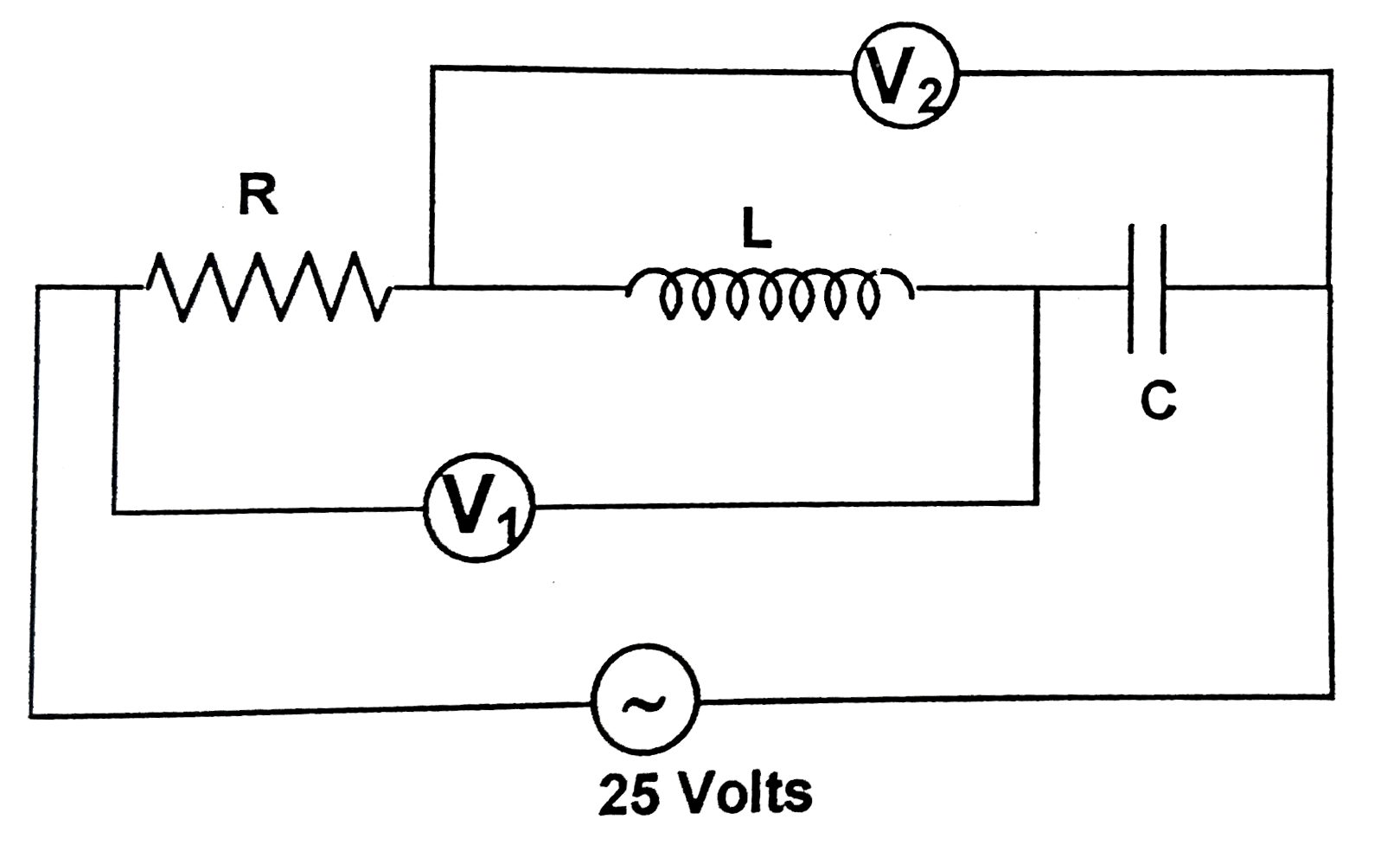
The voltage across resistor is 20 volts

The voltage across inductor is 20 volts

The voltage across capacitor is 5 volts

The power factor of the circuit is $\frac{4}{5}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C, D

$V_{R}^{2} + V_{L}^{2} = V_{1}^{2} = {(20 \sqrt{2})}^{2} = 800$ ……(i)
$V_{L} - V_{C} = 15$ …………….(ii)
$\sqrt{V_{R}^{2} + {(V_{L} - V_{C})}_{L}^{2}} = {(25)}^{2}$
$V_{R}^{2} + 225 = 625$

$V_{0} =$ source voltage
$\Rightarrow V_{R} = 20$ volts
$V_{L} = 20$ volts
$V_{L} - V_{C} = 15$
$20 - V_{C} = 15$
$V_{C} = 5$ volts
Power factor $= cos ϕ = \frac{R}{2} = \frac{i R}{i Z} = \frac{V_{R}}{V_{Z}} = \frac{20}{25} = \frac{4}{5}$

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Similar Questions

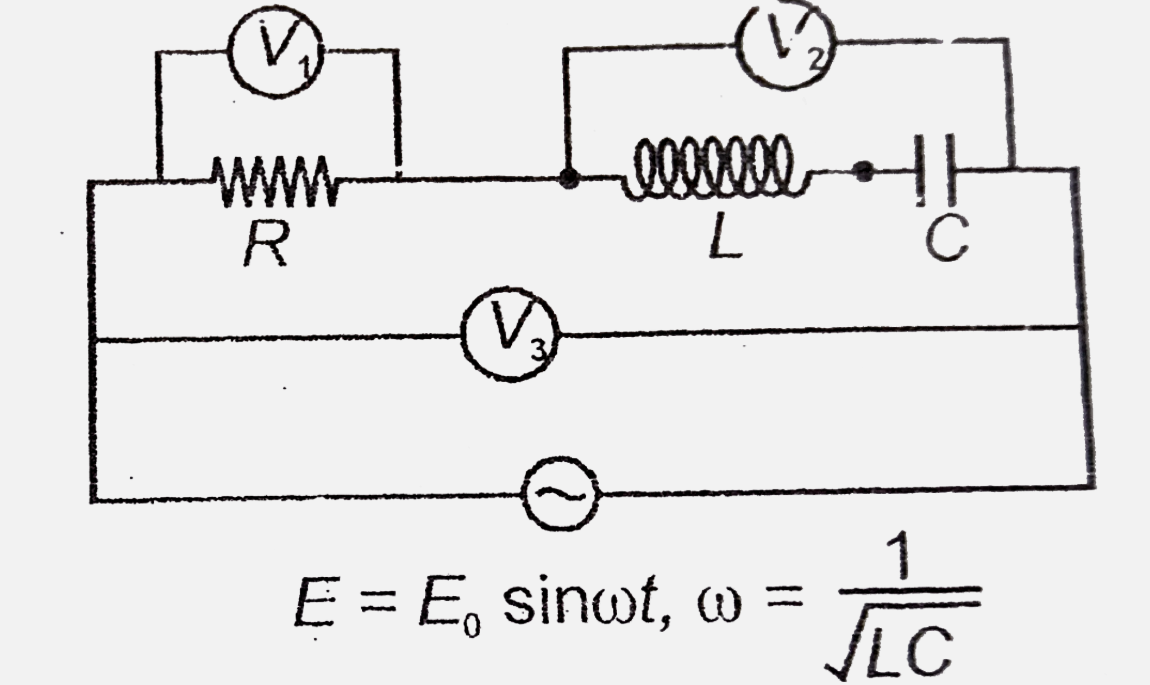
In the circuit shown, the readings of voltmeters $V_{1}, V_{2} and V_{3}$ are given by

View Solution
In the circuit shown below. The reading of the voltmeter V is

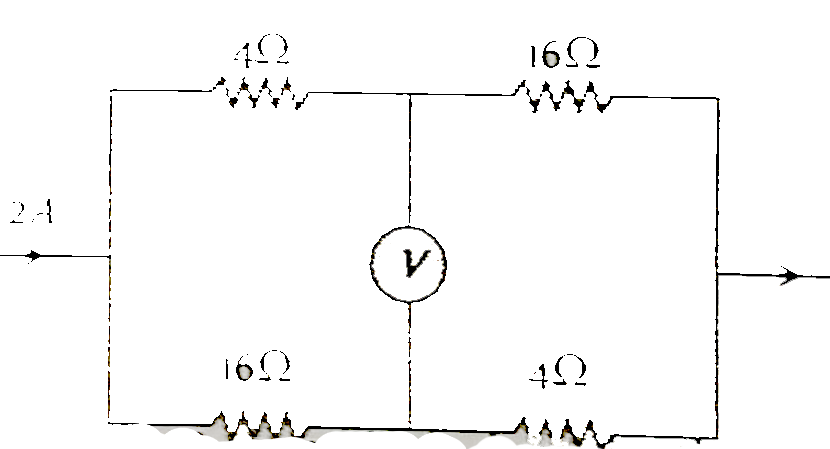View Solution
In a $R - L - C$ series circuit shown in readings of voltmeters $V_{1}$ and $V_{2}$ are $100 V$ and $120 V$ .
Choose the corredct statement(s).
.
View Solution
In the following circuit, determine the reading of ideal voltmeter (V) and ideal ammeter (A).

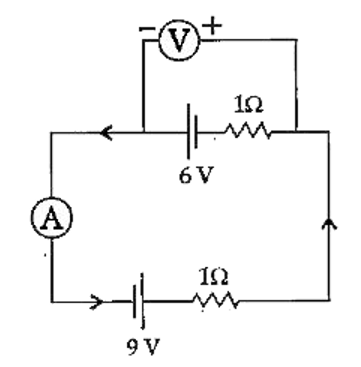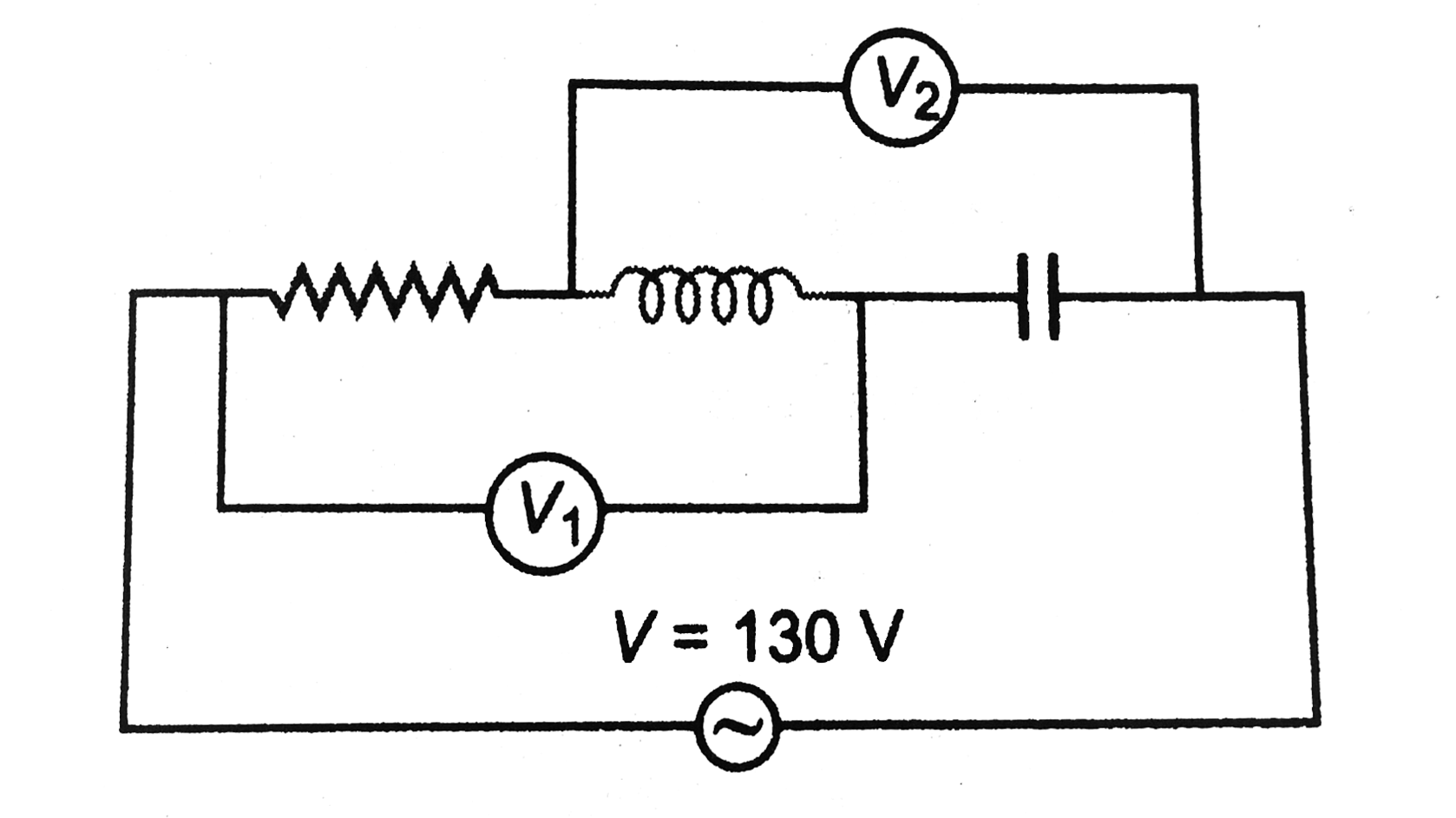
View Solution
In an RLC series circuit shown in fig. the reading of voltmeters $(V_{1}) and (V_{2})$ are 100 V and 200 V respectively. The source voltage is 130 V. for this situation, mark out the correct statements (s).

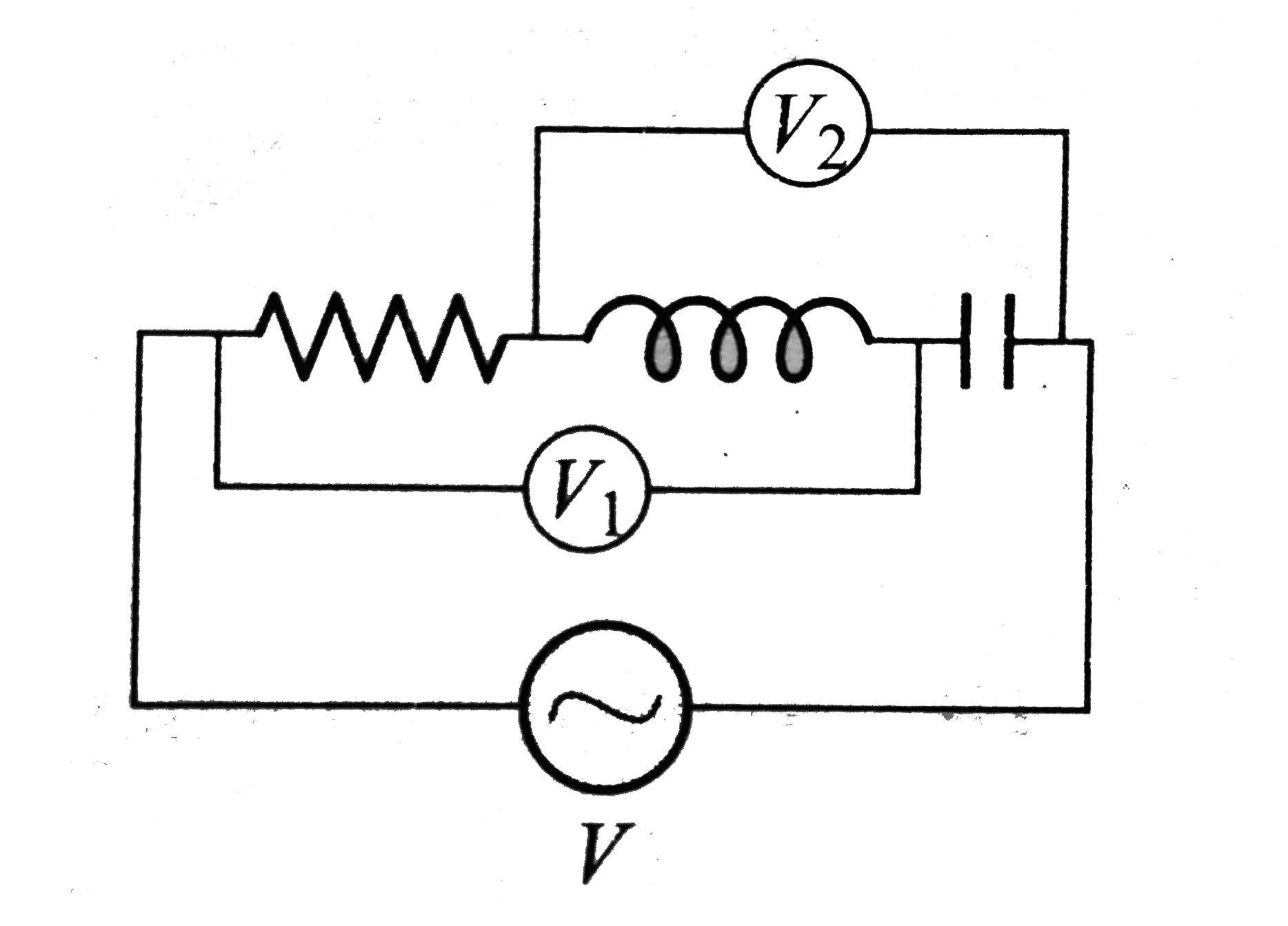View Solution
In the circuit shown in the figure, the voltmeter reading is

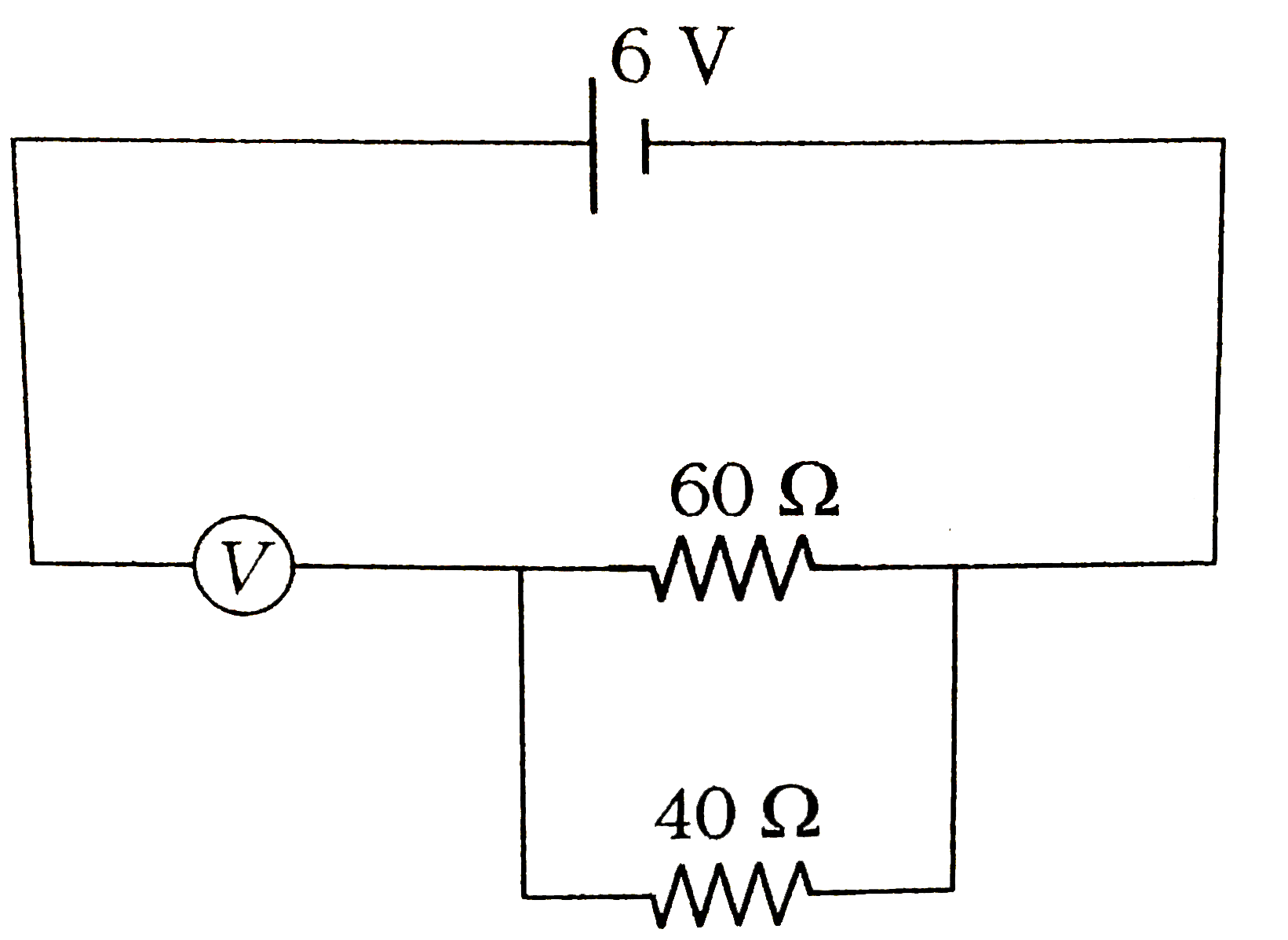View Solution
In the given circuit, the reading of ideal voltmeter is $E / 2$ . The internal resistance of the battery is

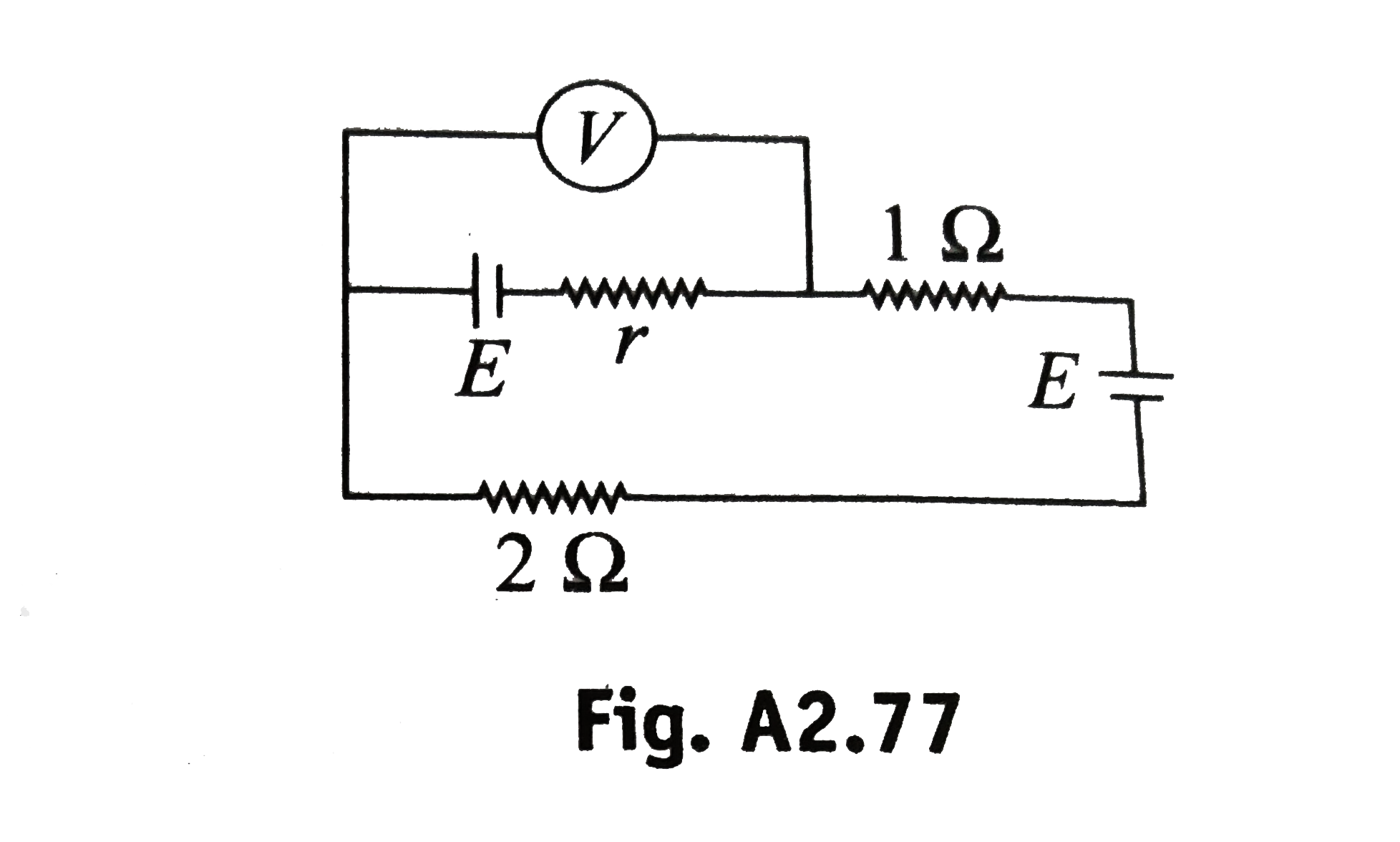View Solution
Consider the circuit shown in the figure, the cell is ideal. The readings of the ideal voltmeters $V_{1}$ and $V_{2}$ in volts are respectively.

View Solution
Following figure 6 shows a series RCL circuit connected to an ac source which generates an alternating emf of frequency 50 Hz. The readings of the voltmeters $V_{1}$ and $V_{2}$ are 80 V and 60 V respectively.

(1) the current in the circuit.
(2) the capacitance of the capacitor.
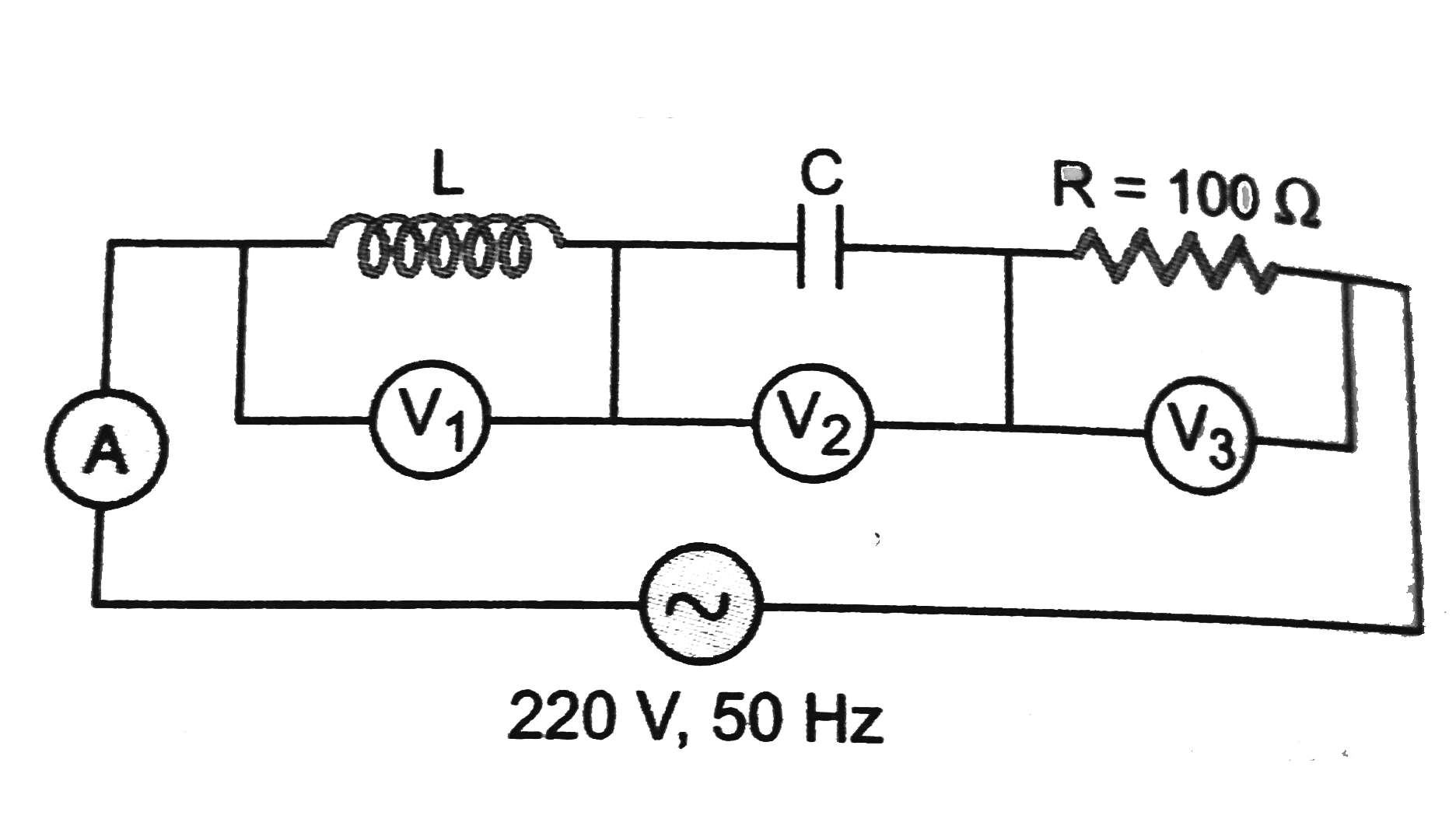
(ii) At resonance, what is the relation between impedance of a series LCR circuit and its resistance R?
View Solution
In the given circuit, Fig., the reading of voltmeter $V_{1}$ and $V_{2}$ 300 votls each. The reading of the voltemeter $V_{3}$ and ammeter $A$ are respectively

View Solution

In an LCR series circuit, the reading of ideal voltmeters V1 and V2 are 20√2 volt and 15 volts respectively as shown in the figure (the given circuit in inductive in nature). Then

The correct Answer is:A, B, C, D

V2R+V2L=V21=(20√2)2=800……(i) VL−VC=15 …………….(ii) √V2R+(VL−VC)2=(25)2 V2R+225=625 V0= source voltage ⇒VR=20 volts VL=20 volts VL−VC=15 20−VC=15 VC=5 volts Power factor =cosϕ=R2=iRiZ=VRVZ=2025=45

Similar Questions

Recommended Questions

In an LCR series circuit, the reading of ideal voltmeters $V_{1}$ and $V_{2}$ are $20 \sqrt{2}$ volt and 15 volts respectively as shown in the figure (the given circuit in inductive in nature). Then