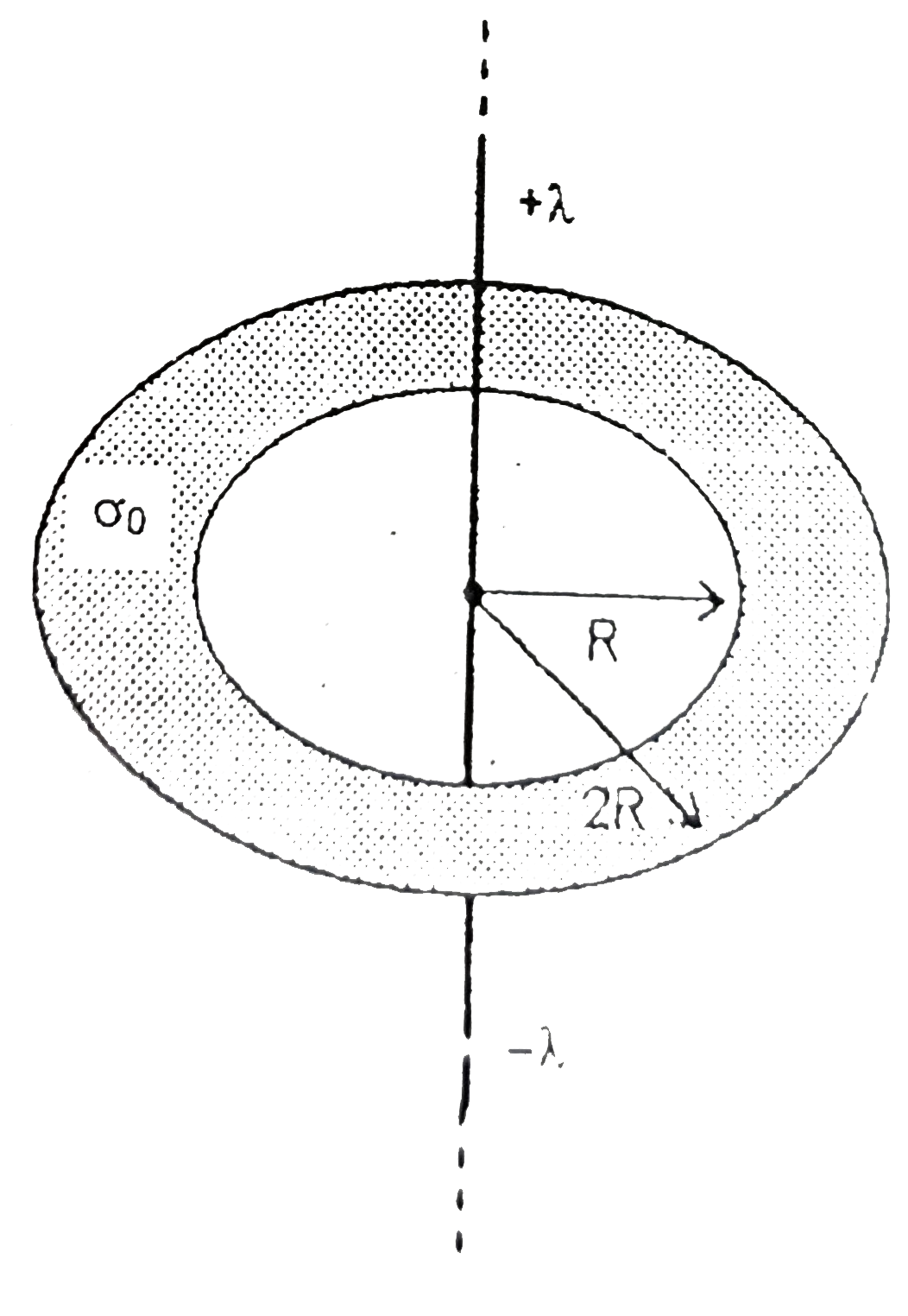
A non conducting infinite rod is placed along the z−axis the upper half of the rod (lying along z≥0) is charged positively with a uniform linear charge density +λ, while the lower half (z<0) is charged negatively with a uniform linear charge density −λ. The origin is located at the junction of the positive and negative halves of the rod. A uniformly charged annular disc (surface charge density : σ0) of inner radius R and outer radius 2R is placed in the x−y plane with its centre at the origin. find the force on the rod due to the disc.
2σ0λRε0
σ0λR2ε0
σ0λRε0
σ0λR3ε0
The force on the rod due to the annular disc is equal and opposite to that on the disc due to the rod. We take an annular strip of radius r and width dr and find the force acting on it
dF=(σ02πrdr)λ2πε0r=λσ0ε0dr
∴ The force
F=σ0λε0∫2RRdr=σ0λRε0
Similar Questions
The dimensional formula of linear charge density λ is
View SolutionA half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
View SolutionIf linear charge density of a wire as shown in the figure is λ
 View Solution
View SolutionWhat is the electric field intensity at any point on the axis of a charged rod of length 'L' and linear charge density λ? The point is separated from the nearer end by a.
View SolutionConsider an infinite line charge having uniform linear charge density and passing through the axis of a cylinder is removed.
 View Solution
View SolutionA wire AMB is placed along y-axis having linear charge density λ C/m. The electric field intensity at point P is ( PM=0.1 m)
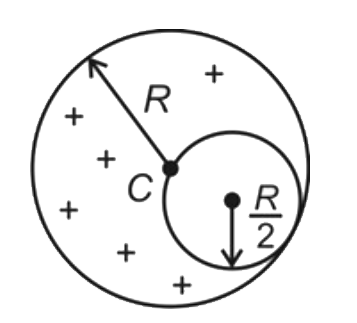
View SolutionA non - conducting disc of radius R is uniformly charged with surface charge density σ. A disc of radius R2 is cut from the disc, as shown in the figure. The electric potential at centre C of large disc will be
 View Solution
View SolutionA rod of length l, has a uniform positive charge per unit length and a total charge Q. calculate the electric field at a point P located along the long axis of the rod and at a distance a from one end
 View Solution
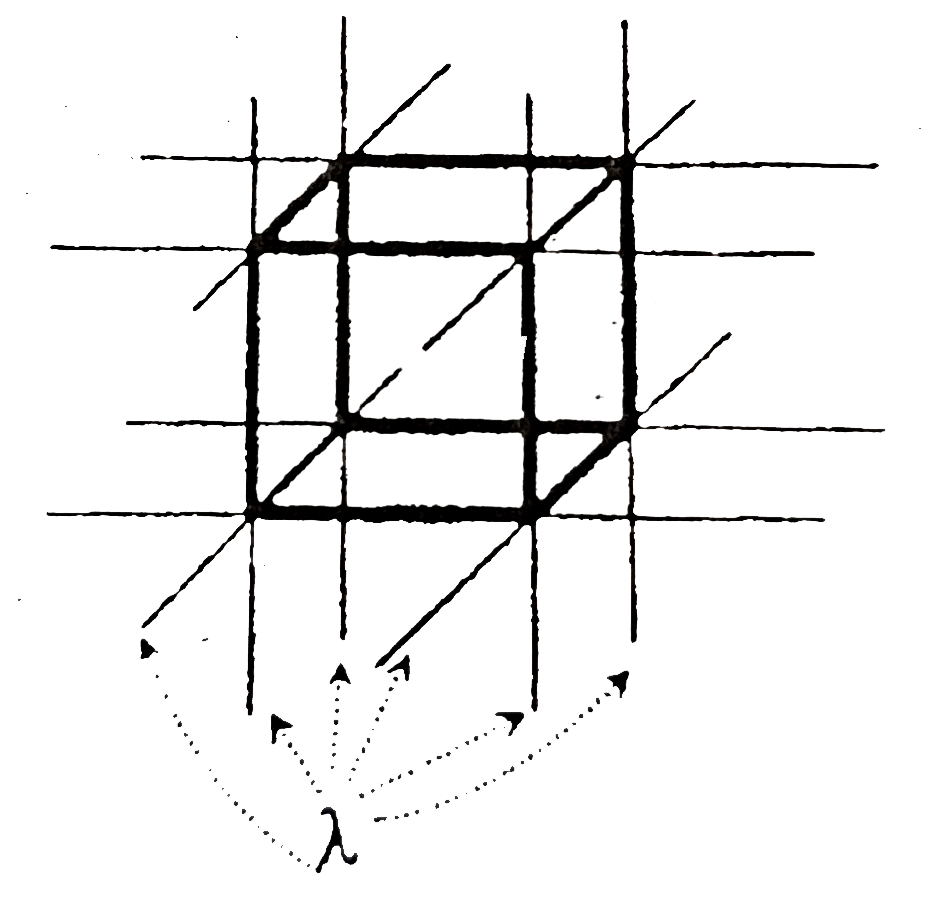
View SolutionTwelve infinite long wire of uniform linear charge density (λ) are passing along the twelve edges of a cube. Find electric flux through any face of cube. ,
 View Solution
View SolutionIntensity of electric field at a point at a perpendicular distance .r. from an infinite line charge, having linear charge density .λ. is given by :
View Solution

