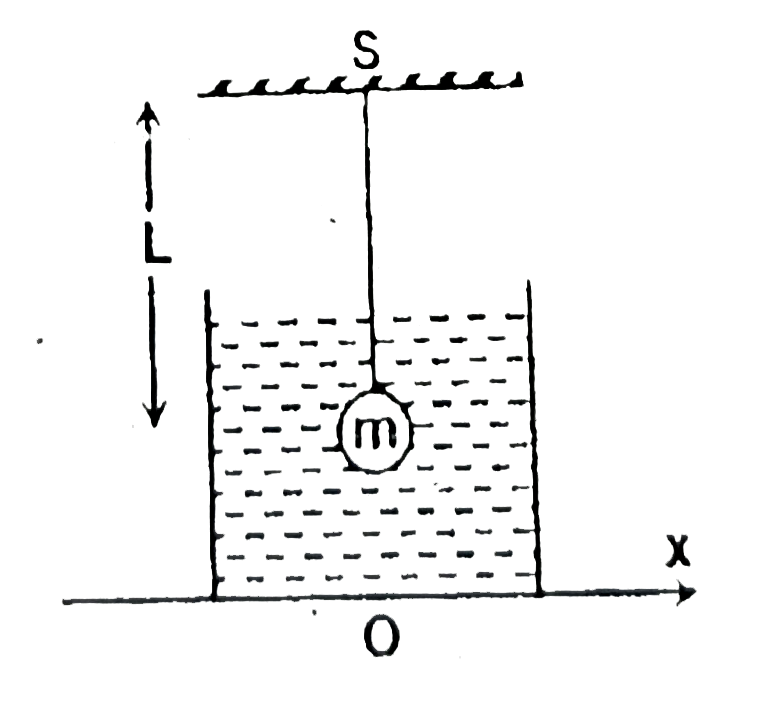
A beaker containing an ideal fluid executes plane in a horizontal plane according to the equation , being the mean position. A bob is suspended at through a string of length as shown in the figure. The line is vertical. Assuming .
The tension in the string is maximum at time
none of these
Tension is maximum at the mean position.
Similar Questions
A beaker containing an ideal fluid executes plane SHM in a horizontal plane according to the equation x=√3gω2sinωt, O being the mean position. A bob is suspended at S through a string of length L as shown in the figure. The line SO is vertical. Assuming L>>√3gω2.
The magnitude of maximum buoyant force and the time with it occurs for the second time are, respectively
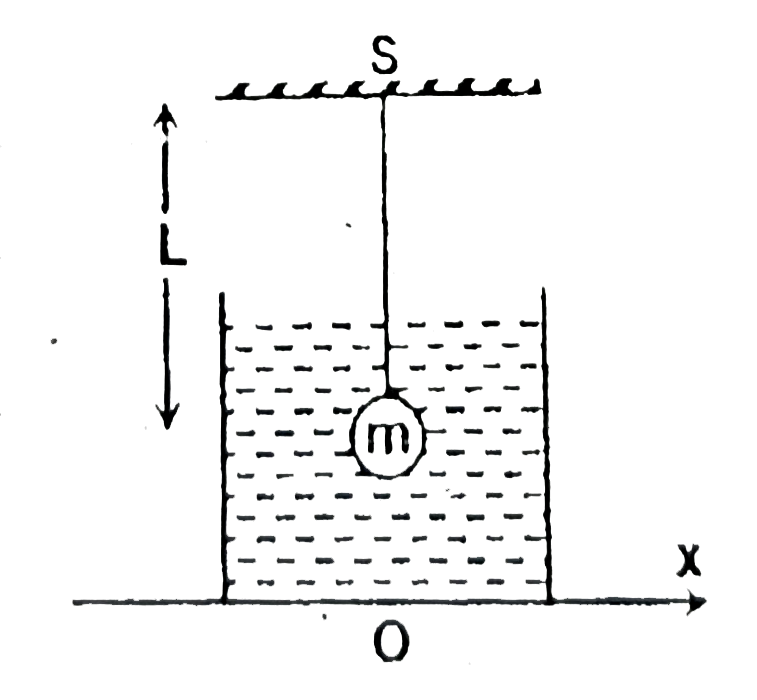 View Solution
View SolutionA beaker containing an ideal fluid executes plane SHM in a horizontal plane according to the equation x=√3gω2sinωt, O being the mean position. A bob is suspended at S through a string of length L as shown in the figure. The line SO is vertical. Assuming L>>√3gω2.
The direction of buoyant force at time t=π/2ω is best represented by
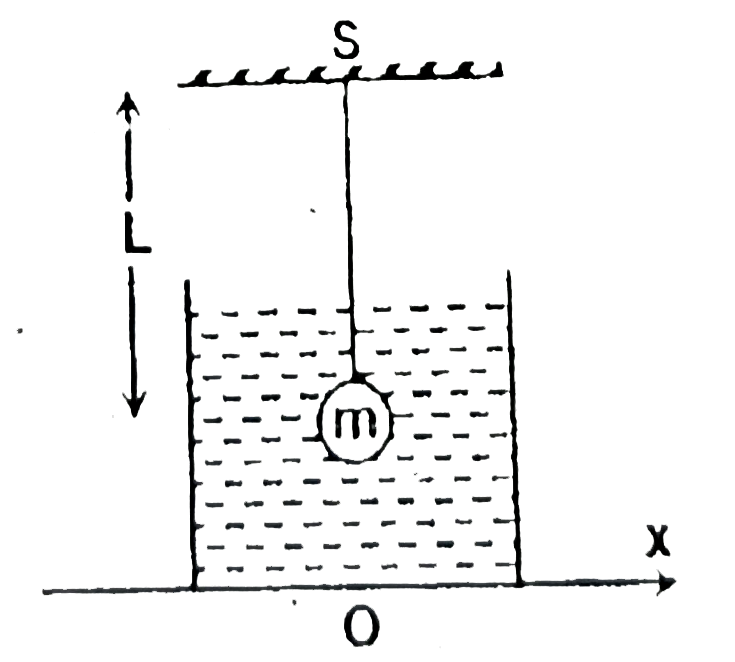 View Solution
View SolutionA uniform rod of mass m and length L is suspended with two massless strings as shown in the figure. If the rod is at rest in a horizontal position the ratio of tension in the two strings T1T2 is:
 View Solution
View SolutionA car is moving on a plane inclined at 30∘ to the horizontal with an acceleration of 10m/s2 parallel to the plane upward. A bob is suspended by a string from the roof. The angle in degrees which the string makes with the vertical is: (Assume that the bob does not move relative to car) [g=10m/s2]
View SolutionIn YDSE set up (see fig.), the light sources executes SHM between P and Q according to the equation x=Asinωt, S being the mean position. Assume d→0 and A<<L. ω is small enough to neglect Doppler effect. If the sources were stationary at S, intenstiy at O would be I0
Read the paragraph carefully and answer the following questions.
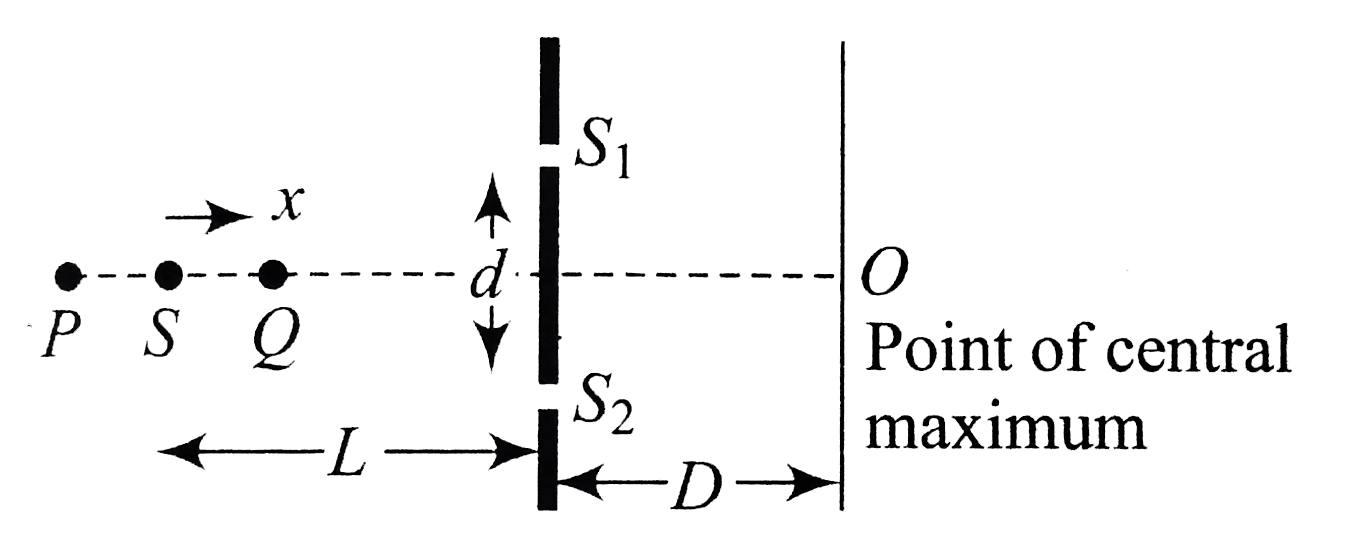
The fringe width β can be expressed asView SolutionIn YDSE set up (see fig.), the light sources executes SHM between P and Q according to the equation x=Asinωt, S being the mean position. Assume d→0 and A<<L. ω is small enough to neglect Doppler effect. If the sources were stationary at S, intenstiy at O would be I0
Read the paragraph carefully and answer the following questions.
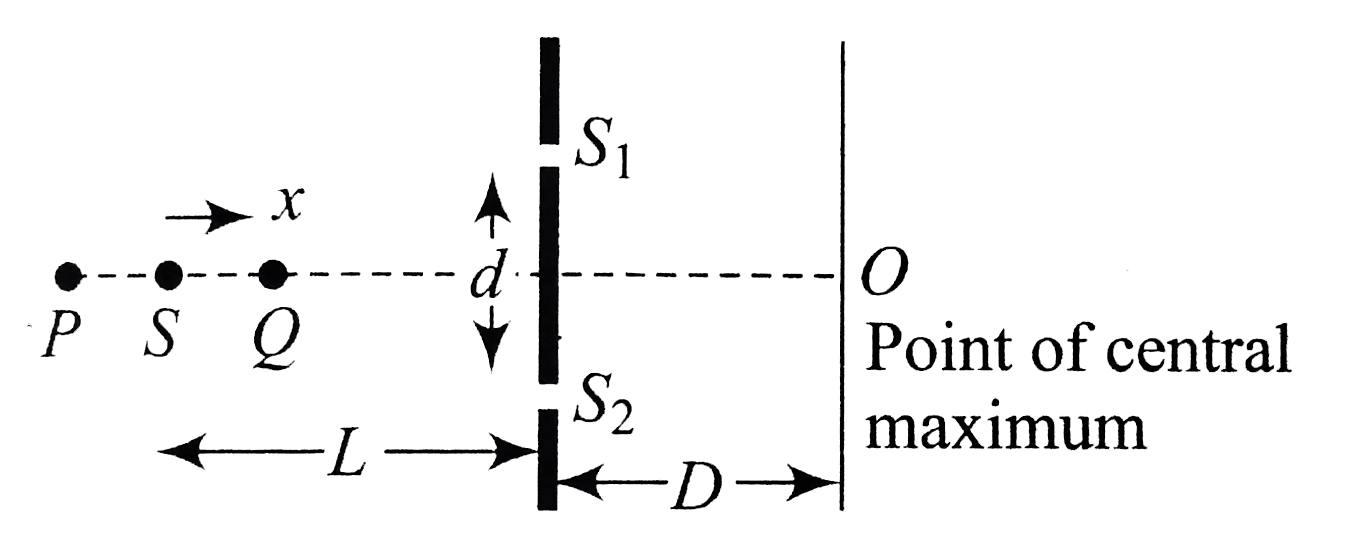
The fractional change in intensity of the central maximum as function of time isView SolutionIn YDSE set up (see fig.), the light sources executes SHM between P and Q according to the equation x=Asinωt, S being the mean position. Assume d→0 and A<<L. ω is small enough to neglect Doppler effect. If the sources were stationary at S, intenstiy at O would be I0
Read the paragraph carefully and answer the following questions.
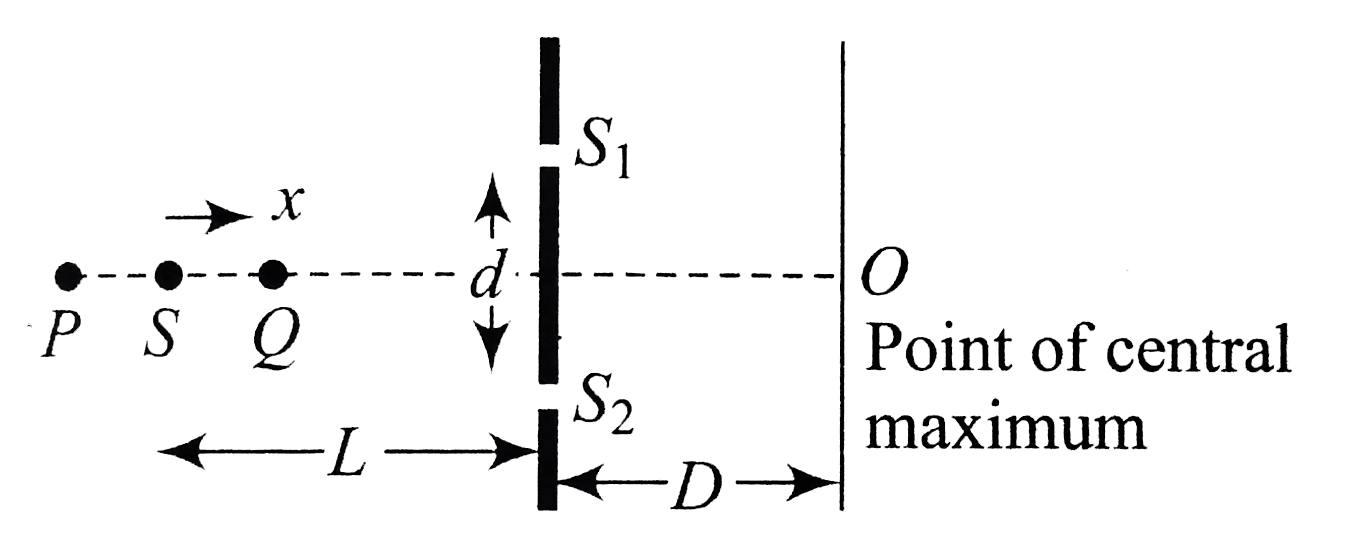
When the source comes toward the point Q,View SolutionA car is moving with constant speed of 10m/s on a horizontal circular path of radius 10√3m . A bob of mass m suspended through a light string from the roof of the car. What is the angle made by the string with the vertical if the bob is stationary with respect to the car? (g = 10 m/s2)
View SolutionA uniform rod length is l hinged at A and suspended with vertical string as shown in figure it string is cut then acceleration of centre of mass at this instant will be (g=10ms2)
View SolutionA body of mass 20kg is moving on a rough horizontal plane. A block of mass 3kg is connected to the 20 kg mass by a string of negligible mass through a smooth pulley as shown in the figure. The tension in the string is 27 N. The coefficient of kinetic friction between the heavier mass and the surface is (g = 10m/s2)
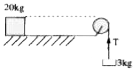 View Solution
View Solution
