A steel rod with a cross-sectional area of 150mm2 is stretched between two fixed points. The tensile load at 20∘C is 5000N.
(a) What will be the stress at −20∘C ?
(b) At what temperature will the stress be zero ?
(Assume α=11.7μm/m∘C and Y=200GN/m2)
(a) Let L be the free length of the rod at 0∘C and L0 be the final stretched length in each case.
If L1 and L2 are the free lengths at +20∘C and −20∘C, respectively, then using geometric conditions δ2=δ1+2αLΔT, where δ1 and δ2 are the load deformations at +20∘C and −20∘C, respectively.
Now, F2LAY=F1LAY+2αLΔT
or, σ2=F2A=F1A+2αYΔT
∴σ2=5000150×10−6+2(11.7×10−6)(2×1011)(20)
=127×106N/m2
(b) Let T' be the temperature at which the stress is zero, then
αLT'=αLΔT+δ1
or T'=ΔT+F1AYα
T'=20+5000(150×10−6)(2×1011)(11.7×10−6)
=34.2∘C

Topper's Solved these Questions
Similar Questions
What will be the stress at −20∘C, if a steel rod with a cross-sectional area of 150 mm2 is stretched between two fixed points? The tensile load at 20∘C is 5000 N (Assume, α=11.7×10−6/∘C and Y = 200×1011N//m^(2))
View SolutionA steel wire of cross-sectional area 0.5mm2 is held between two fixed supports. If the wire is just taut at 20∘C, determine the tension when the temperature falls to 0∘C. Coefficient of linear expansion of steel is 1.2×10−5C−1 and its Young's modulus is 2.0×1011Nm−2.
View Solution
Knowledge Check
- Question 1 - Select One
A steel rod of length 1 m and radius 10 mm is stretched by a force 100 kN along its length. The stress produced in the rod is YSteel=2×1011Nm−2
A3.18×106Nm−2B3.18×107Nm−2C3.18×108Nm−2D3.18×109Nm−2
A steel wire of cross-sectional area 0.5mm2 is held between two fixed supports. If the wire is just taut at 20∘C, determine the tension when the temperature falls to 0∘C. Coefficient of linear expansion of steel is 1.2×10−5∘C(−1) and its Young's modulus is 2.0×1011Nm−2.
View SolutionA brass of length 2m and cross-sectional area 2.0cm2 is attached end to end to a steel rod of length and cross-sectional area 1.0cm2. The compound rod is subjected to equal and oppsite pulls of magnitude 5×104N at its ends. If the elongations of the two rods are equal, the length of the steel rod (L) is
{YBrass=1.0×1011N/m2 and Ysteel=2.0×1011N/m2View SolutionA metal rod of length 2 m has cross sectional areas 2 A and A as shown in figure. The ends are maintained at temperatures 100° C and 70° C . The temperature at middle point C is
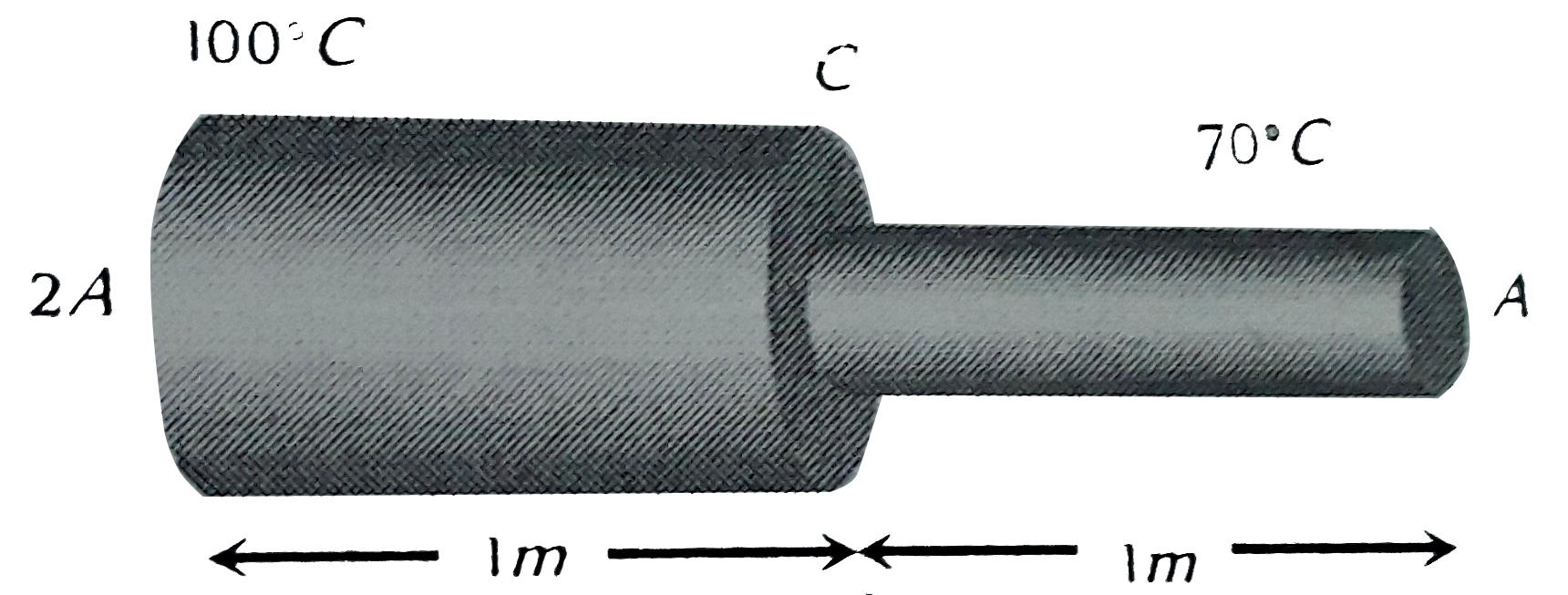 View Solution
View SolutionA uniform wire of length 6m and a cross-sectional area 1.2 cm2 is stretched by a force of 600N . If the Young's modulus of the material of the wire 20×1010Nm−2 , calculate (i) stress (ii) strain and (iii) increase in length of the wire.
View SolutionA wire 2 mm in diameter is just stretched in between two fixed points at a temperature of 50∘C. Calculate the tension in the wire, when the temperature falls to 30∘C. Coefficient of linear expansion is 11×10−4l∘C and Young modulus is 2.1×1011Nm2
View SolutionA steel wire of length 1m and diameter 0.8 mm is kept stretched between two rigid supports. What additional stretching force the wire will experience when its temperature falls by 30K? Linear expansivity of steel α=12×10−6/k . Young's modulus of steel Y=20×1010N/m2.
View SolutionA steel rod of length 25cm has a cross-sectional area of 0.8cm2 . The force required to stretch this rod by the same amount as the expansion produced by heating it through 10∘C is (αsteel=10−5/∘C and Ysteel=2×1010N/m2)
View Solution

