Air is initially at 260∘C and 700pa and occupied 0.028m3. The air is expanded at constant pressure to 0.084m3. A polytropic process with n=1.5 is then carried out followed by a constant temperature process which complete the cycle.
(a) sketch cycle in P−V.
(b) find heat received and heat rejected in the cycle.
(c) efficiency of cycle.
P1=700Pa,V2=0.08m3
P1V1=nRT1
No.of moles v=700×0.0288.31×533=0.004mole
T2T1=V2V1=3
T2=3T1=1599K
PVn=C
P(nRTP)n=C
⇒P=C'Tnn−1
P2P3=(1599533)1.5/0.5=33=27.
Q1−2=vCp(T2−T1)=0.004×72×8.31(1599−533)=124J.
Q2−3=ΔU+∫PdV
=vCv(T3−T2)+nR(T2−T3)n−1=vCv(n−γn−1)(T3−T2)
=0.004×52×8.310.10.5(−1066)=−17.7J.
Q31=W31=vRT1ln(V1V3)=0.004×8.31×533ln(P3P1)=−58.4J
(ΣQ)cycle=(Σw)cycle
ΣW=Q1−2+Q2−3+Q3−1=124−17.7−58.4=47.9J.
ηcycle=WnetHeat supplied=47.9106×100=38.6%

Topper's Solved these Questions
Similar Questions
The density (ρ) versus pressure (p) graph of one mole of an ideal monoatomic gas undergoing a cyclic process is shown in figure. Molecular mass of gas is M.
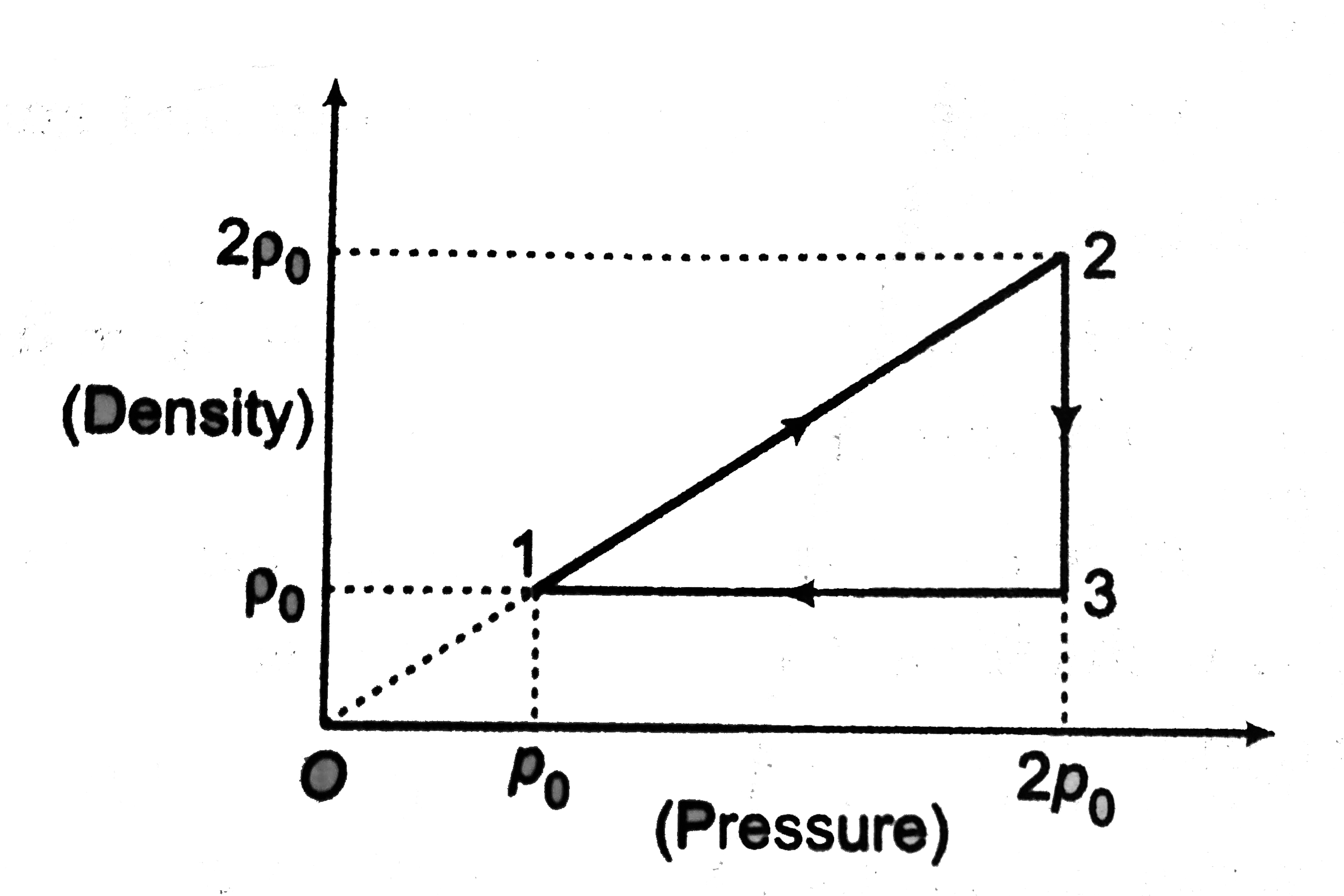
(a) Find work done in each process.
(b) Find heat rejected by gas in one complete cycle.
(c) Find the efficiency of the cycle.
View SolutionThe density (ρ) versus pressure (p) graph of one mole of an ideal monoatomic gas undergoing a cyclic process is shown in figure. Molecular mass of gas is M.
(a) Find work done in each process.
(b) Find heat rejected by gas in one complete cycle.
(c) Find the efficiency of the cycle.

View Solution
Knowledge Check
In a heat engine, the temperature of the source and sink are 500 K and 375 K. If the engine consumes 25×105J per cycle, find(a) the efficiency of the engine, (b) work done per cycle, and (c) heat rejected to the sink per cycle.
A6.25×105JB3×105JC2.19×105JD4×104J
The pressure versus volume graph of one mole of an ideal monatomic gas undergoing a cyclic process is shown in figure. The molecular mass of the gas is M.
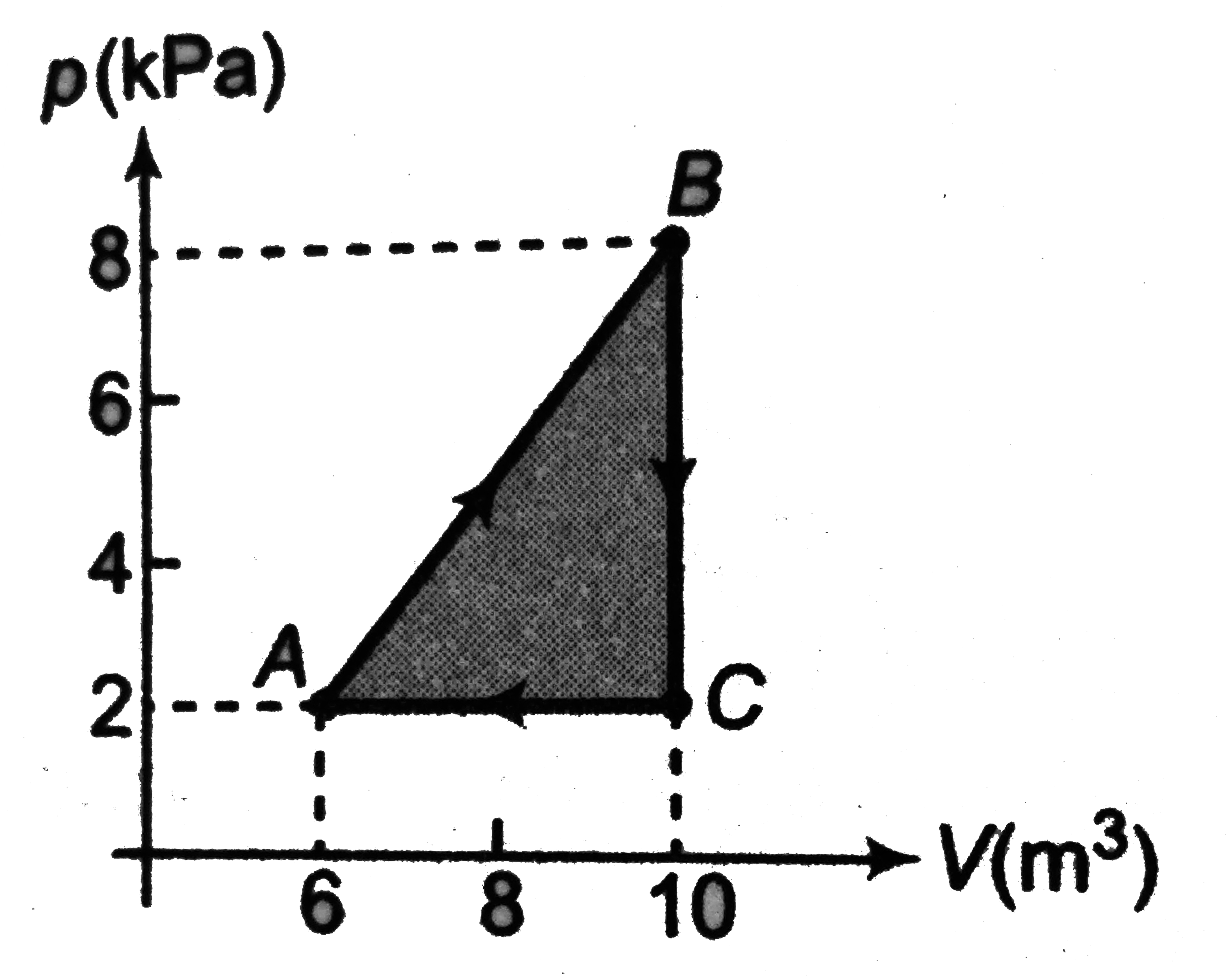
(a) Find the work done in each process.
(b) Find heat rejected by gas in one complete cycle.
(c) Find the effiency of the cycle.
View SolutionOne mole of a mono atomic gas of molar mass M undergoes a cyclic process as shown in the figure. Here ρ is density and P is pressure of the gas.
(a) Calculate the heat rejected by the gas in one complete cycle.
(b) Find the efficiency of the cycle.
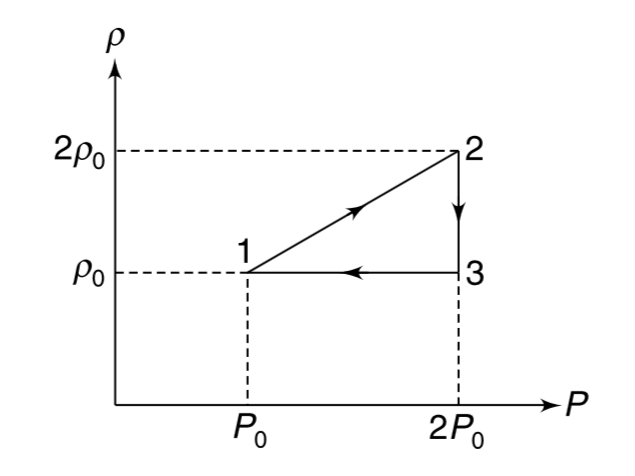
View SolutionAn ideal monoatomic gas occupies volume 10−3m3 at temperature 3K and pressure 103Pa. The internal energy of the gas is taken to be zero at this point. It undergoes the following cycle: The temperature is raised to 300K at constant volume, the gas is then expanded adiabatically till the temperature is 3K , followed by isothermal compression to the original volume . Plot the process on a PV diagram. Calculate (i) The work done and the heat transferred in each process and the internal energy at the end of each process, (ii) The thermal efficiency of the cycle.
View SolutionAn ideal monoatomic gas occupies volume 10−3m3 at temperature 3K and pressure 103Pa. The internal energy of the gas is taken to be zero at this point. It undergoes the following cycle: The temperature is raised to 300K at constant volume, the gas is then expanded adiabatically till the temperature is 3K , followed by isothermal compression to the original volume . Plot the process on a PV diagram. Calculate (i) The work done and the heat transferred in each process and the internal energy at the end of each process, (ii) The thermal efficiency of the cycle.
View SolutionIn a heat engine, the temperature of the source and sink are 500 K and 375 K. If the engine consumes 25×105J per cycle, find(a) the efficiency of the engine, (b) work done per cycle, and (c) heat rejected to the sink per cycle.
View SolutionIn a heat engine, the temperature of the source and sink are 500 K and 375 K. If the engine consumes 25×105J per cycle, find(a) the efficiency of the engine, (b) work done per cycle, and (c) heat rejected to the sink per cycle.
View SolutionAn ideal gas with a known γ, completes a cycle consisting of two isotherms and two isobars. The isothermal processes are executed at temperatures T and T'(<T) and isobaric processes are completed at pressures P0 and eP0 [e = base of natural logarithm]. Find the efficiency of the cycle.
View Solution
