Let V0 be the potential at the origin in an electric field →E=Exˆi+Eyˆj. The potential at the point (x,y) is
V∘+xEx+yEy
V∘+xEx−yEy
V∘−xEx−yEy
√(x2+y2)√(E2x+E2y)−V∘
The correct Answer is:C
To find the potential at the point (x,y) in an electric field given by →E=Ex^i+Ey^j, we can follow these steps:
1. Understand the Relationship Between Electric Field and Potential:
The electric potential V at a point in an electric field is related to the electric field →E by the equation:
VP−V0=−∫P0→E⋅d→r
where VP is the potential at point P, V0 is the potential at the origin, and d→r is the differential displacement vector.
2. Define the Position Vector:
The position vector of point P can be expressed as:
→r=x^i+y^j
3. Calculate the Change in Potential:
The change in potential from the origin to point P can be expressed as:
VP−V0=−∫P0→E⋅d→r
Here, we can express →E as:
→E=Ex^i+Ey^j
4. Evaluate the Dot Product:
The differential displacement d→r can be expressed as:
d→r=dx^i+dy^j
Therefore, the dot product →E⋅d→r becomes:
→E⋅d→r=(Ex^i+Ey^j)⋅(dx^i+dy^j)=Exdx+Eydy
5. Integrate to Find the Potential:
Now we can integrate:
VP−V0=−∫P0(Exdx+Eydy)
This can be separated into two integrals:
VP−V0=−(∫x0Exdx+∫y0Eydy)
6. Evaluate the Integrals:
Assuming Ex and Ey are constant (or can be treated as such over the path), we can evaluate the integrals:
VP−V0=−(Exx+Eyy)
7. Express the Potential at Point P:
Rearranging gives us the potential at point P:
VP=V0−(Exx+Eyy)
Final Answer
Thus, the potential at the point (x,y) is:
VP=V0−(Exx+Eyy)
The electric potential V at a point in an electric field is related to the electric field →E by the equation:
VP−V0=−∫P0→E⋅d→r
where VP is the potential at point P, V0 is the potential at the origin, and d→r is the differential displacement vector.
The position vector of point P can be expressed as:
→r=x^i+y^j
The change in potential from the origin to point P can be expressed as:
VP−V0=−∫P0→E⋅d→r
Here, we can express →E as:
→E=Ex^i+Ey^j
The differential displacement d→r can be expressed as:
d→r=dx^i+dy^j
Therefore, the dot product →E⋅d→r becomes:
→E⋅d→r=(Ex^i+Ey^j)⋅(dx^i+dy^j)=Exdx+Eydy
Now we can integrate:
VP−V0=−∫P0(Exdx+Eydy)
This can be separated into two integrals:
VP−V0=−(∫x0Exdx+∫y0Eydy)
Assuming Ex and Ey are constant (or can be treated as such over the path), we can evaluate the integrals:
VP−V0=−(Exx+Eyy)
Rearranging gives us the potential at point P:
VP=V0−(Exx+Eyy)
Thus, the potential at the point (x,y) is:
VP=V0−(Exx+Eyy)
Similar Questions
Knowledge Check
The potential field of an electric field →E=(yˆi+xˆj) is
AV = -(x+y) + constantBV = constantCV=−(x2+y2)+ constantDV = -xy + constant
Find the potential V of an electrostatic field →E=a(yˆi+xˆj), where a is a constant.
View SolutionA uniform field of 8 N/C exists in space in positive x-direction.
(a) Taking the potential at the origin to be zero, write an expression for the potential at a general point (x, y, z).
(b) At which points, the potential is 160 V ?
(c) It the potential at the origin is taken to be 80V, what will be the expression for the potential at a general point ?
(d) What will be the potential at the origin if the potential at x = infinity is taken to be zero ?
View SolutionThe electric potential V(z,y,z) for a planar charge distribution is given by:
V(x,y,z)=⎧⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪ ⎪ ⎪ ⎪⎩0for x<−d−V0(1+xd)2for −d≤x≤0−V0(1+2xd)for 0≤x<d−3V0for x>d
where −V0 is the potential at the origin and d is a distance. Graph of electric field as a function of position is given as
View SolutionElectric dipole of moment →p=pˆi is kept at a point (x,y) in an electric field →E=4xy2ˆi+4x2yˆj. Find the force on the dipole.
View SolutionAn electric field E=(20ˆi+30ˆj) N/C exists in the space. If the potential at the origin is taken be zero, find the potential at (2m,2m).
View SolutionAn electric field E=(20ˆi+30ˆj) N/C exists in the space. If thepotential at the origin is taken be zero, find the potential at (2m,2m).
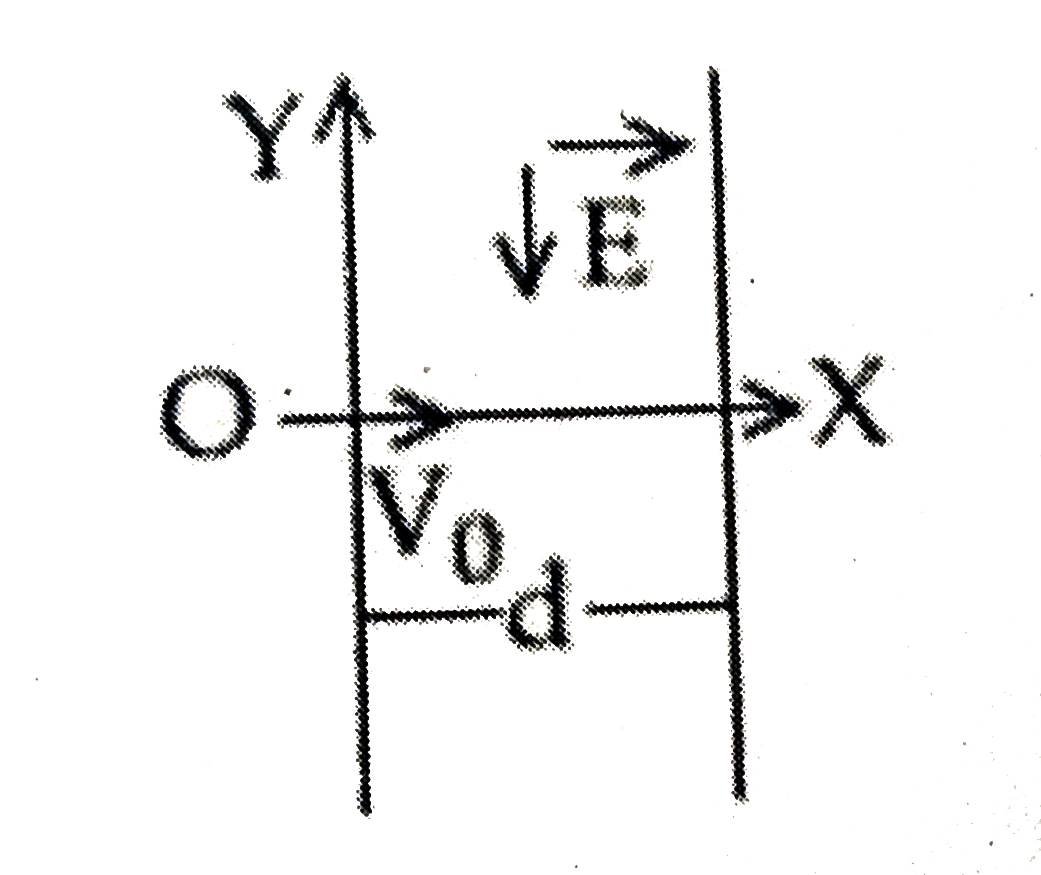
View SolutionA charged particle ( mass m and charge q) moves along X axis with velocity V0 . When it passes through the origin it enters a region having uniform electric field →E=−Eˆj which extends upto x=d . Equation of path of electron in the region . x>d is :
View Solution
