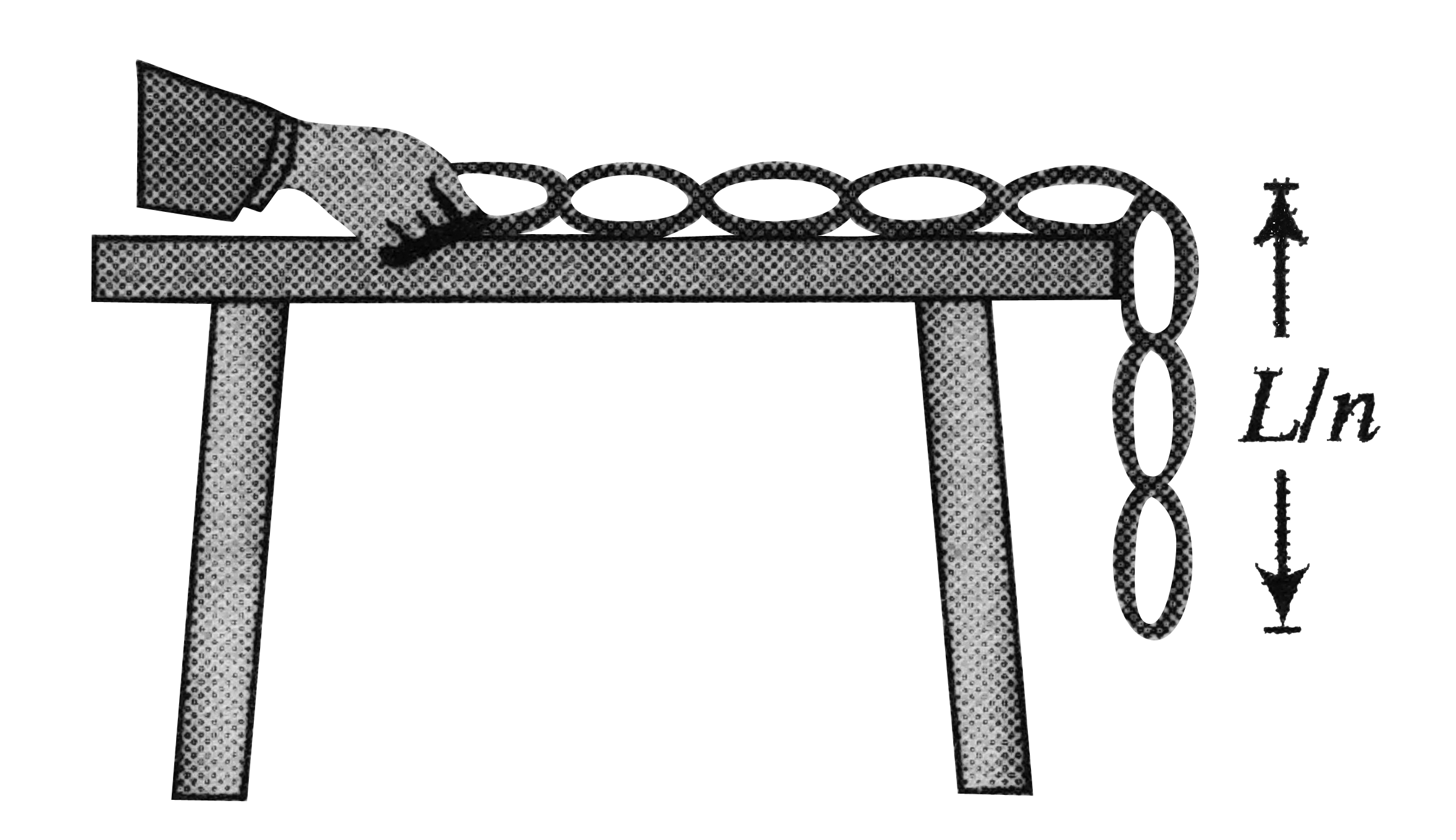
A chain is lying on a rough table with a fraction 1/n of its length hanging down from the edge of the table. if it is just on the point of sliding down from the table, then the coefficient of friction between the table and the chain is -

$\frac{1}{3}$

$\frac{1}{n - 1}$

$\frac{1}{4}$

$\frac{1}{5}$

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem, we need to analyze the forces acting on the chain that is partially hanging off the edge of a rough table. Let's break down the solution step by step.
1. Define the Variables:
- Let the total length of the chain be $L$ .
- The length of the chain hanging down from the table is $\frac{L}{n}$ .
- Therefore, the length of the chain on the table is $L - \frac{L}{n} = L (1 - \frac{1}{n})$ .
2. Calculate the Mass:
- Assume the mass per unit length of the chain is $λ$ .
- The total mass of the chain is $m = λ L$ .
- The mass of the portion hanging down is $m_{h} = λ \cdot \frac{L}{n} = \frac{m}{n}$ .
- The mass of the portion on the table is $m_{t} = m - m_{h} = m - \frac{m}{n} = m (1 - \frac{1}{n})$ .
3. Identify Forces:
- The weight of the hanging part of the chain is $W_{h} = m_{h} g = \frac{m}{n} g$ .
- The normal force $N$ acting on the chain on the table is equal to the weight of the portion on the table, which is $N = m_{t} g = m (1 - \frac{1}{n}) g$ .
4. Friction Force:
- The maximum static friction force $F_{f}$ that can act on the chain is given by:
$F_{f} = μ N = μ m (1 - \frac{1}{n}) g$
5. Condition for Sliding:
- The chain is just about to slide when the weight of the hanging part equals the maximum static friction force:
$W_{h} = F_{f}$
- Substituting the expressions we derived:
$\frac{m}{n} g = μ m (1 - \frac{1}{n}) g$
6. Canceling Common Terms:
- We can cancel $m g$ from both sides (assuming $g \neq 0$ ):
$\frac{1}{n} = μ (1 - \frac{1}{n})$
7. Solving for Coefficient of Friction $μ$ :
- Rearranging the equation gives:
$μ = \frac{1 / n}{1 - 1 / n} = \frac{1 / n}{(n - 1) / n} = \frac{1}{n - 1}$
8. Final Result:
- Therefore, the coefficient of friction $μ$ between the table and the chain is:
$μ = \frac{1}{n - 1}$
Final Answer:
The coefficient of friction between the table and the chain is $\frac{1}{n - 1}$ .

Updated on:18/9/2024

A uniform chain of length $L$ and mass $M$ is lying on a smooth table and one-third of its length is hanging vertically down over the edge of the table. If g is the acceleration due to gravity, the work required to pull the hanging part on to the table is
AMgL
B $\frac{M g L}{3}$
C $\frac{M g L}{9}$
D $\frac{M g L}{18}$