Let S be an imaginary closed surface enclosing mass m. Let d→S be an element of area on S, the direction of d→S being outward from S. Let →E be the gravitational intensity at d→S. We define ϕ=∮sE⋅dS, the integration being carried out over the entire surface S.
ϕ=−Gm
ϕ=−4πGm
ϕ=−Gm4π
No relation of the type (a) , (b) or (c) can exist
The correct Answer is:B
To solve the problem, we need to calculate the gravitational flux ϕ through the closed surface S that encloses a mass m. The gravitational intensity →E at a distance r from the mass m can be expressed using Newton's law of gravitation.
1. Understanding Gravitational Intensity:
The gravitational intensity →E at a distance r from a mass m is given by:
→E=−Gmr2^r
where G is the gravitational constant and ^r is the unit vector pointing radially outward from the mass.
2. Defining the Area Element:
The area element d→S on the closed surface S is directed outward, and its magnitude is dS. Thus, we can write:
d→S=dS^n
where ^n is the outward unit normal vector.
3. Calculating the Gravitational Flux:
The gravitational flux ϕ through the surface S is defined as:
ϕ=∮S→E⋅d→S
Substituting the expressions for →E and d→S:
ϕ=∮S(−Gmr2^r)⋅(dS^n)
4. Considering Symmetry:
For a symmetric surface (like a sphere), at any point on the surface, the direction of ^n is the same as ^r. Thus, we can simplify the dot product:
ϕ=−Gmr2∮SdS
5. Calculating the Total Area:
The total surface area A of a sphere of radius r is:
A=4πr2
Therefore, substituting this into the flux equation:
ϕ=−Gmr2⋅4πr2
6. Final Expression for Flux:
Simplifying the expression gives:
ϕ=−4πGm
Conclusion:
The gravitational flux ϕ through the closed surface S is:
ϕ=−4πGm
The gravitational intensity →E at a distance r from a mass m is given by:
→E=−Gmr2^r
where G is the gravitational constant and ^r is the unit vector pointing radially outward from the mass.
The area element d→S on the closed surface S is directed outward, and its magnitude is dS. Thus, we can write:
d→S=dS^n
where ^n is the outward unit normal vector.
The gravitational flux ϕ through the surface S is defined as:
ϕ=∮S→E⋅d→S
Substituting the expressions for →E and d→S:
ϕ=∮S(−Gmr2^r)⋅(dS^n)
For a symmetric surface (like a sphere), at any point on the surface, the direction of ^n is the same as ^r. Thus, we can simplify the dot product:
ϕ=−Gmr2∮SdS
The total surface area A of a sphere of radius r is:
A=4πr2
Therefore, substituting this into the flux equation:
ϕ=−Gmr2⋅4πr2
Simplifying the expression gives:
ϕ=−4πGm
The gravitational flux ϕ through the closed surface S is:
ϕ=−4πGm
Similar Questions
A satellite of mass m is revolving around the Earth at a height R above the surface of the Earth. If g is the gravitational intensity at the Earth’s surface and R is the radius of the Earth, then the kinetic energy of the satellite will be:
View SolutionA satellite of mass m is revolving around the Earth at a height R above the surface of the Earth. If g is the gravitational intensity at the Earth’s surface and R is the radius of the Earth, then the kinetic energy of the satellite will be:
View Solution
Knowledge Check
- Question 1 - Select One
If ∮sE.ds=0 Over a surface, then
Athe electric field inside the surface and on it is zero.Bthe electric field inside the surface is necessarily uniform.Call charges must necessarily be outside the surface.Dall of these.
If charge q1 and q2 lies inside and outside respectively of a closed surface S. Let E be the electric field at any point on S and ϕ be the electric flux over S. Select the incorrect option.
View SolutionCharges Q1andQ2 lie inside and outside, respectively, of a closed surface S. Let E be the field at any point on S and ϕ be the flux of E over S.
View SolutionCharges Q1andQ2 lie inside and outside, respectively, of a closed surface S. Let E be the field at any point on S and ϕ be the flux of E over S.
View SolutionLet S be the set of all triangles in the xy-plane, each having one vertex at the origin and the other two vertices lie on coordinate axes with integral coordinates. If each triangle in S has area 50 eq. units, then the number of elements in the set S is
View SolutionIf ∮sE.ds=0 Over a surface, then
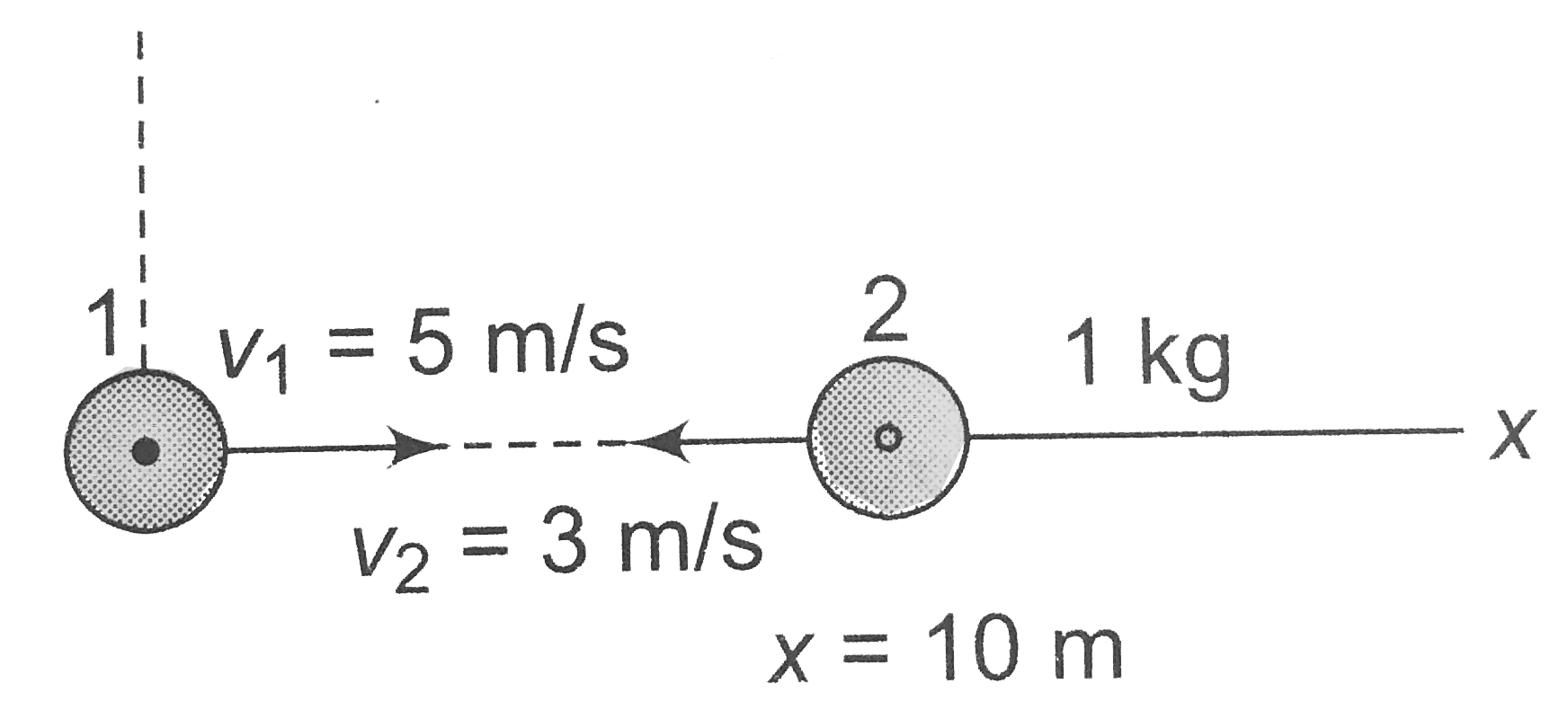
View SolutionAt t=0, the positions and velocities of two particles are as shown in the figure. They are kept on a smooth surface and being mutually attracted by gravitational force. Find the position of centre of mass at t=2s.
 .View Solution
.View SolutionAt t=0, the positions and velocities of two particles are as shown in the figure. They are kept on a smooth surface and being mutually attracted by gravitational force. Find the position of centre of mass at t=2s.
 .View Solution
.View Solution

