Using the formula for the moment of inertia of a uniform sphere, find the moment of inertia of a thin spherical layer of mass m and radius R relative to the axis passing through its centre.
MR2
MR2/2
2/3MR2
2/5MR2
The correct Answer is:C
To find the moment of inertia of a thin spherical layer of mass m and radius R relative to the axis passing through its center, we can follow these steps:
1. Understanding the Moment of Inertia of a Sphere:
The moment of inertia I of a solid uniform sphere about an axis through its center is given by the formula:
I=25MR2
where M is the mass of the sphere and R is its radius.
2. Consider a Thin Spherical Layer:
We consider a thin spherical layer of thickness dr at radius r from the center of the sphere. The total mass of this thin layer is m.
3. Calculate the Volume of the Thin Layer:
The volume dV of the thin spherical layer can be calculated using the formula for the volume of a sphere:
dV=Surface Area×Thickness=4πr2⋅dr
4. Relate Mass to Density:
The mass m of the thin layer can be expressed in terms of its density ρ:
m=ρ⋅dV=ρ⋅(4πr2dr)
5. Express Density in Terms of Mass:
Rearranging the equation gives:
ρ=m4πr2dr
6. Moment of Inertia of the Thin Layer:
The moment of inertia I′ of the thin spherical layer can be derived from the moment of inertia of a sphere of radius r+dr minus the moment of inertia of a sphere of radius r:
I′=I(r+dr)−I(r)
Using the formula for the moment of inertia of a sphere, we get:
I(r+dr)=25M(r+dr)2
I(r)=25Mr2
7. Expanding the Moment of Inertia:
Expanding I(r+dr) using the binomial expansion:
I(r+dr)=25M(r2+2r⋅dr+(dr)2)
Thus,
I′=25M(r2+2r⋅dr+(dr)2)−25Mr2
Simplifying gives:
I′=25M(2r⋅dr+(dr)2)
8. Neglecting Higher Order Terms:
Since dr is very small, we can neglect (dr)2:
I′≈25M(2r⋅dr)=45Mr⋅dr
9. Final Expression:
Therefore, the moment of inertia of the thin spherical layer is:
I′=45mr2
The moment of inertia I of a solid uniform sphere about an axis through its center is given by the formula:
I=25MR2
where M is the mass of the sphere and R is its radius.
We consider a thin spherical layer of thickness dr at radius r from the center of the sphere. The total mass of this thin layer is m.
The volume dV of the thin spherical layer can be calculated using the formula for the volume of a sphere:
dV=Surface Area×Thickness=4πr2⋅dr
The mass m of the thin layer can be expressed in terms of its density ρ:
m=ρ⋅dV=ρ⋅(4πr2dr)
Rearranging the equation gives:
ρ=m4πr2dr
The moment of inertia I′ of the thin spherical layer can be derived from the moment of inertia of a sphere of radius r+dr minus the moment of inertia of a sphere of radius r:
I′=I(r+dr)−I(r)
Using the formula for the moment of inertia of a sphere, we get:
I(r+dr)=25M(r+dr)2
I(r)=25Mr2
Expanding I(r+dr) using the binomial expansion:
I(r+dr)=25M(r2+2r⋅dr+(dr)2)
Thus,
I′=25M(r2+2r⋅dr+(dr)2)−25Mr2
Simplifying gives:
I′=25M(2r⋅dr+(dr)2)
Since dr is very small, we can neglect (dr)2:
I′≈25M(2r⋅dr)=45Mr⋅dr
Therefore, the moment of inertia of the thin spherical layer is:
I′=45mr2
Similar Questions
Find the moment of inertia of a pair of spheres, each having a mass m and radius r kept in contact about the tangent passing through the point of contact.
View SolutionFind the moment of inertia of a uniform ring of mass M and radius R about a diameter.
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
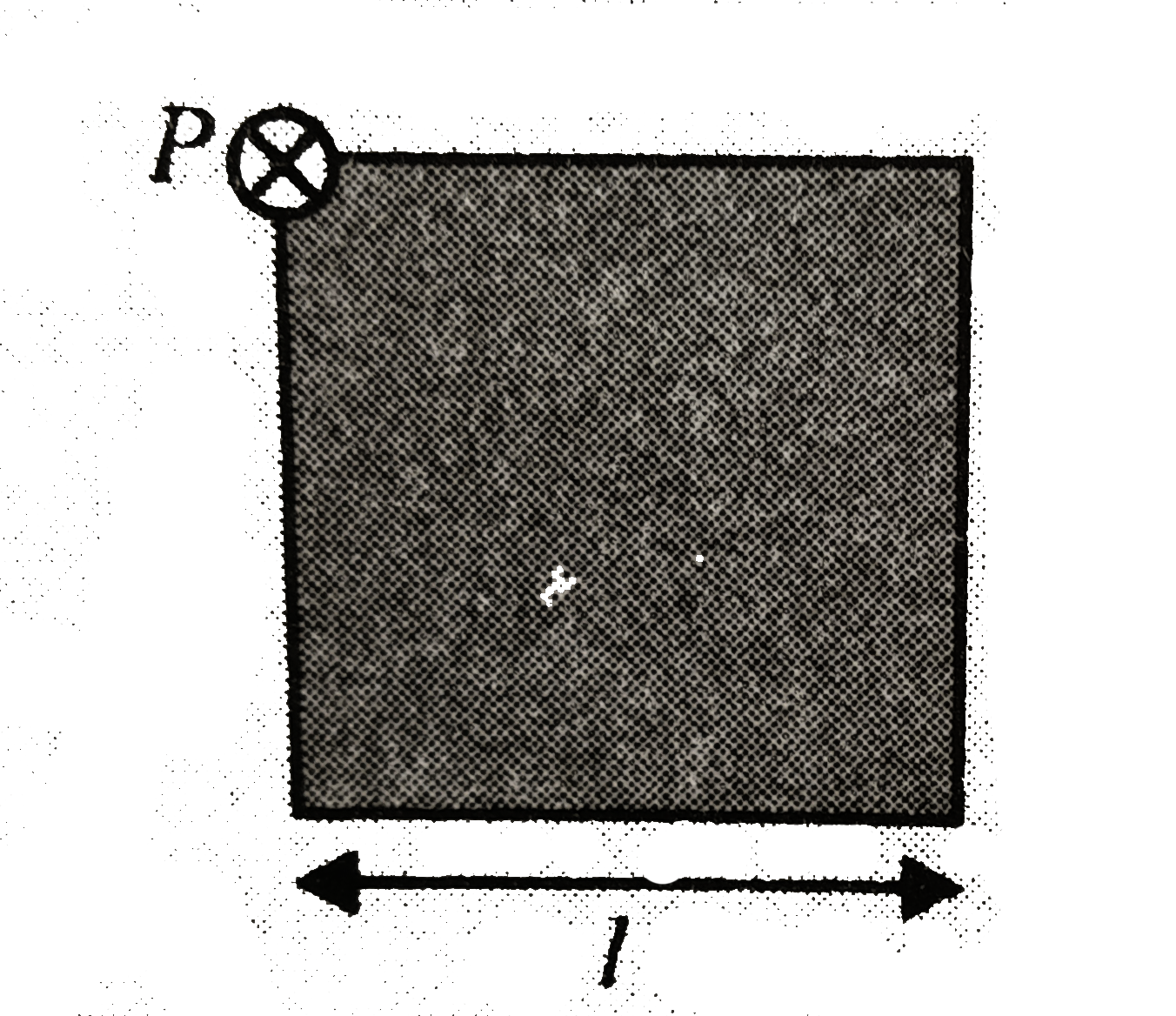
View SolutionFind the moment of inertia of a uniform square plate of mass M and edge of length 'l' about its axis passing through P and perpendicular to it.
View SolutionThe moment of inertia of a spherical shell and a hemispherical shell of same mass and radius about a tangential axis, passing through P will be

View SolutionFind the moment of inertia of a uniform sphere of mass m and radius R about a tangent if the spheres (1) solid (ii) hollow?
View SolutionThe moment of inertia of thin uniform circular disc about one of the diameter is I. Its moment of inertia about an axis perpendicular to the circular surface and passing through its centre is
View SolutionThe curve for the moment of inertia of a sphere of constant mass M versus its radius will be:
View SolutionWhy does a solid sphere have smaller moment of inertia than a hollow cylinder of same mass and radius, about an axis passing through their axes of symmentry ?
View SolutionFind the moment of inertia of a uniform rectangular plate of mass M and edges of length 'I' and 'b' about its axis passing through the centre and perpendicular to it.
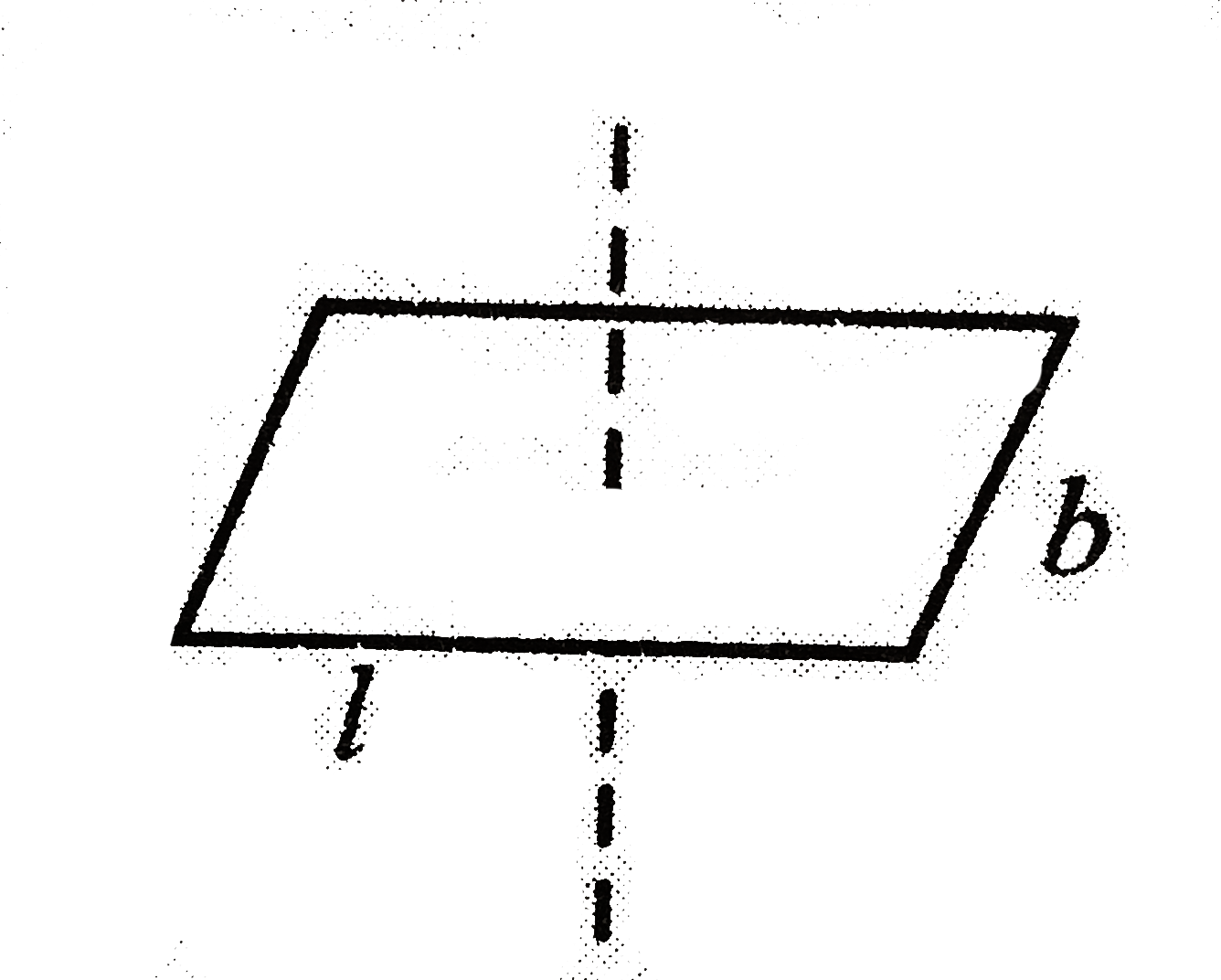
View Solution
