An open rail road car of mass M is moving with initial velocity v0 along a straight horizontla and friction less track.It suddenly starts raining at time t=0. The rain drops fall vertically with velocity with velocity u and add a mass of m kg/sec of water . The velocity of car after t second is
M+mMV0t
MV0tM+v
Mv0M+mt
M(M+m)v0
The correct Answer is:C
To solve the problem, we will use the principle of conservation of momentum. Here's a step-by-step breakdown of the solution:
Step 1: Understand the Initial Conditions
At time t=0:
- The mass of the railroad car is M.
- The initial velocity of the car is v0.
- The initial momentum of the system (car) is given by:
Initial Momentum=M⋅v0
Step 2: Analyze the Situation After Time t
After time t:
- The rain adds mass to the car at a rate of m kg/s.
- The total mass added by the rain after time t is m⋅t.
- Therefore, the total mass of the car plus the rain after time t is:
Total Mass=M+m⋅t
Step 3: Apply the Conservation of Momentum
Since there are no external horizontal forces acting on the system, we can apply the conservation of momentum:
- The initial momentum of the system (car) is M⋅v0.
- The final momentum of the system after time t is given by the total mass multiplied by the final velocity v:
Final Momentum=(M+m⋅t)⋅v
Setting the initial momentum equal to the final momentum:
M⋅v0=(M+m⋅t)⋅v
Step 4: Solve for the Final Velocity v
Rearranging the equation to solve for v:
v=M⋅v0M+m⋅t
Conclusion
The velocity of the car after time t is:
v=M⋅v0M+m⋅t
At time t=0:
- The mass of the railroad car is M.
- The initial velocity of the car is v0.
- The initial momentum of the system (car) is given by:
Initial Momentum=M⋅v0
After time t:
- The rain adds mass to the car at a rate of m kg/s.
- The total mass added by the rain after time t is m⋅t.
- Therefore, the total mass of the car plus the rain after time t is:
Total Mass=M+m⋅t
Since there are no external horizontal forces acting on the system, we can apply the conservation of momentum:
- The initial momentum of the system (car) is M⋅v0.
- The final momentum of the system after time t is given by the total mass multiplied by the final velocity v:
Final Momentum=(M+m⋅t)⋅v
M⋅v0=(M+m⋅t)⋅v
Rearranging the equation to solve for v:
v=M⋅v0M+m⋅t
The velocity of the car after time t is:
v=M⋅v0M+m⋅t
Similar Questions
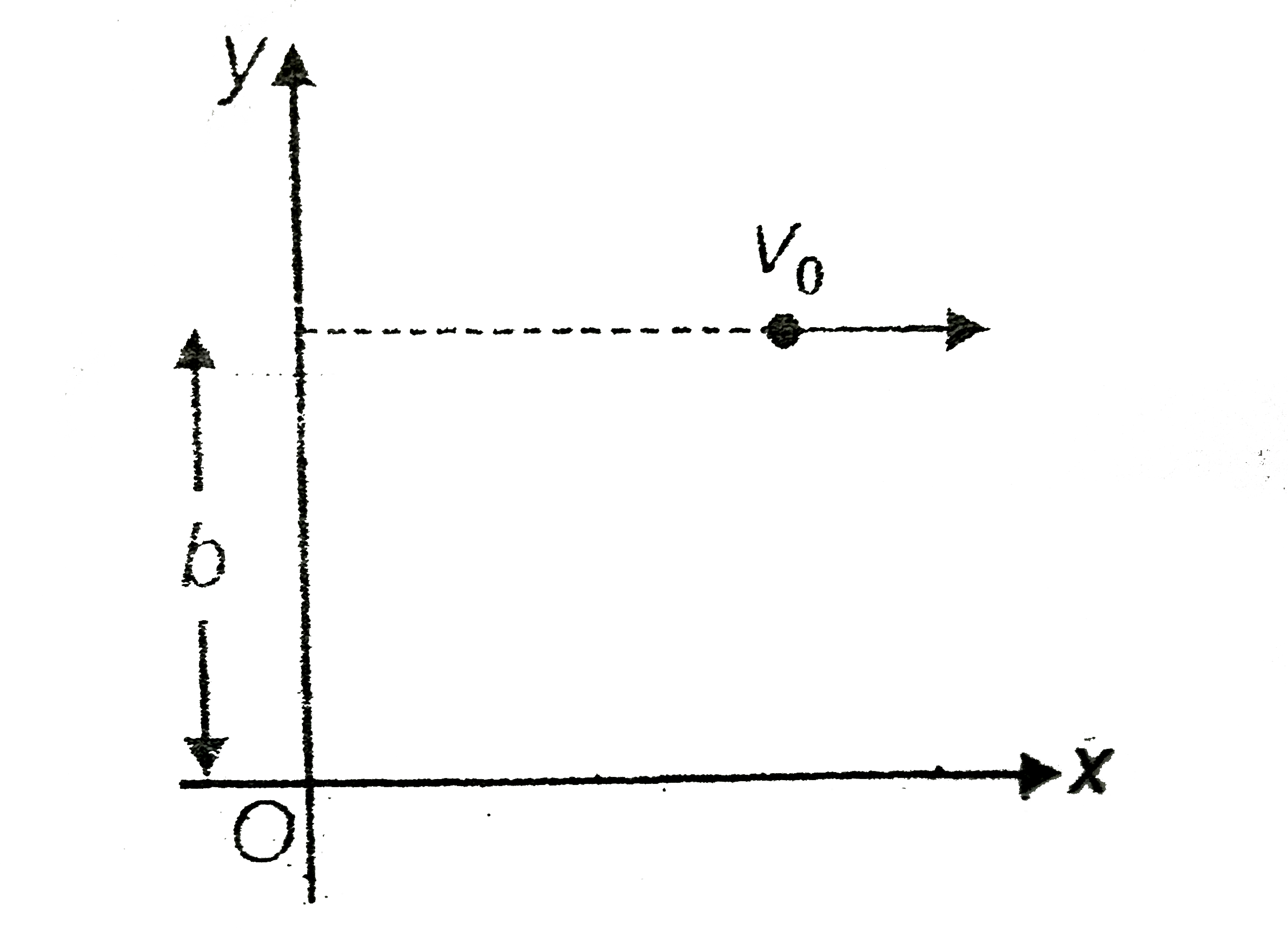
A particle of mass m is moving with constant velocity v0 along the line y=b. At time t=0 it was at the point (0,b). At time t=bv0, the
 View Solution
View SolutionAn open water tight railway wagon of mass 5×103kg moves at an initial velocity 1.2m/s without friction on a railway track. Rain drops fall vertically downwards into the wagon. The velocity of the wagon after it has collected103kg of water will be :-
View SolutionA plank of mass m moving with a velocity v along a friction less horizontal track and a body of mass m/2 moving with 2v collides with plank elastically. Final speed of the plank is:
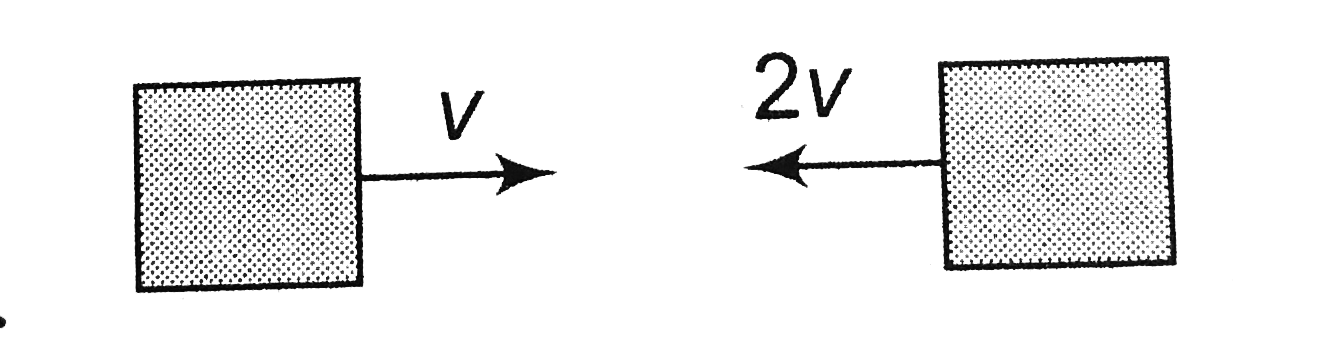 View Solution
View SolutionA body of mass m is thrown upwards at an angle θ with the horizontal with velocity v. While rising up the velocity of the mass after t second will be
View Solutiona. A rail road car of mass M is moving without friction on a straight horizontal track with a velocity ct. A man of mass m lands on it normally from a helicopter. What will be the new velocity of the car? ltbgt b. If now the man begins to run on it with speed um with respect to car in a direction opposite to motion of the car, what will be the new velocity of the car?
View SolutionA particle moves with an initial velocity V0 and retardation αv , where α is a constant and v is the velocity at any time t.
Velocity of particle at time is :View SolutionA car of mass M is moving with a uniform velocity υ on a horizontal road when the hero of a Hindi film drops himself on it from above. Taking the mass of the hero to be m what will be the velocity of the car after the event?
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View Solutiona. A rail road flat car of mass M can roll without friction along a straight horizontal track . Initially, a man of mass m is standing on the car which is moving to the right with speed v0. What is the change in velocity of the car if the man runs to the left so that his speed relative to the car is vrel just before he jumps off at the left end?
b. If there are n men each of mass m on the car, should they all run and jump off together or should they run and jump one by one in order to give a greater velocity to the car?
 View Solution
View Solution

