Consider 3 identical balls, each having mass of 0.1 kg. The ball A has an initial velocity of 10√3m/s. It then collides simultaneously with balls B and C, whose centres are on a line perpendicular to the initial velocity of the ball A. They are initially in contact with each other and are at rest. The ball A is aimed directly at the contact point of B and C. After the collision, if the ball A comes to rest, then
Collision in elastic.
Collision is inelastic and final kinetic energy of the system-Initial kinetic energy of the system = 5J
Collision is inelastic and final kinetic energy of the system - Initial kinetic energy of the system = - 5J
Collision in elastic, but the final kinetic energy of the system is less than the initial kinetic energy of the system.
The correct Answer is:C
To solve the problem step by step, we will analyze the collision of the three identical balls and apply the principles of conservation of momentum and kinetic energy.
Step 1: Understand the Initial Conditions
- We have three identical balls (A, B, and C), each with a mass m=0.1kg.
- Ball A has an initial velocity of vA=10√3m/s.
- Balls B and C are at rest and in contact with each other, positioned such that their centers are on a line perpendicular to the initial velocity of ball A.
Step 2: Analyze the Collision Geometry
- When ball A collides with balls B and C, it is aimed directly at the contact point between B and C.
- The centers of the balls form an equilateral triangle at the moment of collision, with angles of 60∘ between the lines connecting the centers.
- The angles α at which balls B and C will move after the collision are 30∘ each.
Step 3: Apply Conservation of Momentum
- The initial momentum of the system is entirely due to ball A:
pinitial=mvA=0.1×10√3=1√3kg m/s
- After the collision, ball A comes to rest, so its momentum is 0.
- Let the velocities of balls B and C after the collision be v1 (since they are identical). The momentum of balls B and C can be expressed as:
pfinal=mv1cos(30∘)+mv1cos(30∘=2mv1cos(30∘)
- Setting the initial momentum equal to the final momentum:
1√3=2(0.1)v1(√32)
Step 4: Solve for v1
- Simplifying the equation:
1√3=0.1v1√3
- Dividing both sides by √3:
1=0.1v1
- Thus, we find:
v1=10.1=10m/s
Step 5: Calculate Kinetic Energy Before and After Collision
- Initial Kinetic Energy (KE_initial):
KEinitial=12mv2A=12×0.1×(10√3)2=12×0.1×300=15J
- Final Kinetic Energy (KE_final):
KEfinal=12mv21+12mv21=2×12mv21=mv21=0.1×(10)2=10J
Step 6: Determine the Nature of the Collision
- The change in kinetic energy is:
ΔKE=KEfinal−KEinitial=10J−15J=−5J
- Since the kinetic energy after the collision is less than before, the collision is inelastic.
Conclusion
- The collision is inelastic, and the change in kinetic energy is −5J.
- We have three identical balls (A, B, and C), each with a mass m=0.1kg.
- Ball A has an initial velocity of vA=10√3m/s.
- Balls B and C are at rest and in contact with each other, positioned such that their centers are on a line perpendicular to the initial velocity of ball A.
- When ball A collides with balls B and C, it is aimed directly at the contact point between B and C.
- The centers of the balls form an equilateral triangle at the moment of collision, with angles of 60∘ between the lines connecting the centers.
- The angles α at which balls B and C will move after the collision are 30∘ each.
- The initial momentum of the system is entirely due to ball A:
pinitial=mvA=0.1×10√3=1√3kg m/s
- After the collision, ball A comes to rest, so its momentum is 0.
- Let the velocities of balls B and C after the collision be v1 (since they are identical). The momentum of balls B and C can be expressed as:
pfinal=mv1cos(30∘)+mv1cos(30∘=2mv1cos(30∘)
- Setting the initial momentum equal to the final momentum:
1√3=2(0.1)v1(√32)
- Simplifying the equation:
1√3=0.1v1√3
- Dividing both sides by √3:
1=0.1v1
- Thus, we find:
v1=10.1=10m/s
- Initial Kinetic Energy (KE_initial):
KEinitial=12mv2A=12×0.1×(10√3)2=12×0.1×300=15J
KEfinal=12mv21+12mv21=2×12mv21=mv21=0.1×(10)2=10J
- The change in kinetic energy is:
ΔKE=KEfinal−KEinitial=10J−15J=−5J
- Since the kinetic energy after the collision is less than before, the collision is inelastic.
- The collision is inelastic, and the change in kinetic energy is −5J.
Similar Questions
A ball A of mass M collides elastically with a similar ball B at rest as shown in figure. Initially velocity of ball A is u m/s. After collision,
 View Solution
View SolutionA ball thrown vertically upwards with an initial velocity of 1.4m/s. The total displcement of the ball is
View SolutionA ball is thrown vertically upwards. It returns 6 s later. Calculate the initial velocity of the ball. (Take g=10m s−2 )
View SolutionA ball is thrown vertically upwards. It was observed at a height h twice after a time interval Δt. The initial velocity of the ball is
View SolutionThe ball A collides elastically with an identical ball B with a speed v. The ball B touches another identical ball C. The velocity of C, after the impact, is equal to
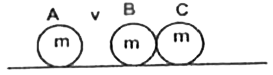 View Solution
View SolutionA heavy ball of mass 2M moving with a velocity v0 collides elastically head on with a cradle of three identical ball each of mass M as shown in figure. Determine the velocity of each ball after collision.
 View Solution
View SolutionThe figure below shows three identical balls A , B and C. Initially , the baiis B and C are at rest and the ball A while moving with a veiocity v, collides with ball B. if the collision is perfectiy elastic , then after the collision
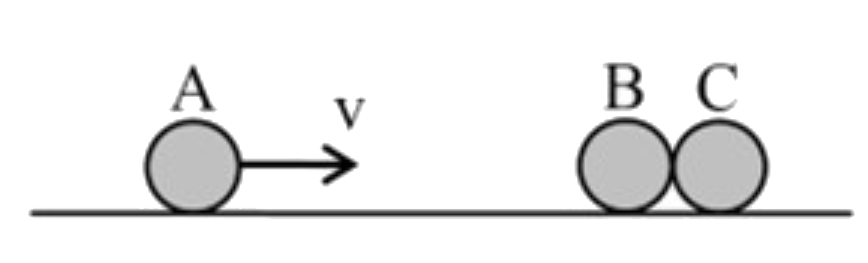 View Solution
View SolutionA ball of mass 10 g is moving with a velocity of 50ms−1. On applying a constant force on ball for 2.0 s, it acquires a velocity of 70ms−1. Calculate the initial momentum of ball
View SolutionBall 1 collides directly with another identical ball 2 at rest. Velocity of second ball becomes two times that of 1 after collison. Find the coefficient of restitution between the two balls?
View SolutionBall 1 collides directly with another identical ball 2 at rest. Velocity of second ball becomes two times that of 1 after collison. Find the coefficient of restitution between the two balls?
View Solution
