STATEMENT-1: In electric circuits, wires carrying currents in opposite directions are often twisted together. because
STATEMENT-2: The magnetic field in the surrounding space of a twisted wire system is not precisely zero because the wires are not in the same location in space.
Statement-1 is True , Statement-2 is True , Statement-2 is a correct explanation for Statement-1.
Statement-1 is True , Statement-2 is True , Statement-2 is NOT a correct explanation for Statement-1.
Statement-1 is True , Statement-2 is False
Statement-1 is False , Statement-2 is True
The correct Answer is:A
To analyze the given statements, we will break down the reasoning step by step.
Step 1: Understanding Statement 1
- Statement 1 states that in electric circuits, wires carrying currents in opposite directions are often twisted together.
- The reason for twisting wires is to minimize the magnetic field generated by the currents.
Hint for Step 1: Consider the effect of current on magnetic fields and how they interact when currents flow in opposite directions.
---
Step 2: Understanding Statement 2
- Statement 2 claims that the magnetic field in the surrounding space of a twisted wire system is not precisely zero because the wires are not in the same location in space.
- When two wires carry currents in opposite directions, they create magnetic fields that can cancel each other out in certain regions.
Hint for Step 2: Think about how magnetic fields from different sources combine and how their locations affect the resultant field.
---
Step 3: Analyzing the Magnetic Field
- When the wires are twisted, the magnetic fields produced by each wire do not completely cancel out in all regions because they are not located at the same point in space.
- The magnetic field due to each wire can be determined using the right-hand rule, and in some regions, the fields will add up instead of canceling out completely.
Hint for Step 3: Use the right-hand rule to visualize the direction of the magnetic fields produced by each wire.
---
Step 4: Conclusion on Statements
- Statement 1 is correct because twisting wires helps to reduce the net magnetic field in the vicinity of the wires.
- Statement 2 is also correct in that the magnetic field is not precisely zero due to the spatial separation of the wires.
- However, Statement 2 does not provide a correct explanation for Statement 1. The reason for twisting the wires is to minimize the magnetic field, not because they are not in the same location.
Hint for Step 4: Reflect on the relationship between the two statements and how one serves as a reason for the other.
---
Final Conclusion:
- Both statements are correct, but Statement 2 is not a correct explanation for Statement 1. Therefore, the correct answer is that Statement 1 is true, and Statement 2 is true but does not explain Statement 1.
- Statement 1 states that in electric circuits, wires carrying currents in opposite directions are often twisted together.
- The reason for twisting wires is to minimize the magnetic field generated by the currents.
- Statement 2 claims that the magnetic field in the surrounding space of a twisted wire system is not precisely zero because the wires are not in the same location in space.
- When two wires carry currents in opposite directions, they create magnetic fields that can cancel each other out in certain regions.
- When the wires are twisted, the magnetic fields produced by each wire do not completely cancel out in all regions because they are not located at the same point in space.
- The magnetic field due to each wire can be determined using the right-hand rule, and in some regions, the fields will add up instead of canceling out completely.
- Statement 1 is correct because twisting wires helps to reduce the net magnetic field in the vicinity of the wires.
- Statement 2 is also correct in that the magnetic field is not precisely zero due to the spatial separation of the wires.
- However, Statement 2 does not provide a correct explanation for Statement 1. The reason for twisting the wires is to minimize the magnetic field, not because they are not in the same location.
- Both statements are correct, but Statement 2 is not a correct explanation for Statement 1. Therefore, the correct answer is that Statement 1 is true, and Statement 2 is true but does not explain Statement 1.
Similar Questions
Two long straight wires, each carrying a current I in opposite directions are separated by a distance R. The magnetic induction at a point mid way between the wire is
View SolutionQuite often, connecting wires carrying currents in opposite directions are twisted together in using electrical appliances. Explain how it avoids unwanted magnetic fields.
View SolutionThe two long, straight wires carrying electric currents in opposite directions. The separation between the wires is 5.0 cm. Find the magnetic field at a point P midway between the wires.
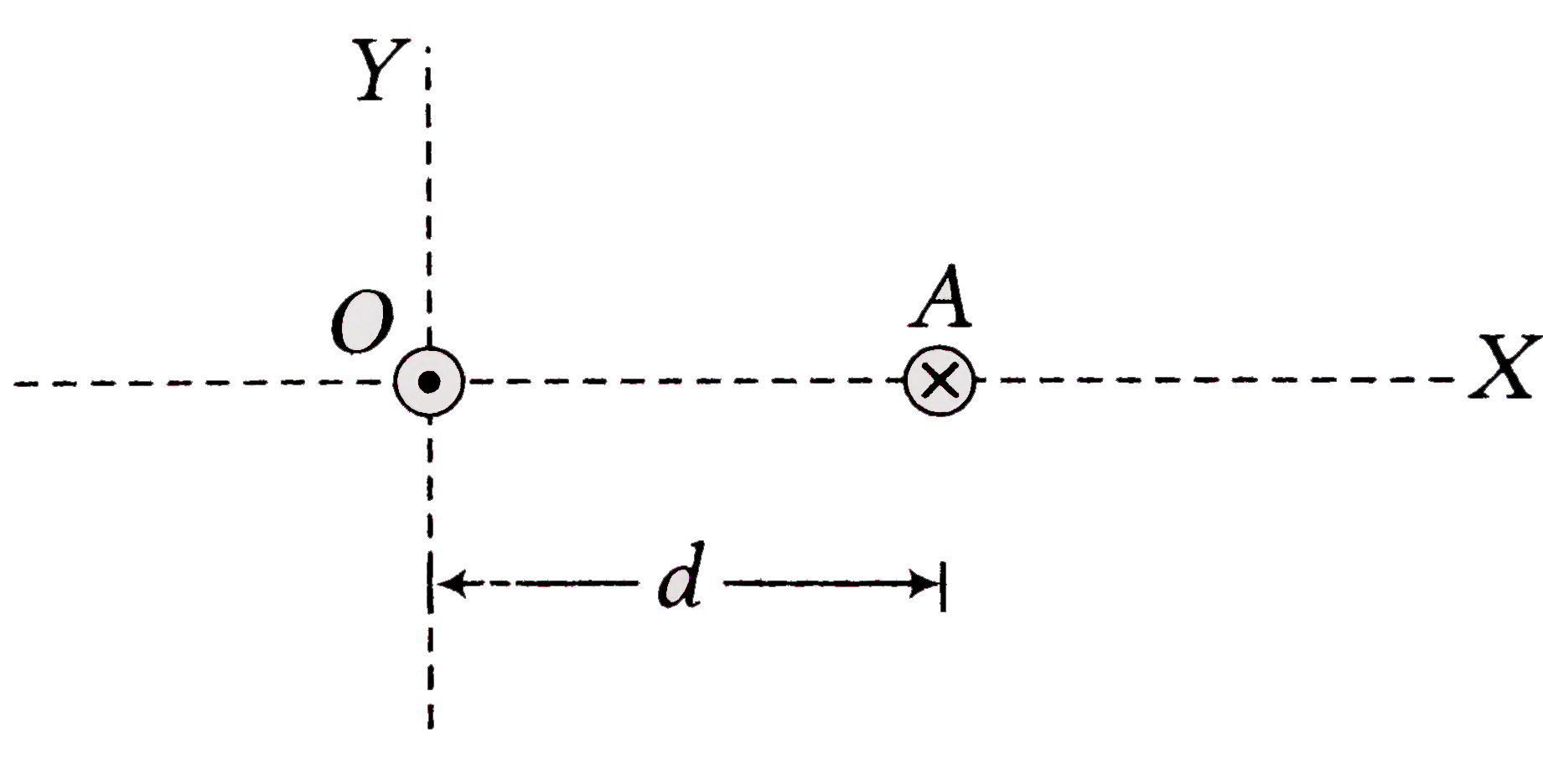
View SolutionTwo long wires carrying same currents in opposite directions are placed at separation D as shown.Predict variation of magnetic field as one moves from the point O and A
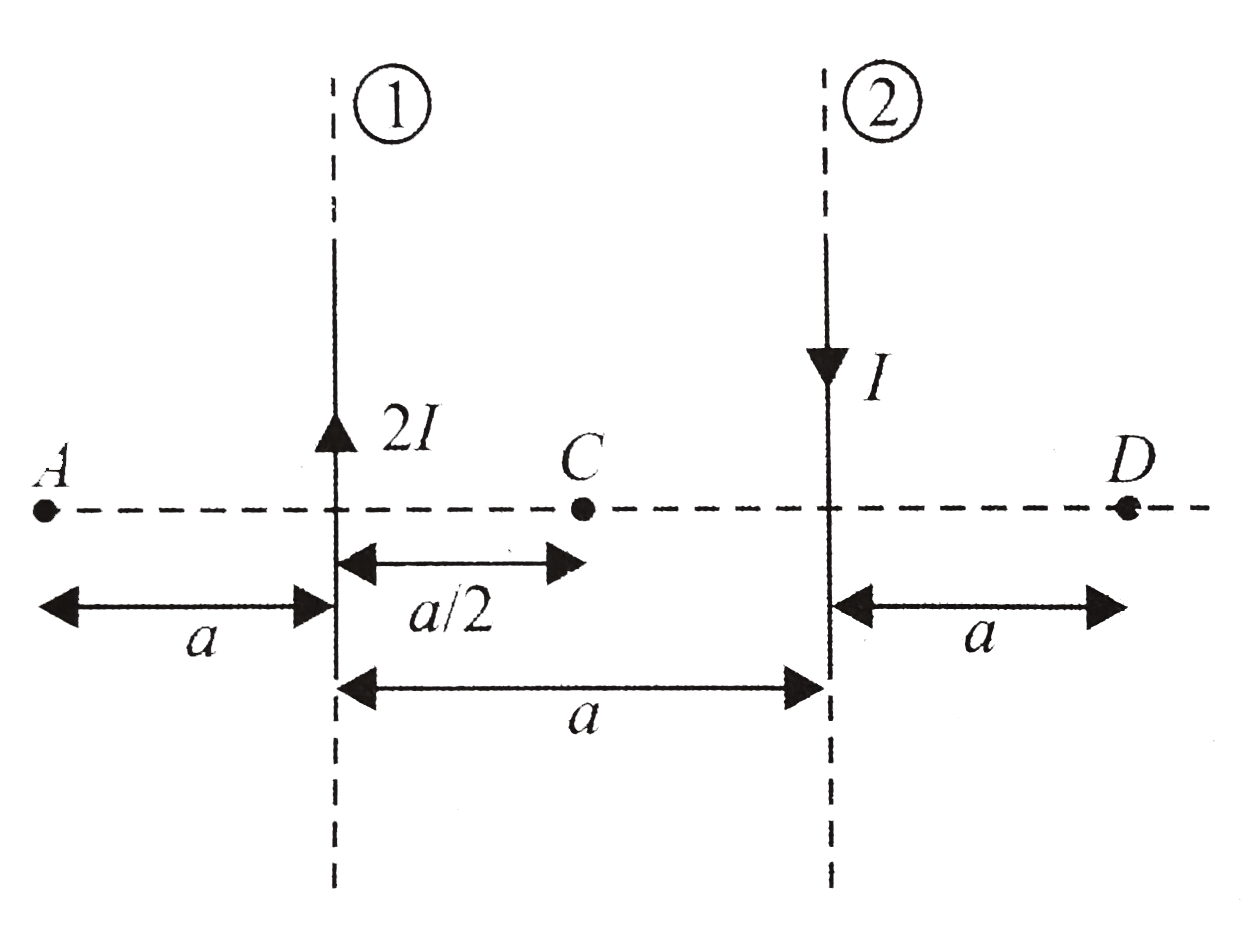
View SolutionFind magnetic field at C by the system of current carrying wire.

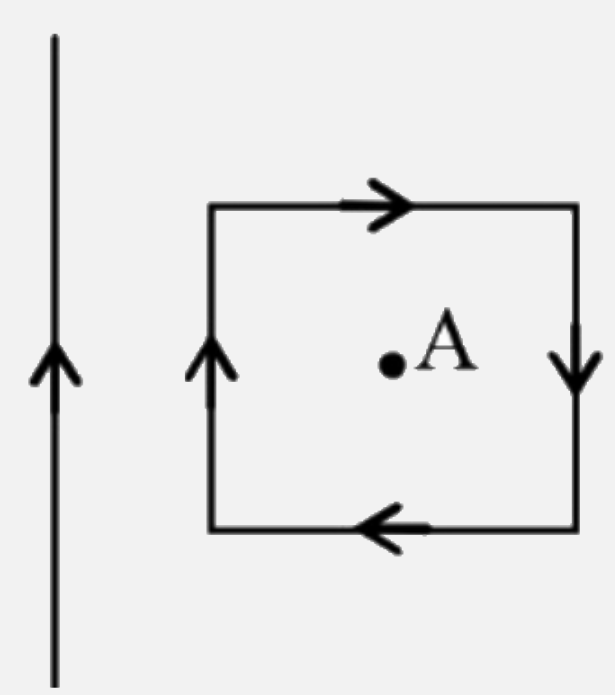
View SolutionA wire is parallel to a square coil . The coil and wire carry currents in the directions Shown . Then, at any point A within the coil the magnetic field will be -
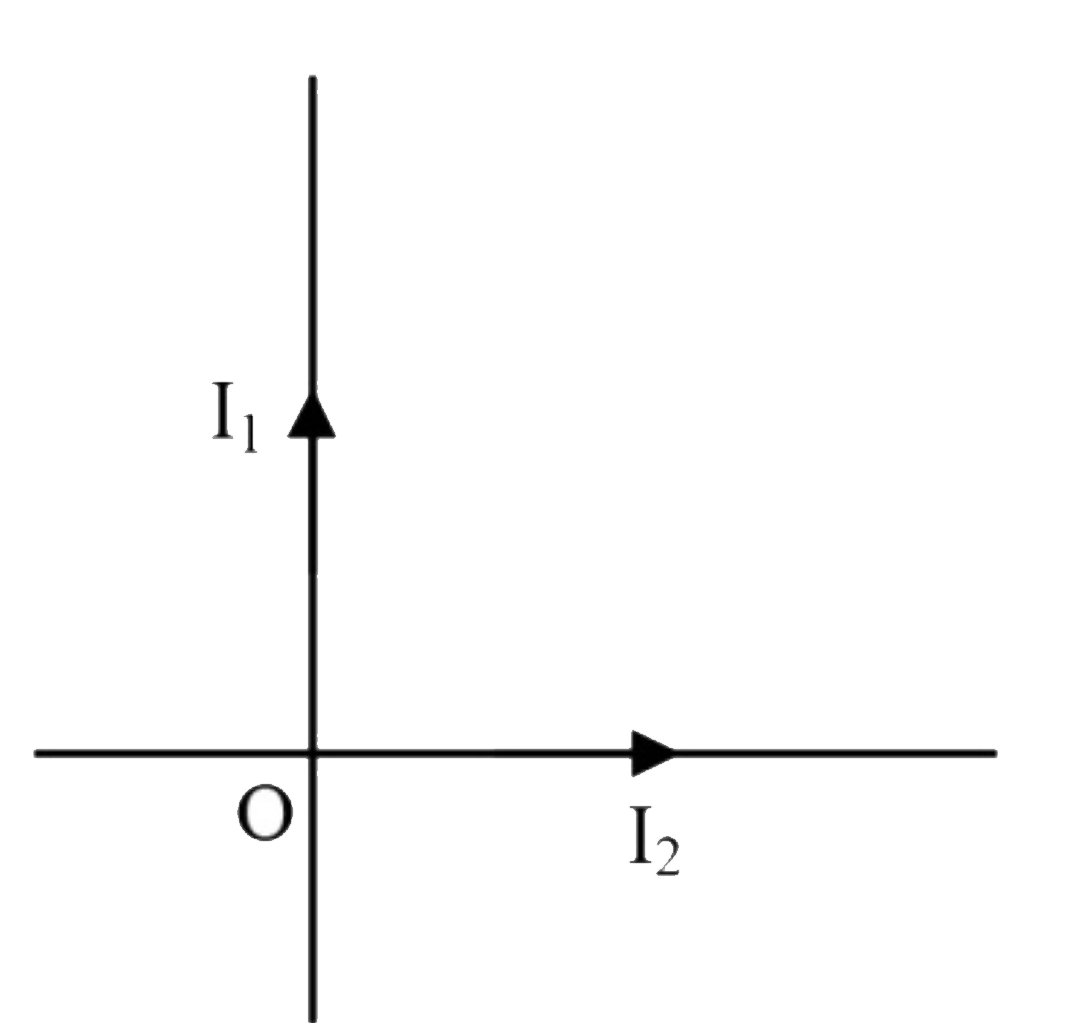
View SolutionTwo long wires carrying current are kept crossed ( not joined at ) . The locus where magnetic field is zero is -
View SolutionAn otherwise infinite, straight wire has two concentric loops of radii a and b carrying equal currents in opposite directions as shown in Fig. The magnetic field at the common centre is zero for
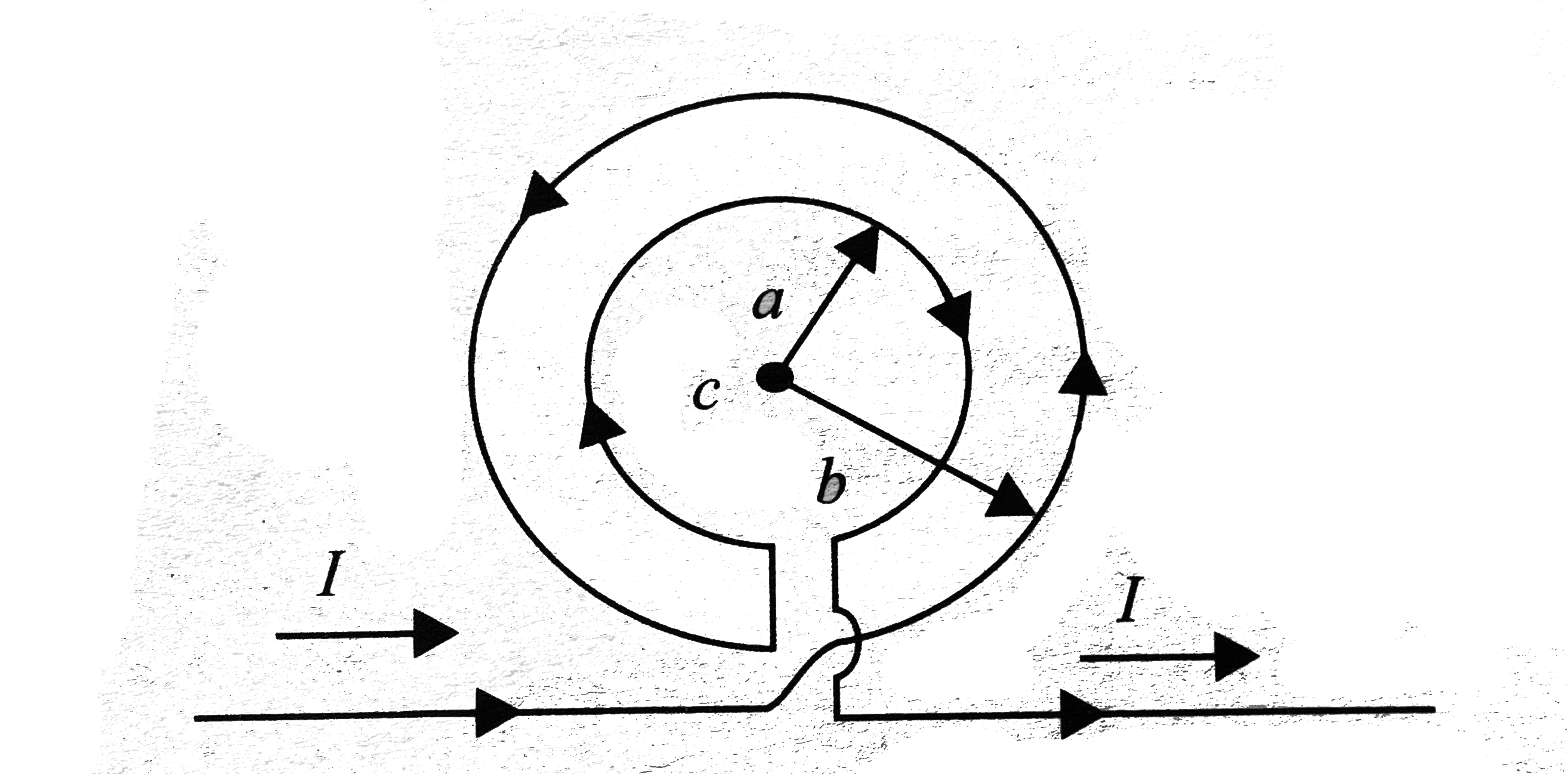
View SolutionTwo very long, straight wires carrying, currents as shown in Fig. Find all locations where the net magnetic field is zero.

View SolutionTwo parallel wires carry equal currents of 10A along the same direction and are separated by a distance of 2.0 cm. Find the magnetic field at a point which is 2.0 cm away from each of these wires.
View Solution
