A hypothetical planet of mass m is moving along an elliptical path around sun of mass Ms under the influence of its gravitational pull. If the major axis is 2R, find the speed of the planet when it is at a distance of R from the sun.
√GMsR
√2GMsR
2√GMsR
mM√GMsR
The correct Answer is:A
To solve the problem, we need to find the speed of a hypothetical planet of mass m when it is at a distance R from the Sun of mass Ms. The major axis of the elliptical orbit is given as 2R, which means the semi-major axis a is R.
1. Identify the Semi-Major Axis:
The semi-major axis a of the elliptical orbit is given by:
a=2R2=R
2. Apply Kepler's Third Law:
According to Kepler's Third Law, the square of the orbital period T of a planet is proportional to the cube of the semi-major axis a:
T2=4π2a3GMs
Substituting a=R:
T2=4π2R3GMs
3. Calculate the Orbital Period T:
Taking the square root of both sides to find T:
T=2π√R3GMs
4. Determine the Speed v:
The speed v of the planet at a distance R can be calculated using the formula for speed in terms of the distance traveled in one complete orbit divided by the period:
v=Circumference of orbitT
The circumference of the orbit (approximated as a circle at distance R) is:
Circumference=2πR
Thus, substituting for T:
v=2πRT=2πR2π√R3GMs
5. Simplify the Expression:
The 2π cancels out:
v=R√R3GMs
This can be simplified further:
v=R√GMsR3/2=√GMsR
Final Result:
The speed of the planet when it is at a distance R from the Sun is:
v=√GMsR
The semi-major axis a of the elliptical orbit is given by:
a=2R2=R
According to Kepler's Third Law, the square of the orbital period T of a planet is proportional to the cube of the semi-major axis a:
T2=4π2a3GMs
Substituting a=R:
T2=4π2R3GMs
Taking the square root of both sides to find T:
T=2π√R3GMs
The speed v of the planet at a distance R can be calculated using the formula for speed in terms of the distance traveled in one complete orbit divided by the period:
v=Circumference of orbitT
The circumference of the orbit (approximated as a circle at distance R) is:
Circumference=2πR
Thus, substituting for T:
v=2πRT=2πR2π√R3GMs
The 2π cancels out:
v=R√R3GMs
This can be simplified further:
v=R√GMsR3/2=√GMsR
The speed of the planet when it is at a distance R from the Sun is:
v=√GMsR
Similar Questions
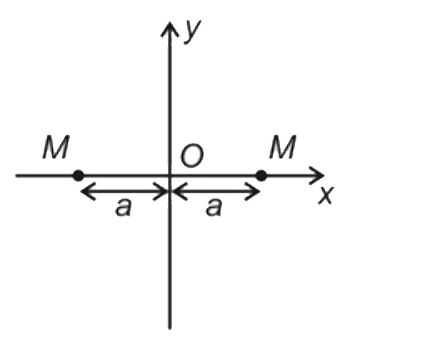
Two point masses M are kept fixed on the x-axis at a distance a from the origin, another point mass m is moving in a circular path of radius R (in y - z plane) under the influence of gravitational force of attraction, then speed of m will be (Assume no forces are acting on m other than the gravitational forces by two M)
View SolutionA particle of mass M moves with constant speed along a circular path of radius r under the action of a force F. Its speed is
View Solution
Knowledge Check
A planet is revolving in an elliptical orbit around the sun. Its closest distance from the sun is r and the farthest distance is R. If the velocity of the planet nearest to the sun be v and that farthest away from the sun be V. then v/V is
AR2/r2Br2/R2CR/rDr/R
A planet of mass m revolves in elliptical orbit around the sun of mass M so that its maximum and minimum distance from the sun equal to ra and rp respectively. Find the angular momentum of this planet relative to the sun.
View SolutionA planet of mass m moves along an ellipse around the Sun so that its maximum and minimum distances from the Sun are equal to r1 and r2 respectively. Find the angular momentum M of this planet relative to the centre of the Sun.
View SolutionTwo hypothetical planets 1 and 2 are moving in the same eliptical path as shown in the figure. If the planets are situated at minimum and maximum distance from the sun and one of the planet, say 1, has speed v. Find the relative angular speed of the planets for the given situation.

View SolutionA planet of mass m is moving in an elliptical orbit about the sun (mass of sun = M). The maximum and minimum distances of the planet from the sun are r1 and r2 respectively. The period of revolution of the planet wil be proportional to :
View SolutionA planet of mass m is in an elliptical orbit about the sun (m << Msun) with an orbital period T. If A be the area of orbit, then its angular momentum would be:
View SolutionA planet of mass m moves along an ellipse around the sum of mass M so that its maximum and minimum distances from sum are a and b respectively. Prove that the angular momentum L of this planet relative to the centre of the sun is L=m√2GMab(a+b)
View SolutionFour particles of equal masses M move along a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
View Solution
