If a small sports car collides head on with a massive track, which vehicle expenences the greater impact force? Which vehicle experiences the greater acceleration?
To solve the problem of which vehicle experiences the greater impact force and which vehicle experiences the greater acceleration during a head-on collision between a small sports car and a massive truck, we can follow these steps:
1. Identify the Vehicles and Their Masses:
- Let the mass of the sports car be m.
- Let the mass of the truck be M (where M≫m).
2. Apply Newton's Third Law of Motion:
- According to Newton's third law, when two objects collide, they exert equal and opposite forces on each other. Therefore, the force experienced by the sports car (Fcar) is equal in magnitude to the force experienced by the truck (Ftruck):
Fcar=−Ftruck
- This means that both vehicles experience the same magnitude of impact force.
3. Set Up the Equations of Motion:
- For the sports car, using Newton's second law:
F=ma⟹Fcar=m⋅acar
- For the truck:
F=Ma⟹Ftruck=M⋅atruck
4. Relate the Accelerations:
- Since the forces are equal in magnitude:
m⋅acar=M⋅atruck
- Rearranging gives:
acar=Mm⋅atruck
5. Determine the Acceleration:
- Since M≫m, the ratio Mm is a large number. This implies that acar will be much greater than atruck:
acar≫atruck
- Therefore, the sports car experiences greater acceleration (or deceleration) in the collision.
6. Conclusion:
- Impact Force: Both vehicles experience equal impact forces.
- Acceleration: The sports car experiences greater acceleration (or deceleration).
Final Answer:
- Greater Impact Force: Both vehicles experience equal impact force.
- Greater Acceleration: The sports car experiences greater acceleration.
- Let the mass of the sports car be m.
- Let the mass of the truck be M (where M≫m).
- According to Newton's third law, when two objects collide, they exert equal and opposite forces on each other. Therefore, the force experienced by the sports car (Fcar) is equal in magnitude to the force experienced by the truck (Ftruck):
Fcar=−Ftruck
- This means that both vehicles experience the same magnitude of impact force.
- For the sports car, using Newton's second law:
F=ma⟹Fcar=m⋅acar
- For the truck:
F=Ma⟹Ftruck=M⋅atruck
- Since the forces are equal in magnitude:
m⋅acar=M⋅atruck
- Rearranging gives:
acar=Mm⋅atruck
- Since M≫m, the ratio Mm is a large number. This implies that acar will be much greater than atruck:
acar≫atruck
- Therefore, the sports car experiences greater acceleration (or deceleration) in the collision.
- Impact Force: Both vehicles experience equal impact forces.
- Acceleration: The sports car experiences greater acceleration (or deceleration).
- Greater Impact Force: Both vehicles experience equal impact force.
- Greater Acceleration: The sports car experiences greater acceleration.
Similar Questions
A large truck and a car, both moving with a velocity of magnitude v, have a head-on collision and both of them come to a halt after that. If the collision lasts for 1s :
(a) Which vehicle experiences the greater force of impact?
(b) which vehicle experiences the greater change in momentum?
(c ) which vehicle experiences the greater acceleration?
(d) why is the car likely to suffer more damage than the truck?
View SolutionA woman riding a bicycle collides headon with a parked school bus. Which object feels greater force ?
View Solution
Knowledge Check
Assertion : Ea of the forward reaction is higher than that of backward reaction in a reversible endothermic reaction.
Reason : Increasing the tempreature of the substance increases the fraction of molecules which collide with energies greater than Ea.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.
A car runs at constant speed around the horizontal race track shown in the figure. Over which portion of the track is the magnitude of the acceleration the greatest?

View SolutionTwo planets of equal mass orbit a much massive star (figure). Planet m1 moves in circular orbit of radius 1×108km with period 2yr. Planet m2 moves in an elliptical orbit with closed distance r1=1×108km and farthest distance r2=1.8×108km, as shows.
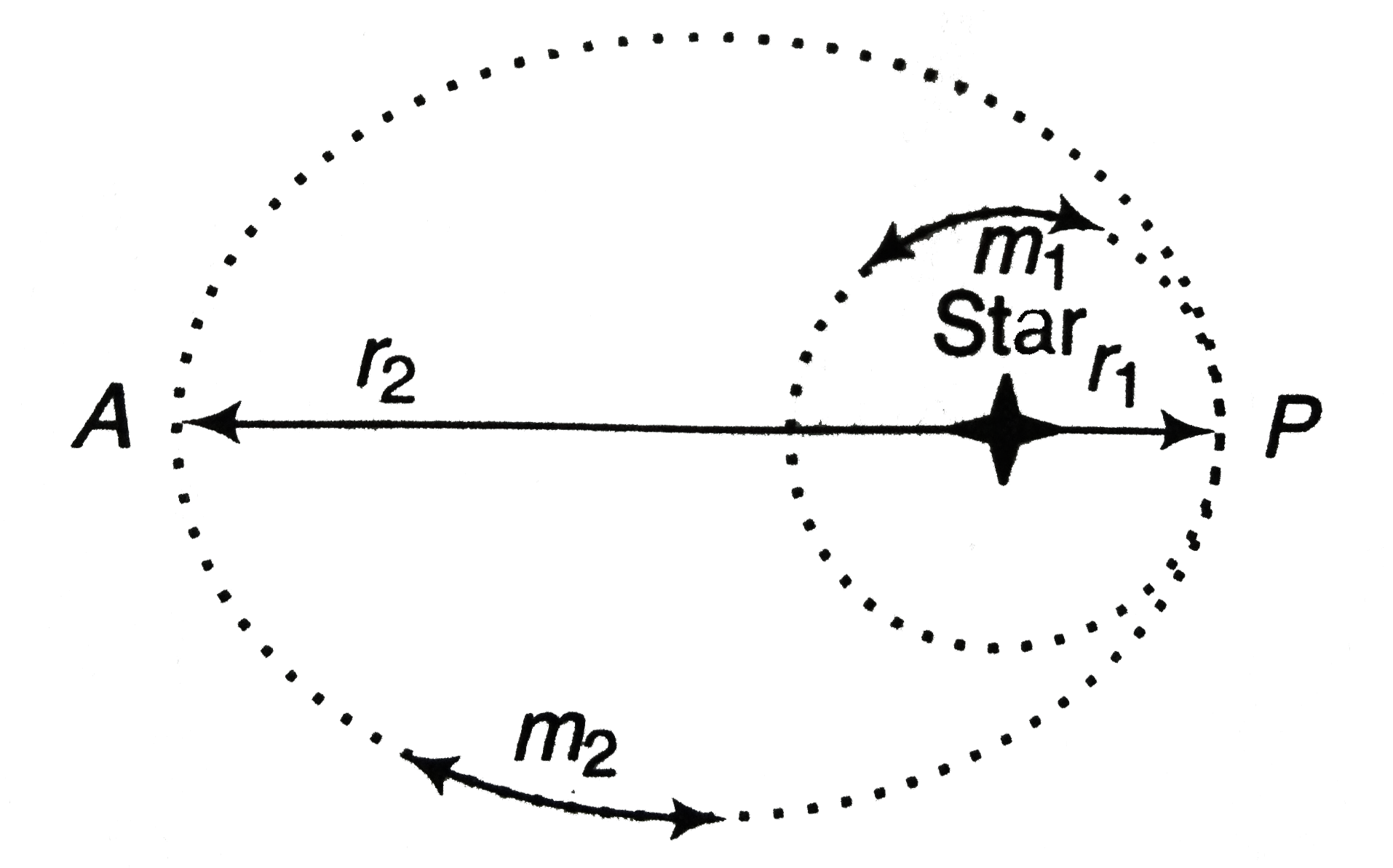
(a) Using the fact that the mean radius of an elliptical orbit is the length of the semi-major axis, find the period of m2's orbit.
(b) Which planet has the greater speed at point P? Which has the greater total energy?
(c ) Compare the speed of planet m2 at P with that at A.
View Solution(a). A cube of side 5 cm is immersed in water and then in saturated salt solution. In which case will it experience a greater buoyant force. If each side of the cube is reduced to 4 cm and then immersed in water, what will be the effect on the buoyant force experienced by the cube as compared to the first case for water. Given reason for each case.
(b). A ball weghing 4 kg of density 4000 kg/m3 is completely immersed in water of density 103kg/m3 find the force of buoyancy on it. (given g=10m/s2)
View SolutionA small toy car moves round a circular track of radius 4 m. The car makes one revolution in 10 s. Calculate (a) the speed of the car, (b) its centripetal acceleration, (c) the centripetal force exerted,by the track if the.mass of the car is 200 g, (d) the safe speed with which the car can move around without toppling, if the distance between the wheels is 4 cm and the height of the centre of gravity of the car from the horizontal is 2 cm.
View SolutionA car of mass m is driven with acceleration a along a straight level road against a constant external resistive force R. When the velocity of the car V, the rate at which the engine of the car is doing work will be
View SolutionA car of mass m is driven with acceleration a along a straight level road against a constant external resistive force R. When the velocity of the car V, the rate at which the engine of the car is doing work will be
View SolutionTwo identical balls moving in opposite directions in free space collide head on. How they will move after the impact, is decided by coefficient of restitution. For different coeffecients of restiution, few possible outcomes are shown in the following figures. Which of the above outcomes are physically possible for a collision?

View Solution
