Period of oscillation of a bar magnet in earth's magnetic field is T . If its mass is reduced to one - fourth of the original mass , maintaining all other parameters same , the new time period will be
T
2 T
(T/2)
(T/4)
The correct Answer is:C
To solve the problem, we need to analyze the relationship between the time period of oscillation of a bar magnet in a magnetic field and its mass. The formula for the time period T of a bar magnet in a magnetic field is given by:
T=2π√ImB
Where:
- T = time period of oscillation
- I = moment of inertia of the magnet
- m = mass of the magnet
- B = magnetic field strength
Step 1: Understand the effect of mass on the time period
Given that the mass of the magnet is reduced to one-fourth of its original mass, we denote the original mass as m and the new mass as:
m′=m4
Step 2: Substitute the new mass into the time period formula
Since all other parameters (moment of inertia I and magnetic field B) remain constant, we can substitute m′ into the time period formula:
T′=2π√Im′B=2π
⎷I(m4)B=2π√4ImB
Step 3: Relate the new time period to the original time period
Now we can express the new time period T′ in terms of the original time period T:
T′=2π√4ImB=2π⋅2√ImB=2T
Step 4: Conclusion about the new time period
Since we have derived that:
T′=2T
This means that the new time period T′ is twice the original time period T.
Final Answer
The new time period will be:
T′=2T
- T = time period of oscillation
- I = moment of inertia of the magnet
- m = mass of the magnet
- B = magnetic field strength
⎷I(m4)B=2π√4ImB
Similar Questions
Time period of a bar magnet oscillating in earth's magnetic field is T. Its magnetic moment is M. Now, a bar magnet of magnetic moment 2M is brought near it. The new time period will be
View SolutionA bar magnet is oscillating in the earth's magnetic field with a time period T. If the mass is increased four times, then its time period will be:
View Solution
Knowledge Check
- Question 1 - Select One
The time period of oscillation of a bar magnet suspended horizontaliy along the magnetic meridian is T0. If this magnet is replaced by another magnet of the same size and pole strength but with double the mass, the new time period will be
AT02BT0√2C√2T0D2T0
The time period of the magnetic in an oscillation pmagnetometer in the earth magnetic field is 2s. A short bar magnet is placed to the north of the magnetometer, at a separation 10cm from the oscillation magnet, with its north pole pointing towards north. The time period beceomes half. Calculate the magnetic moment of this short magnet.
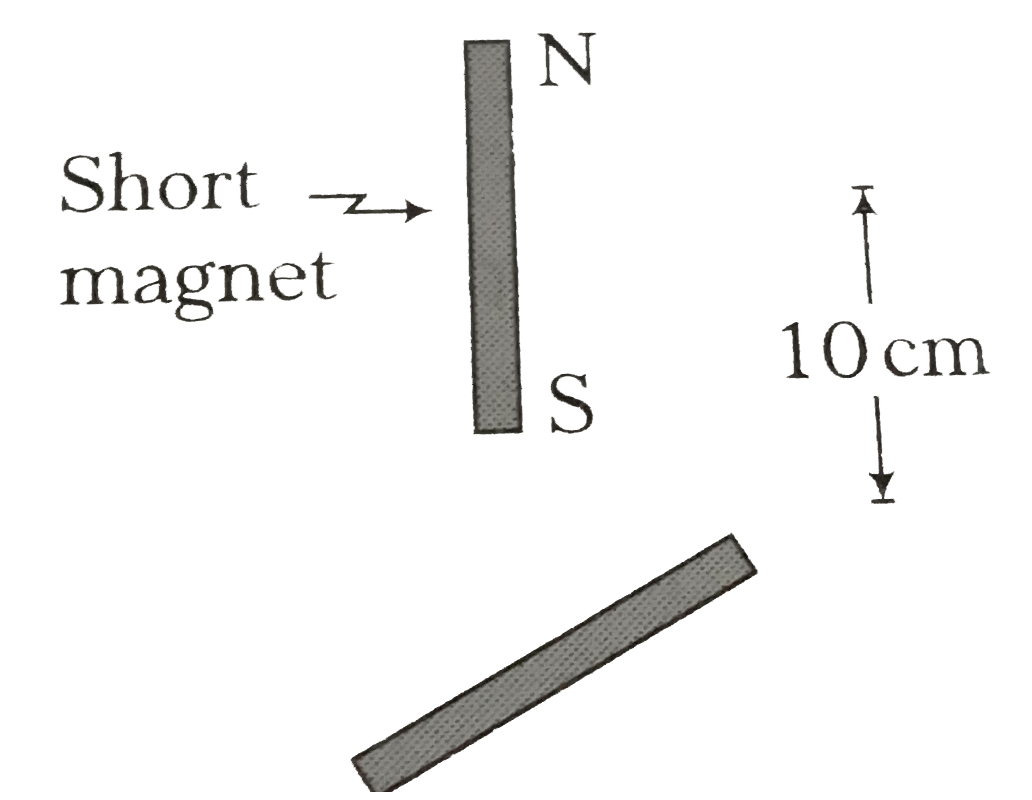 View Solution
View SolutionA charged particle moves in a circular path in a uniform magnetic field. If its speed is reduced, then its tiem period will
View SolutionA vibration magnetometer consist of two identical bar magnets placed one over the other such that they are mutually perpendicular and bisect each other. The time period of oscillations of combination in a horizontal magnetic field is 4s. If one of the magnets is removed, then the period of oscillations of the other in the same field is
View SolutionThe time period of oscillation of a freely suspended bar magnet with usual notations is given by
View SolutionThe time period of a mass suspended from a spring is T. If the spring is cut into four equal parts and the same mass is suspended from one of the parts, then the new time period will be
View SolutionThe period of oscillations of a magnet is 2 sec. When it is remagnetised so that the pole strength is 4 times its period will be
View SolutionThe period of oscillations of a magnet is 2 sec. When it is remagnetised so that the pole strength is 4 times its period will be
View Solution

