Two identical tennis ball of mass m and charge q are hinged by a common support with help of a string of length 'l'. If system is in equilibrium then find distance between balls? ignore gravitational interactions between balls. (θ is very small)
(kq2lmg)13
(3kq2lmg)13
(2kq2lmg)13
(3kq2l2mg)13
To solve the problem of finding the distance between two identical tennis balls of mass m and charge q that are hinged by a common support with a string of length l, we will follow these steps:
Step 1: Understand the Forces Acting on the Balls
The two tennis balls are charged and will repel each other due to the electrostatic force. The gravitational force acting on each ball is mg (where g is the acceleration due to gravity), but we will ignore this force as per the problem statement. The tension in the string will also play a role in the equilibrium of the system.
Step 2: Set Up the Geometry
Each ball is connected to the common support by a string of length l. When the balls are in equilibrium, they will form an angle θ with the vertical. The horizontal distance between the two balls can be denoted as x.
Step 3: Analyze the Forces
For each ball, we can break down the tension T in the string into two components:
- The vertical component: Tcosθ
- The horizontal component: Tsinθ
In equilibrium, the horizontal component of the tension must equal the electrostatic force F between the two balls, and the vertical component must balance the weight of the balls (which we ignore).
Step 4: Write the Equations
From the geometry, we can express the distance x between the two balls in terms of θ:
x=2Lsinθ
Since θ is small, we can use the small angle approximation:
sinθ≈θ
Thus,
x≈2Lθ
Step 5: Relate the Forces
The electrostatic force F between the two balls can be expressed using Coulomb's Law:
F=kq2x2
where k is Coulomb's constant.
Step 6: Set Up the Equilibrium Condition
From the equilibrium condition, we have:
Tsinθ=F
Substituting for F:
Tsinθ=kq2x2
Step 7: Substitute for T
The vertical component of tension can be related to the weight of the balls:
Tcosθ=mg
Thus, we can express T as:
T=mgcosθ
Step 8: Substitute T into the Force Equation
Substituting T into the equilibrium condition gives:
mgcosθsinθ=kq2x2
This simplifies to:
mgtanθ=kq2x2
Step 9: Substitute for tanθ
Using the small angle approximation tanθ≈θ:
mgθ=kq2x2
Step 10: Substitute for x
Substituting x≈2Lθ into the equation gives:
mgθ=kq2(2Lθ)2
This simplifies to:
mgθ=kq24L2θ2
Step 11: Rearranging the Equation
Multiplying both sides by 4L2θ2 gives:
4L2mgθ3=kq2
Step 12: Solve for θ
Rearranging for θ3:
θ3=kq24L2mg
Step 13: Substitute Back to Find x
Now substituting back to find x:
x=2Lθ≈2L(kq24L2mg)1/3
Thus, the final expression for the distance x between the two balls is:
x=2k1/3q2/3(4L2mg)1/3
Final Result
The distance x between the two balls is:
x=2k1/3q2/3L2/3(mg)1/3
The two tennis balls are charged and will repel each other due to the electrostatic force. The gravitational force acting on each ball is mg (where g is the acceleration due to gravity), but we will ignore this force as per the problem statement. The tension in the string will also play a role in the equilibrium of the system.
Each ball is connected to the common support by a string of length l. When the balls are in equilibrium, they will form an angle θ with the vertical. The horizontal distance between the two balls can be denoted as x.
For each ball, we can break down the tension T in the string into two components:
- The vertical component: Tcosθ
- The horizontal component: Tsinθ
From the geometry, we can express the distance x between the two balls in terms of θ:
x=2Lsinθ
Since θ is small, we can use the small angle approximation:
sinθ≈θ
Thus,
x≈2Lθ
The electrostatic force F between the two balls can be expressed using Coulomb's Law:
F=kq2x2
where k is Coulomb's constant.
From the equilibrium condition, we have:
Tsinθ=F
Substituting for F:
Tsinθ=kq2x2
The vertical component of tension can be related to the weight of the balls:
Tcosθ=mg
Thus, we can express T as:
T=mgcosθ
Substituting T into the equilibrium condition gives:
mgcosθsinθ=kq2x2
This simplifies to:
mgtanθ=kq2x2
Using the small angle approximation tanθ≈θ:
mgθ=kq2x2
Substituting x≈2Lθ into the equation gives:
mgθ=kq2(2Lθ)2
This simplifies to:
mgθ=kq24L2θ2
Multiplying both sides by 4L2θ2 gives:
4L2mgθ3=kq2
Rearranging for θ3:
θ3=kq24L2mg
Now substituting back to find x:
x=2Lθ≈2L(kq24L2mg)1/3
Thus, the final expression for the distance x between the two balls is:
x=2k1/3q2/3(4L2mg)1/3
The distance x between the two balls is:
x=2k1/3q2/3L2/3(mg)1/3
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
Two indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View SolutionTwo identical ball having mass density ρ , mass m and charge q are suspended from a common point by two insulating massless strings of equal length. At equilibrium each string makes an angle theta with the vertical. Now both the balls are immersed in a fluid. At equilibrium, theta remains same. If the mass density of liquid is σ then dielectric constant of liquid will be
View Solution
Knowledge Check
Two identical tennis balls each having mass 'm' and charge 'q' are suspended from a fixed point by threads of length 'l'. What is the equilibrium separation when each thread makes a small angle 'θ' with the vertical ?
Ax=(q2l2πε0mg)1/2Bx=(q2l2πε0mg)1/3Cx=(q2l22πε0m2g)1/3Dx=(q2l22πε0m2g2)1/3Two identical small bodies each of mass m and charge q are suspended from two strings each of length l from a fixed point. This whole system is taken into an orbiting artificial satellite, then find the tensio in strings
AKq2l2+2mgBKq24l2+2mgCKq2l2DKq24l2Assertion : Two identical balls are charged by q. They are suspended from a common point by two insulating threads l each. In equilibrium, the angle between the tension in the threads is 180∘. (Ignore gravity).
Reason : In equilibrium tension in the spring is
T=14πε0q.ql2AIf both Assertion and Reason are correct and Reason is the correct explanation of AssertionBIf both Assertion and Reason are correct but Reason is not the correct explanation of AssertionCIf Assertion is true but Reason is falseDIf Assertion is false but Reason is true
Two idential pith balls , each of mass m and charge +q. are suspended from a point with threads of length l each. If in equiilbrium state θ be the angle which each thread makes with vertical in equilibrium , find value of charge on each ball.
View SolutionTwo identical small bodies each of mass m and charge q are suspended from two strings each of length l from a fixed point. This whole system is taken into an orbiting artificial satellite, then find the tensio in strings
View SolutionTwo identical spheres of mass m and charge q are suspended from a common point by two threads of the same length l and it is found that threads make an angle θ with the vertical when the spheres are in equilibrium . Calculate the value of q.
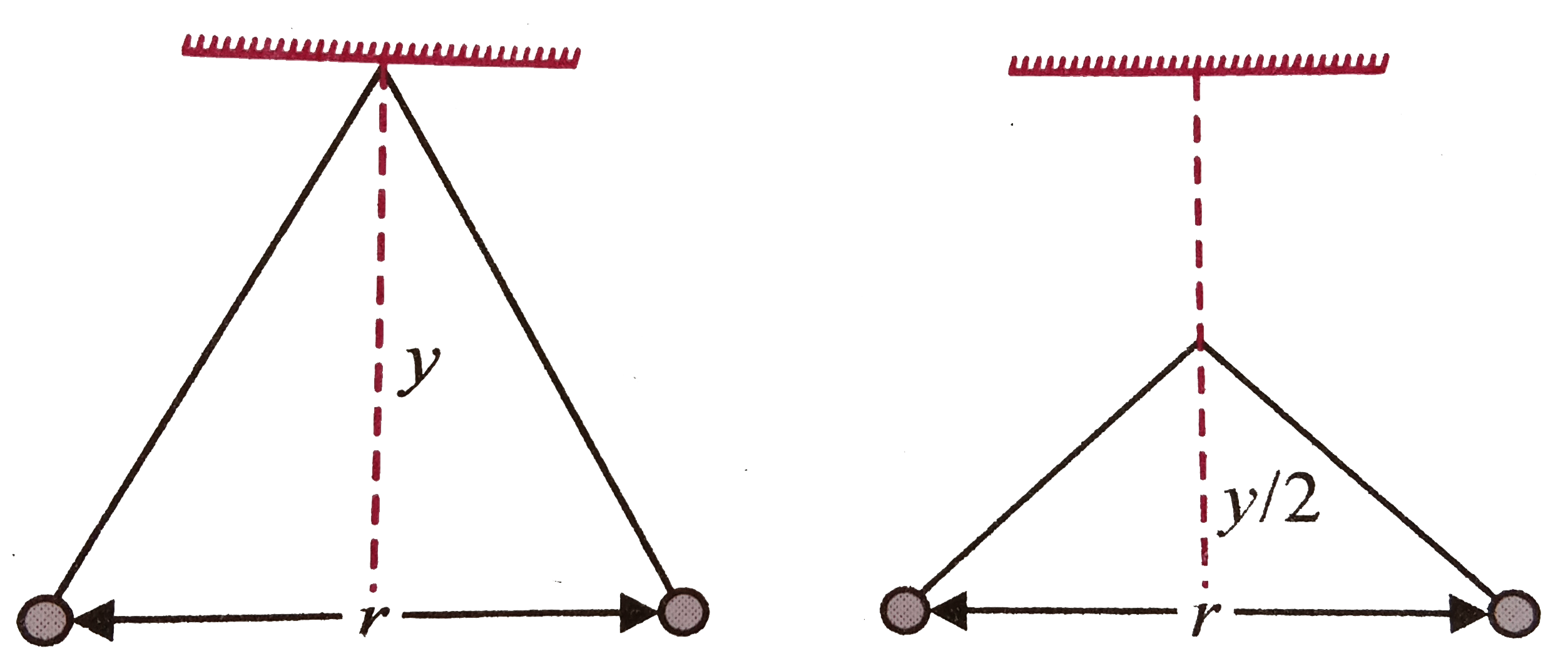
View SolutionTwo path balls carrying eqaul chareges are suspended froom a common point by strings of equal length, the strings are rightly clamped at half the height. The equilibrium separation between the balls, now becomes :
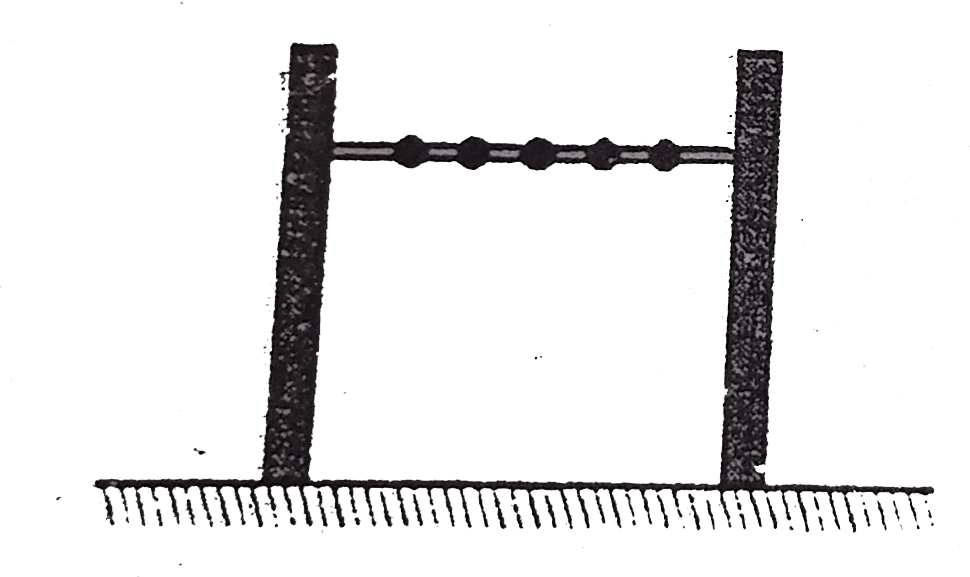
View SolutionFive identical balls each of mass m and radius r are string like beads at random and at rest along a smooth rigid horizontal thin rod of length L mounted between immovable supports Assume 10r≤L and that the collision between balls or between balls and supports are elastic if one ball is struck horizontally so as to acquire a speed v the average force felt by the support is
View Solution
