ABC is a triangle and the sides AB, BC and CA are produced to E, F and G respectively. If ∠CBE=∠ACF=130∘ then the value of ∠GAB is
100∘
130∘
80∘
90∘
The correct Answer is:A
To solve the problem step by step, we will analyze the triangle ABC and the angles given.
Step 1: Understand the Triangle and Given Angles
We have triangle ABC with sides extended to points E, F, and G. We know:
- Angle CBE = 130°
- Angle ACF = 130°
Step 2: Identify Angles X and Y
Let:
- Angle GAB = X
- Angle ABC = Y
Step 3: Apply Linear Pair on Angle CBE
Since angle CBE and angle GAB (X) are on a straight line:
X+Angle CBE=180°
Substituting the value of Angle CBE:
X+130°=180°
Thus,
X=180°−130°=50°
Step 4: Apply Linear Pair on Angle ACF
Similarly, for angle ACF:
Y+Angle ACF=180°
Substituting the value of Angle ACF:
Y+130°=180°
Thus,
Y=180°−130°=50°
Step 5: Apply Angle Sum Property in Triangle ABC
The sum of angles in triangle ABC is:
X+Y+Angle BAC=180°
Substituting the values of X and Y:
50°+50°+Angle BAC=180°
This simplifies to:
100°+Angle BAC=180°
Thus,
Angle BAC=180°−100°=80°
Step 6: Apply Linear Pair on Angle CAG
Now, we apply the linear pair on angle CAG:
Angle BAC+Angle GAB=180°
Substituting the value of Angle BAC:
80°+Angle GAB=180°
Thus,
Angle GAB=180°−80°=100°
Conclusion
The value of angle GAB is 100°.
---
We have triangle ABC with sides extended to points E, F, and G. We know:
- Angle CBE = 130°
- Angle ACF = 130°
Let:
- Angle GAB = X
- Angle ABC = Y
Since angle CBE and angle GAB (X) are on a straight line:
X+Angle CBE=180°
Substituting the value of Angle CBE:
X+130°=180°
Thus,
X=180°−130°=50°
Similarly, for angle ACF:
Y+Angle ACF=180°
Substituting the value of Angle ACF:
Y+130°=180°
Thus,
Y=180°−130°=50°
The sum of angles in triangle ABC is:
X+Y+Angle BAC=180°
Substituting the values of X and Y:
50°+50°+Angle BAC=180°
This simplifies to:
100°+Angle BAC=180°
Thus,
Angle BAC=180°−100°=80°
Now, we apply the linear pair on angle CAG:
Angle BAC+Angle GAB=180°
Substituting the value of Angle BAC:
80°+Angle GAB=180°
Thus,
Angle GAB=180°−80°=100°
The value of angle GAB is 100°.
Topper's Solved these Questions
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IV)
35 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-V)
30 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-II)
25 Videos
- DISCOUNTBOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself
10 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
Similar Questions
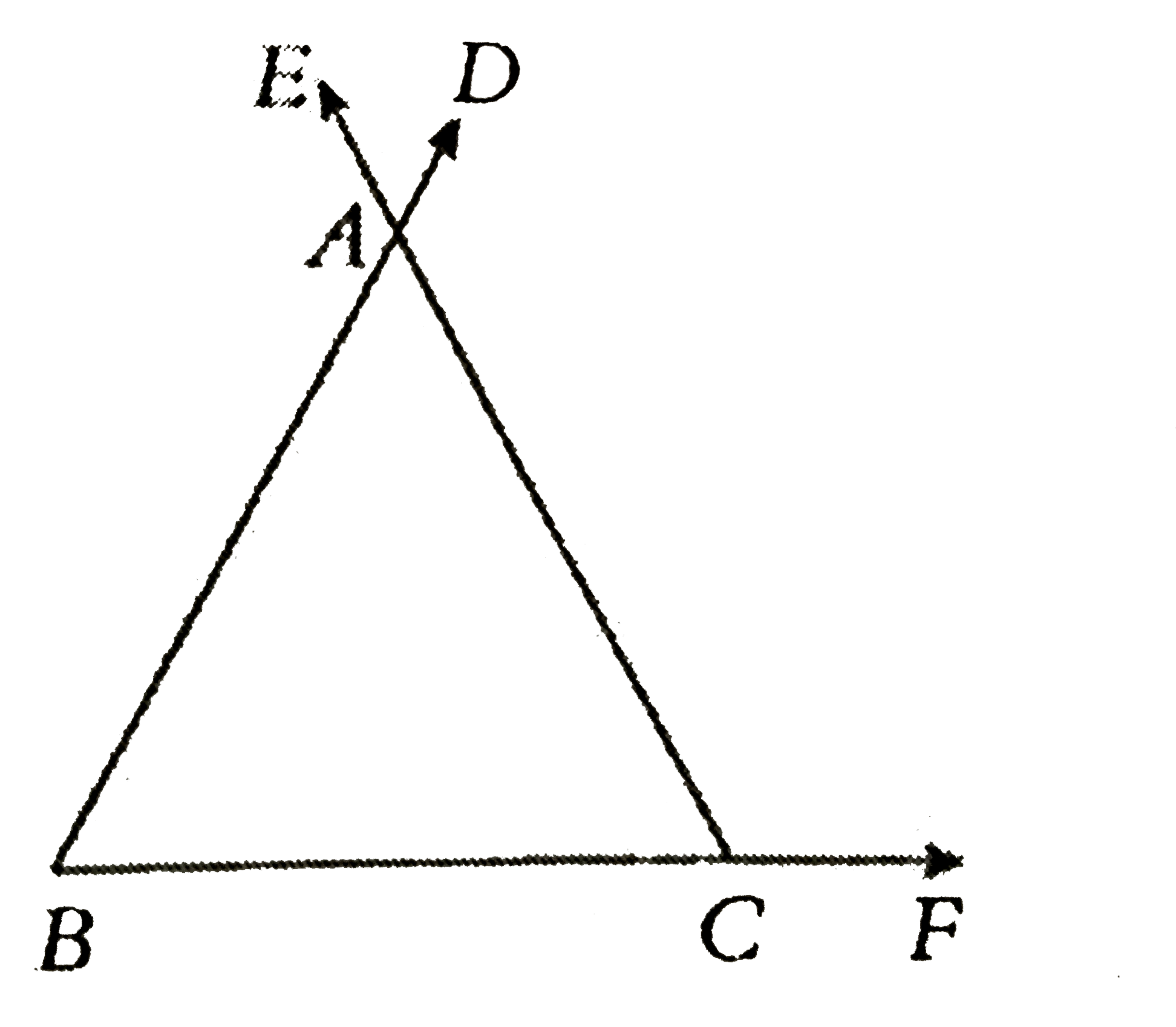
In the above figure ( not to scale ) the sides BA,BC and CA ofΔABC are produced to D,F, and E respectively such that ∠ACF=120∘ and ∠BAE=150∘. Then ∠ABC=_____.
View SolutionAn exterior angle of a triangle is 1100, and one of the interior opposite angles is 300. Find the other two angles of the triangle.
View Solution
Knowledge Check
ABC is a triangle and the sides AB, BC and CA are produced to E, F and G respectively. If ∠CBE=∠ACF=130∘ then the value of ∠GAB is
A100∘B80∘C130∘D90∘ΔABC is an isosceles triangle and AB = AC. If all the sides AB. BC and CA of a ΔABC touch a circle at D, E and F respectively. Then BE is equal to :
A12 BCB13 BCC14 BCD23 BCG is the centroid of the triangle ABC, where AB, BC and CA are 7 cm, 24 cm and 25 cm respectively, then BG is:
G त्रिभुज ABC का केन्द्रक है जिसमें AB, BC और CA क्रमशः 7 सेमी, 24 सेमी और 25 सेमी हैं | BG का मान ज्ञात करें |A613cmB813cmC512cmD416cm
In the given figure, a circle inscribed in a triangle ABC touches the sides AB, BC and CA at points D, E and F respectively. If AB = 14 cm, BC = 8 cm and CA = 12 cm. Find the lengths AD, BE and CF.
View SolutionIn a triangle ABC,DandE are the mid-points of BC,CA respectively. If AD=5,BC=BE=4,then CA=
View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
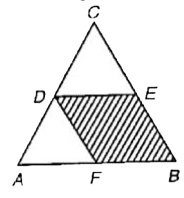
View SolutionIn a ΔABC, D, E and F are the midpoints of BC, CA and AB respectively. If the lengths of side AB, BC and CA are 7 cm, 8 cm and 9 cm respectively, the perimeter of ΔDEF is a cm. The value of a/2 is
View SolutionIn △ABC,D, E and F are the midpoints of sides AB, BC and CA, respectively. If AB = 12 cm, BC = 20 cm and CA = 15 cm, then the value of 12(DE+EF+DF) is:
त्रिभुज ABC में, D, E तथा F क्रमशः भुजाओं AB, BC तथा CA के मध्य बिंदु हैं | यदि AB =12 सेमी, BC = 20 सेमी तथा CA =15 सेमी है, तो 12(DE+EF+DF) का मान क्या होगा ?
View Solution
