The side BC of a triangle ABC is produced to D. If ∠ACD=112∘ and ∠B=34∠A, then the mea sure of ∠B is
30∘
48∘
45∘
64∘
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the given information
We are given:
- Angle ACD = 112°
- Angle B = (3/4) * Angle A
Step 2: Use the exterior angle theorem
According to the exterior angle theorem, the exterior angle (ACD) is equal to the sum of the two opposite interior angles (A and B). Therefore, we can write the equation:
∠A+∠B=∠ACD
Substituting the known value:
∠A+∠B=112°
Step 3: Substitute the expression for angle B
We know that:
∠B=34∠A
Substituting this into the equation from Step 2:
∠A+34∠A=112°
Step 4: Combine like terms
Combining the angles, we have:
44∠A+34∠A=112°
This simplifies to:
74∠A=112°
Step 5: Solve for angle A
To find angle A, multiply both sides by 4:
7∠A=448°
Now, divide by 7:
∠A=448°7=64°
Step 6: Find angle B
Now that we have angle A, we can find angle B using the relation:
∠B=34∠A
Substituting the value of angle A:
∠B=34×64°=48°
Conclusion
The measure of angle B is:
∠B=48°
---
We are given:
- Angle ACD = 112°
- Angle B = (3/4) * Angle A
According to the exterior angle theorem, the exterior angle (ACD) is equal to the sum of the two opposite interior angles (A and B). Therefore, we can write the equation:
∠A+∠B=∠ACD
Substituting the known value:
∠A+∠B=112°
We know that:
∠B=34∠A
Substituting this into the equation from Step 2:
∠A+34∠A=112°
Combining the angles, we have:
44∠A+34∠A=112°
This simplifies to:
74∠A=112°
To find angle A, multiply both sides by 4:
7∠A=448°
Now, divide by 7:
∠A=448°7=64°
Now that we have angle A, we can find angle B using the relation:
∠B=34∠A
Substituting the value of angle A:
∠B=34×64°=48°
The measure of angle B is:
∠B=48°
Topper's Solved these Questions
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IV)
35 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-V)
30 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-II)
25 Videos
- DISCOUNTBOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself
10 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
Similar Questions
The side BC of a triangle ABC is produced to D, bisector of the angle ABC and ACD meet at P. If ∠BPC=x∘ and ∠BAC=y∘, then which one of the following is correct ?
View SolutionThe sides BC,CA and AB of a triangle ABC, are produced in order, forming exterior angles ∠ACD,∠BAE and ∠CBF . Show that ∠ACD+∠BAE+∠CBF=3600
View Solution
Knowledge Check
In △ABC, side BC has been produced to D. If ∠ACD= 132∘ and ∠A = 54∘, then ∠B = ?
A48∘.B78∘C68∘D58∘∠Aof ΔABC is a right angle. AD is perpendicular on BC. If BC = 14 cm and BD = 5 cm, then measure of AD is :
A2√5cm.B√5cm.C3√5cm.D3.5√5cm.In the triangle ABC, side BC is produced to D. ∠ACD=100∘ if BC = AC. Then ∠ABC is :
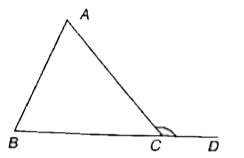 A40∘B50∘C80∘Dcan't be determined
A40∘B50∘C80∘Dcan't be determined
In a triangle ABC, if AB=AC and AB is produced to D such that BD=BC, find ∠ACD : ∠ADC
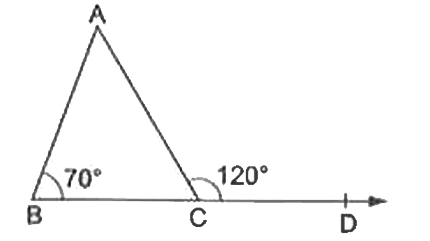
View SolutionIn the given figure, side BC of ∠ABC is produced to D such that ∠ABC=70∘ and ∠ACD=120∘. Then, ∠ BAC = ?
View SolutionIn triangle ABC,∠BAC=75∘,∠ABC=45∘.¯¯¯¯¯¯BC is produced to D. If ∠ACD=x∘, then x3% of 60∘ is
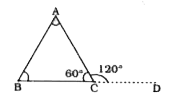
View SolutionIf in a triangle ABC as drawn in the figure, AB=AC and ∠ACD=120∘, then ∠A is equal to
View SolutionIn ΔABC,Ifac=3,bc=4and(A−B)=34, then
View Solution
