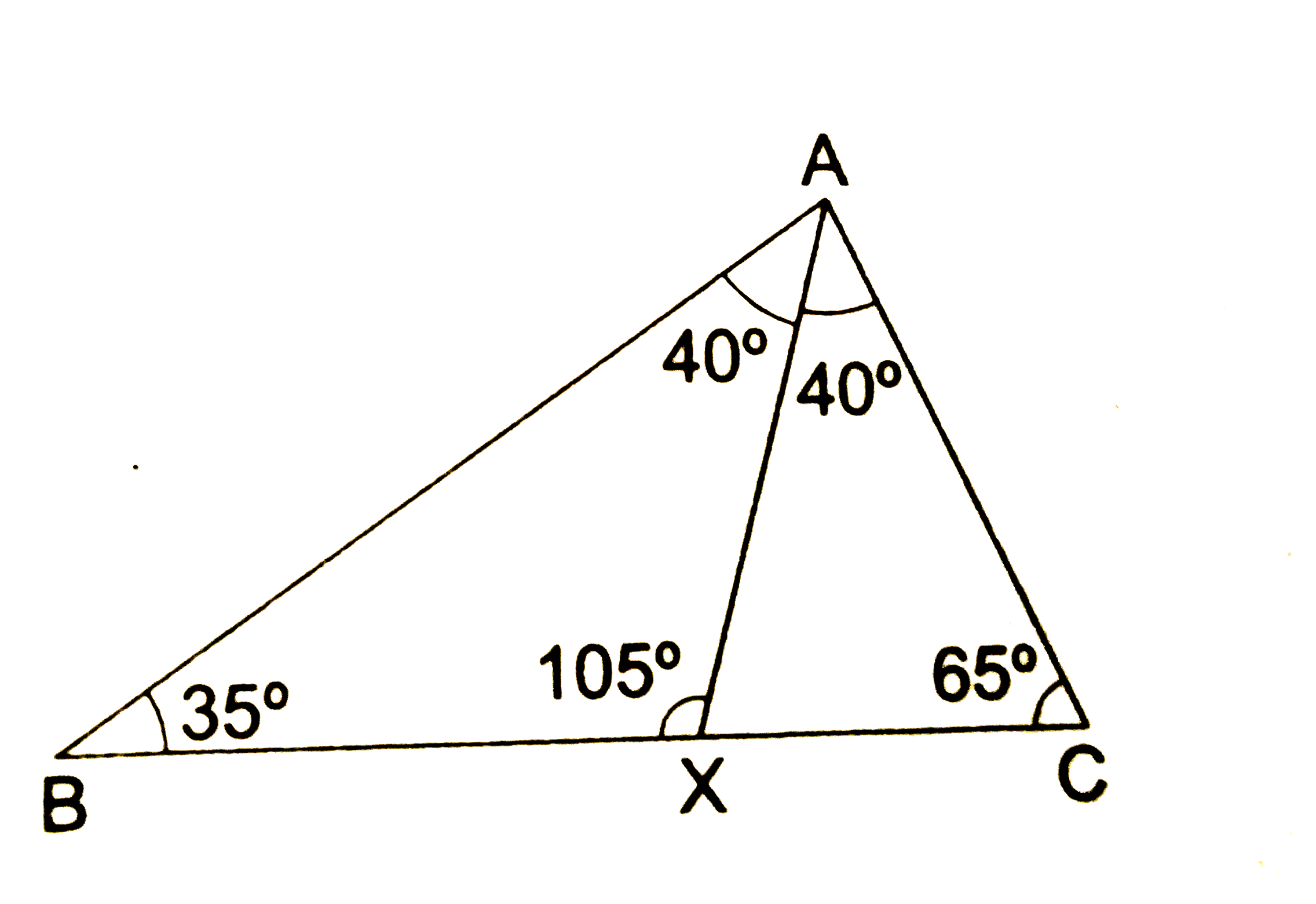In and the bisector of meets BC in D? Then is :
The correct Answer is:D
To solve the problem step by step, we will follow the reasoning provided in the video transcript.
Step 1: Determine the angles of triangle ABC
We know that in triangle ABC:
- Angle B = 35°
- Angle C = 65°
To find angle A, we use the property that the sum of angles in a triangle is 180°.
Calculation:
Angle A=180°−Angle B−Angle C=180°−35°−65°=80°
Step 2: Identify the angle bisector
The angle bisector of angle A (which is 80°) divides it into two equal parts. Therefore, each part will be:
Angle BAD=Angle CAD=80°2=40°
Step 3: Analyze triangle ADB
Now we focus on triangle ADB. We know:
- Angle B = 35°
- Angle ADB (which we need to find) = ?
- Angle BAD = 40°
Step 4: Use the triangle angle sum property
In triangle ADB, the sum of the angles must equal 180°:
Angle ADB+Angle BAD+Angle B=180°
Substituting the known values:
Angle ADB+40°+35°=180°
Step 5: Solve for angle ADB
Now we can solve for angle ADB:
Angle ADB+75°=180°
Angle ADB=180°−75°=105°
Conclusion
Thus, the value of angle ADB is:
Angle ADB=105°
We know that in triangle ABC:
- Angle B = 35°
- Angle C = 65°
Angle A=180°−Angle B−Angle C=180°−35°−65°=80°
The angle bisector of angle A (which is 80°) divides it into two equal parts. Therefore, each part will be:
Angle BAD=Angle CAD=80°2=40°
Now we focus on triangle ADB. We know:
- Angle B = 35°
- Angle ADB (which we need to find) = ?
- Angle BAD = 40°
In triangle ADB, the sum of the angles must equal 180°:
Angle ADB+Angle BAD+Angle B=180°
Substituting the known values:
Angle ADB+40°+35°=180°
Now we can solve for angle ADB:
Angle ADB+75°=180°
Angle ADB=180°−75°=105°
Thus, the value of angle ADB is:
Angle ADB=105°
Topper's Solved these Questions
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IV)
35 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-V)
30 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-II)
25 Videos
- DISCOUNTBOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself
10 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
Similar Questions
Knowledge Check
In an isosceles triangle ΔABC,AB=AC and ∠A=80∘. The bisector of ∠Band∠C meet at D. The ∠BDC is equal to the
A90∘B100∘C130∘D80∘In △ABC ,∠A=40∘. If the bisector of ∠B and ∠C meet at a point O in the interior of the triangle, then ∠BOC is equal to:
त्रिभुज ABC में, ∠A=40∘ है |यदि कोण B तथा कोण C के द्विभाजक त्रिभुज के आतंरिक भाग में एक बिंदु O पर मिलते हैं, तो ∠BOC का क्या होगा ?A130∘B90∘C70∘D110∘In ΔABC,∠C=54∘, the perpendicular bisector of AB at D meets BC at E. If ∠EAC=42∘, then what is the value (in degrees) of ∠ABC?
A25B42C50D60
In ΔABC;∠B=45∘;∠C=55∘ and bisector of ∠A meets BC at a point D. find ∠ADBand∠ADC
View SolutionIn ABC, ∠B=450, ∠C=550 and bisector of ∠A meets BC at a point D. Find ∠ADB and ∠ADC
View SolutionIn triangle ABC,∠B=45∘,∠C=55∘ and bisector of ∠A meets BC at a point D. Find ∠ADB and ∠ADC.
View SolutionIn ΔABC,∠B=35∘,∠C=65∘and∠BAC is bisected and it meet BC at X, then put ¯¯¯¯¯¯AX,¯¯¯¯¯¯BX and ¯¯¯¯¯¯AB in decending order-
View SolutionIn ΔABC,∠BAC=90∘ and AB=12BC. Then the measure of ∠ACB is :
View Solution