Question

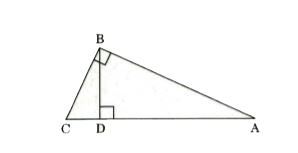
In triangle $ABC,\angle BAC={90}^{\circ }$and AD is perpendicular to BC. If $AD=6cm$and $BD=4cm$, then the length of BC is:

Options

A. 10 cm
B. 12 cm
C. 13 cm
D. 15 cm.

Answer

C

Explanation

To find the length of $$BC$$ in triangle $$ABC$$ where $$\angle BAC={90}^{\circ }$$, $$AD$$ is perpendicular to $$BC$$, $$AD=6\text{cm}$$, and $$BD=4\text{cm}$$, we can follow these steps:

Step 1: Understand the triangle configuration
We have triangle \( ABC \) with \( A \) at the top, \( B \) on the left, and \( C \) on the right. Since \( \angle BAC = 90^\circ \), \( AB \) is vertical and \( AC \) is horizontal. \( AD \) is the height from \( A \) to line \( BC \).

Step 2: Identify the segments
Since \( D \) is the foot of the perpendicular from \( A \) to \( BC \), we know:
- \( AD = 6 \, \text{cm} \) (height from \( A \) to \( BC \))
- \( BD = 4 \, \text{cm} \) (part of \( BC \))

Let \( DC \) be the other part of \( BC \). Thus, we can express \( BC \) as:
\( BC = BD + DC \)

Step 3: Use the Pythagorean theorem
To find \( AC \) and \( AB \), we can apply the Pythagorean theorem in triangle \( ABD \):
\( AB^2 = AD^2 + BD^2 \)
Substituting the known values:
\( AB^2 = 6^2 + 4^2 = 36 + 16 = 52 \)
Thus,
\( AB = \sqrt{52} = 2\sqrt{13} \, \text{cm} \)

Step 4: Calculate \( AC \) using the tangent function
Using the right triangle \( ABD \), we can find \( AC \) using the tangent function:
\( \tan(\angle ABD) = \frac{AD}{BD} = \frac{6}{4} = \frac{3}{2} \)
This means that for every 2 units of \( BD \), \( AD \) rises 3 units. Therefore, we can express \( AC \) in terms of \( AB \):
\( AC = \frac{3}{2} \times AB \)
Substituting \( AB = 2\sqrt{13} \):
\( AC = \frac{3}{2} \times 2\sqrt{13} = 3\sqrt{13} \, \text{cm} \)

Step 5: Find \( DC \)
Now, we can find \( DC \) using the Pythagorean theorem in triangle \( ACD \):
\( AC^2 = AD^2 + DC^2 \)
Substituting the values:
\( (3\sqrt{13})^2 = 6^2 + DC^2 \)
This simplifies to:
\( 117 = 36 + DC^2 \)
Thus,
\( DC^2 = 117 - 36 = 81 \implies DC = \sqrt{81} = 9 \, \text{cm} \)

Step 6: Calculate \( BC \)
Now we can find the total length of \( BC \):
\( BC = BD + DC = 4 + 9 = 13 \, \text{cm} \)

Final Answer
The length of \( BC \) is \( 13 \, \text{cm} \).

---